This blog 3 is related to my Self-Claimed Method, submitted to an International Scientific Community in Jan. 2022. Also, in this blog, I will explain design examples and basics, with how to select components and flyback transformer practical basics. This blog is again a tutorial blog without experimentation (experiments are in blog-4,5 and final blog), though the self-claimed method is based on experimentation and simulation.
In all my videos, I am not mentioning any vendor name, thus, controller name is not given.
All these videos, can be improved but reaching deadline of this contest. As these are elementary for school/diploma/graduate/phd, so they can make out. And these are also neccesary as scientific community would know, I have studied book myself before I present my novel research.
I. Videos Basics
1. Electrostatics
2. Magnetostatics
3. Cores
4. Air Gaps, What is Saturation
5. window utilization
6. Flyback transformer efficiency
II. Videos Flyback
1. Complete Design Example 1 , with component selection
Input : 18 V - 36V
Output 1 : 5 V, 4 A
Output 2 : 10 V, 20 mA
Topology : Current Mode Flyback
2. Complete Design Example 2 , with component selection
Input : 90 VAC - 264 VAC
Input line frequency : 50/60 Hz
Output : 19.95 3.25 A
Topology : Active-Clamp & ZVS Flyback
IV. Self-Claimed Novel ß Method
From the videos uploaded above, I hope engineers/phd/professors/post-phds can know, i have the knowledge of the subject.
Now, I explain my 'self-claimed novel method' which I had proved in Jan.2022 with 72 flyback transformers but unfortunately not much understood. I as such wont rewrite the paper as suggested by one reviewer but I may write other kind paper.
You can see the tables, all data different but accomplished graduate engineer & other phd reviewed with comment all data is same copied, all graphs & tables of part-2 and part-3 same . The conclusions do in part-2 & part-3 are similar but not any data or other comparative results ( earlier was suggested to separate them, now will have to unify.)
Paper - 1 explains the underlying methodology including simulation set-up and way of calculations are same in both series.
Paper-2 has results on 36 transformers
Paper 3 has results on another 36 transformers
A. Paper aim
Predict core saturation and instability in the control loop.
Proposed loss function can be justified to predict core saturation iff first order derivative,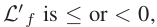
which indicates stability or asymptotically stablility.
The objective of simulation is to use non-linear parameters available from the above calculations which are used in practical flyback transformers and obtain the hysteresis BH curves and Lyapunov exponent.
GOAL
novel idea of representing flyback transformer losses as loss functions using hyperbolic secant spline interpolation, and its use in determining flyback transformer core saturation and instability in the control feedback loop from the loss function using Lyapunov method has been presented with scientific proof and validity.
This only was my goal and aim, which I had achieved.There can be future work on these papers and I had mentioned this in the paper.
However, this study still lacks generalized loss function, as proposed functions are related to a particular wattage and voltage.
My goal was not this, I had worked on some particular objective and achieved , whether such is possible or not, I used practical results on 72 transformers with 30 cores, and found it true (that is my proposition holding) in all the 72 transformers designed with 30 cores !
Moreover, 1-2 weakness I could write /review myself but these do not make this research garbage or like some accomplished degree engineer- not original paper or with no results etc.
The paper may not be important in itself due to non-generazilation but I have other things in mind for improved, and generalized result; and this paper helps me to think on it.
B. Method
For scientific validity, 72 working transformers at thirty-six wattage's which are being internationally used, have been examined to check, whether such proposition is valid or not.
Thirteen calculations for each transformer have been tabulated and used to derive mathematical loss function using hyperbolic secant spline interpolation which is subjected to Lyapunov method to predict core saturation and instability in the control loop.
In part-2 ,15 different cores - EPC13, EF12.6, EFD15, EE13, EE16, EEL16,EE19, EEL19,EFD20, EE25,E20/10/6, PR18x11,EEL22,RM6S/I, RM8/I, taken.
For part-3 , following 15 different cores - EEL22, EE25, EEL25,EF25, EE28, EER28, EER28L, EE35, RM8/I, ETD29/16/10,ETD34/17/11, E25/13/7, PR30x19, ATQ27/18, RM12/I, taken.
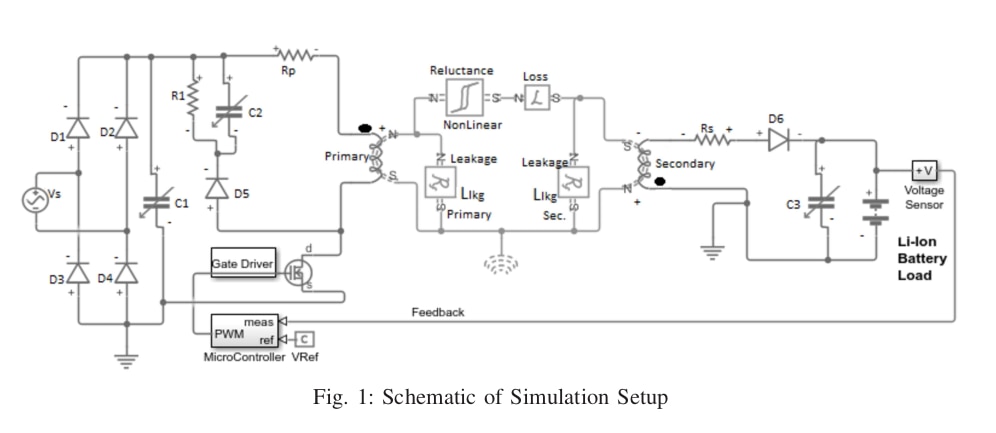
As the flyback transformers have inherent non-linear reluctance, lossess, primary/secondary leakages and primary/secondary windings, primary/seconday winding resistances, using these five components, simulation has been performed.
These 72 flyback transformers will be used in battery/mobile/laptop chargers, so the load is fixed non-linear lithium-ion battery with non-linearities.
Load Type : Li-Ion Battery (Mobile or Laptop)
C. Type of Results
- Definiteness of matrix,
- singular value decomposition,
- hysteresis curves
- Core Loss Lcr,combination of eddy & hysteresis losses.
- Copper Loss Lcp,include both DC & AC skin effect losses
- Leakage Loss
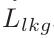which is commonly measured from the transformer primary with the pins of the secondary windings shorted. - maximum flux density Bm,
- peak flux density Bp,
- AC flux density,
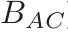
- reflected output voltage, Vor,
- continuous or discontinuous modemode, m , denoted as ‘C’ or ‘D’
- ratio
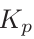
- gapped core specific inductance
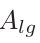
- MOSFET losses, LMOS,
- original losses
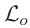
- proposed function which could mimic the original losses
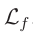
- errors from the plot of original loss function
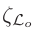
- errors from the plot of loss functions,
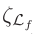
- original function
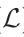
- definiteness of matrix for original function 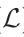is
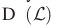
- definiteness of matrix for proposed function which could mimic the original losse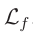s is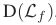
- Cholesky matrix decomposition, tabulated as yes (‘Y’) or No (‘N’).
- Lyapunov exponent
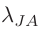which is calculated from BH curve of core loss - Lyapunov exponent
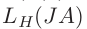,From simulation, that is, hysteresis losses LH (JA) in mW, after JA perturbation simulation. - inter-domain coupling factor α,
- Stability
All tabulated !
D. Simulation
Each of these 72 transformers are simulated with non-linearies of Li-ion battery namely state of charge (SoC), self-discharge resistance, temperature-dependent leakage resistance, five time-constant dynamics, deterioration of battery performance over repeateld charge and discharge cycles. The coefficients in the Jiles-Atherton model have been altered with fractional perturbations to obtain hysteresis curves.Various interesting comparisons in Bm,Bp,AC flux densities, effect on varying the coefficients in JA, residual errors, Lyapunov exponents, switching frequency vs MOSFET and transformer losses, reflected voltages, are tabulated and plotted.
1. Paper, Part- 1
1.1 Experimental Validation
The constant output voltage is one of the requirements in flyback transformers used in mobile or laptop
battery chargers, so it is regulated by feedback loop .
Thirty Six wattages from 2W to 85 W which are internationally used in laptop and mobile chargers have been selected. The voltage and current ratings used in this study are not from my choice but has been selected as per market specifications.
For each of these thirty-six flyback transformers, two transformers with the best optimization and lowest possible losses in different modes – continuous or discontinuos on different cores, have been calculated manually, which were later sold to manufacturers to be originally used in the product, viz., mobile or laptop charger.
These flyback transformers are operated in continuous or discontinuous mode, switched from MOSFET which is driven from PWM signals by microcontroller. The switching frequency is fixed for a particular transformer but all these 72 transformers have different switching frequencies from 41 kHz to 97 kHz, and have different core materials. The selection of which transformer rating is operated in continuous or discontinuous mode or designed with which core, is in general random but has been decied by these factors - best optimization, lowest possible losses, capability of the core.
To add more practicality, for the same wattage, different voltage and current ratings have been used, for instance, 20W can be made with 15V or 9 V or 11V or 12V. The transformers which have been derived by such combination are prefixed with ’p’ in ‘Watt’ column,e.g. p0, p1, p2, p3 means one design with one voltage and current rating, second design with other voltage and current, third design with other voltage and current, and fourth design with other voltage and current rating.
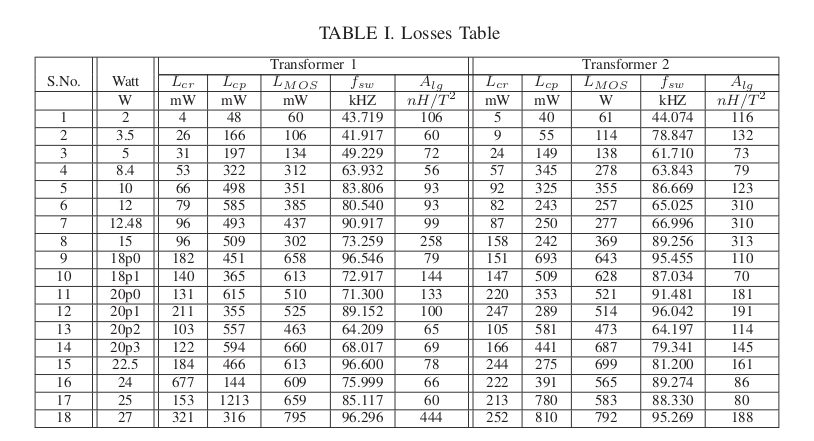
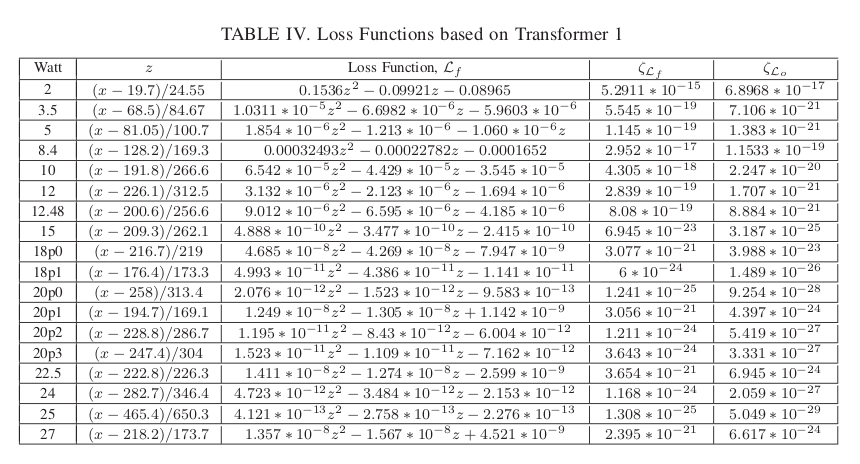
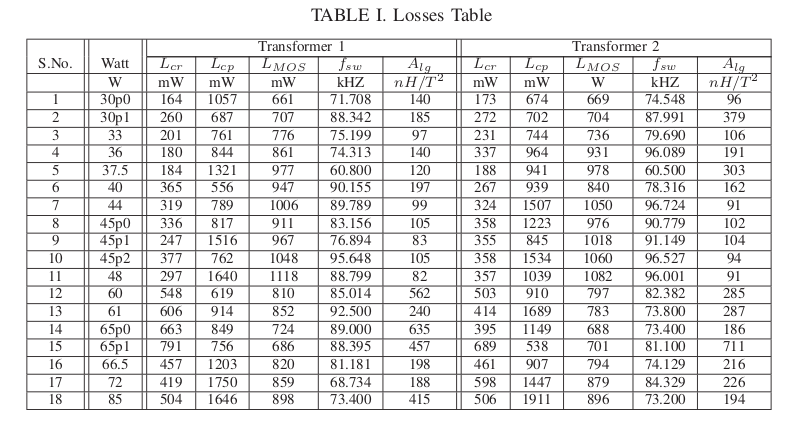
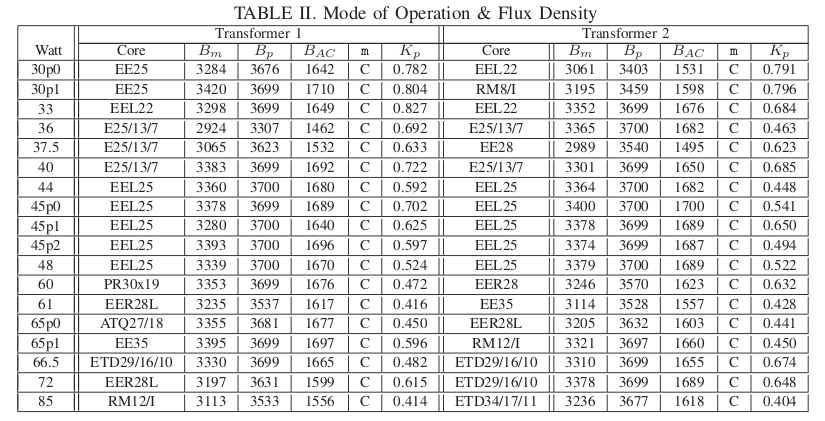
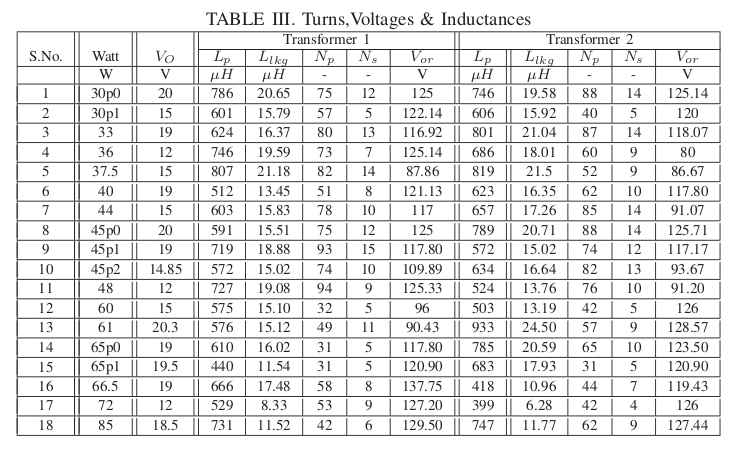
For each of thes 72 transformers, thirteen calculcations - core losses, copper loss, leakage losses in the flyback transformer, combined MOSFET losses, gapped core specific inductance,Alg , primary inductance, current waveform, flux densities - maximum flux density, peak flux density, AC flux density, reflected output voltage, number of primary turns, number of secondary turns, are done manually using formulae given in references which are tabulated in Tables I, II, and III.
As this study is specifically on the flyback transformer losses and magnetics, the collective MOSFET losses,LMOS , include conduction losses,(RDS(ON ) loss,current sense resistor losses), and switching losses
( CV2F, , crossover loss), are given in the Table I.
These MOSFET losses,LMOS thus, include losses due to finite switching time of MOSFET and due to dissipation in the stored energy of the parasitic capacitance of the transformer and the output capacitance of MOSFET.
Flyback transformer has three kinds of losses- (i) Core Loss, Lcr , which is combination of eddy & hysteresis losses. (ii) Copper Loss Lcp , include both DC & AC skin effect losses and (iii) Leakage Losses in the transformer, Llkg , which is commonly measured from the transformer primary with the pins of the secondary windings shorted. But, in this paper, the mentioned values in the table are calculated and estimated with primary inductance tolerance of 5 % .
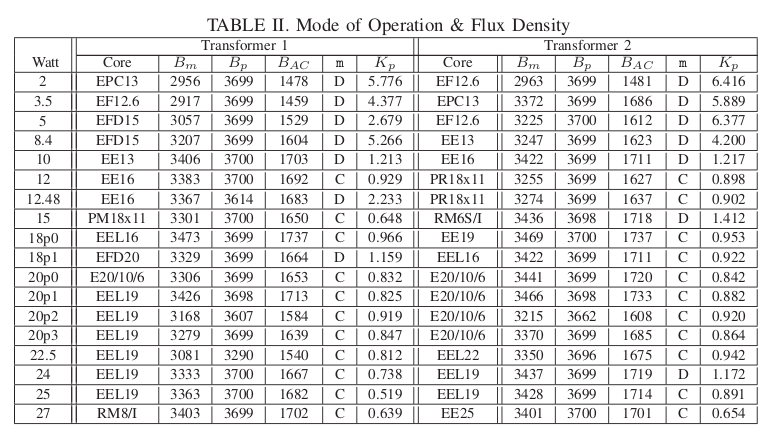
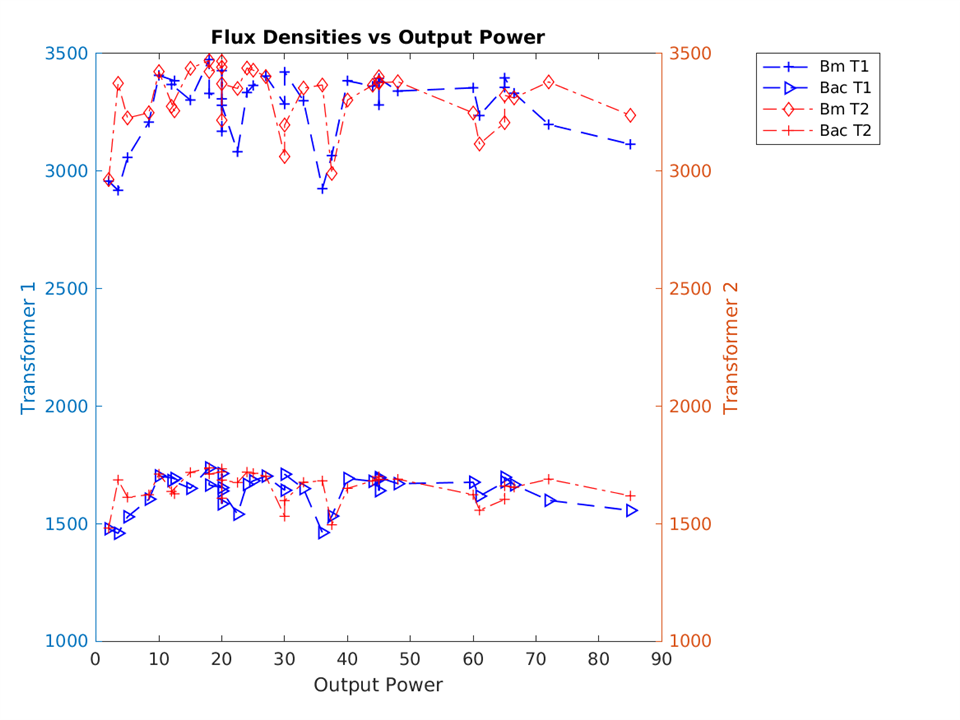
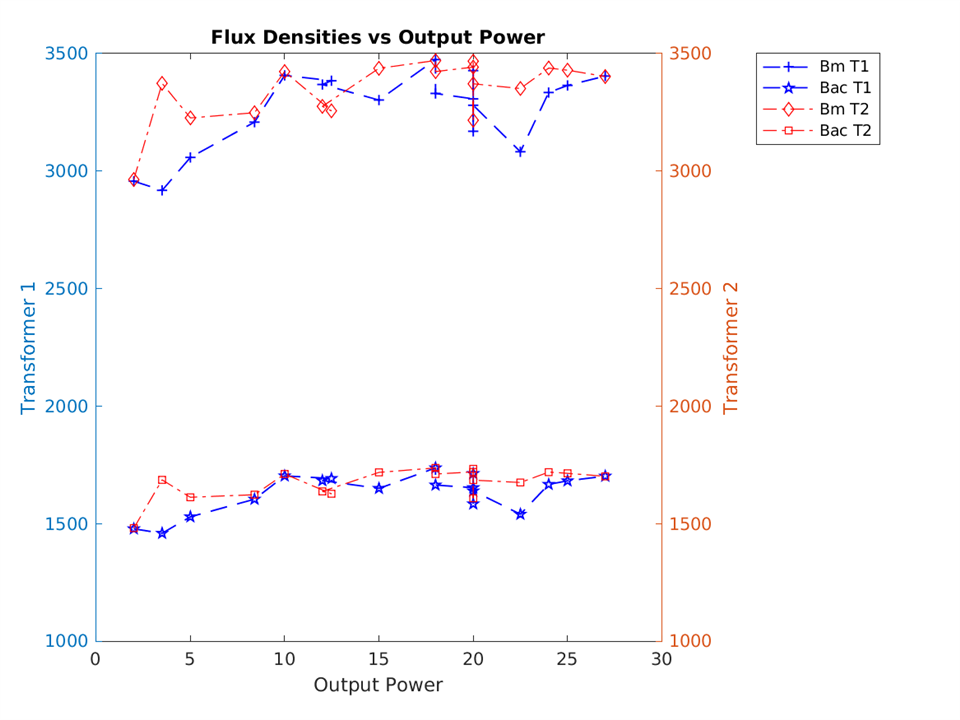
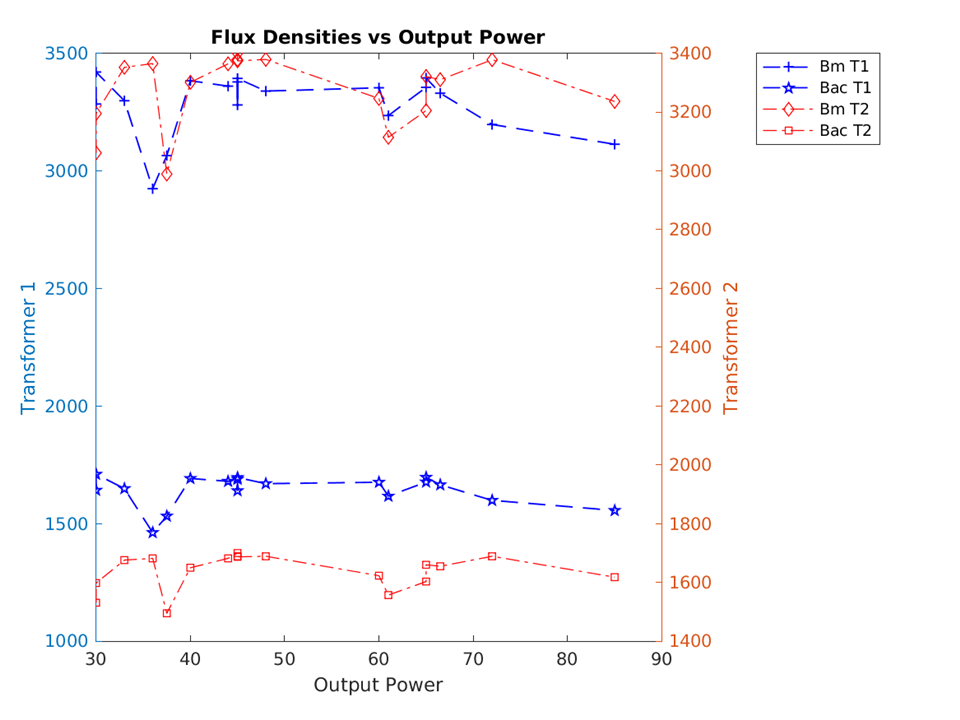
The flux densities is also divided into three - maximum flux density (Bm ), peak flux density (Bp ), and AC flux density (BAC ). These parameters if gets exceeded during the operation causes saturation in the flyback transformer core, which are known to happen at the time of transformer energization. From Table II, BAC < Bm < Bp , BAC is the lowest as it is the flux density amplitude at full power, and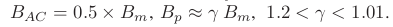 
This constitutes the experimental validation as earlier mentioned, based on these 12 calculations, the transformers were designed by the manufacturer and sold in the market.
All the tables are tabulated for both designed transformers T1 & T2. Thus,
Table I lists all the three losses, Lcr , Lcp , LMOS at the transformer switching frequency fsw along with gapped core specific inductance,Alg . Alg defines its’ non-linear relation with inductance, and is the inductance coefficient.
Table II lists the cores used along with three flux densities (Bm , Bp , BAC ) and continuous/discontinuous mode, m with ratio, Kp .
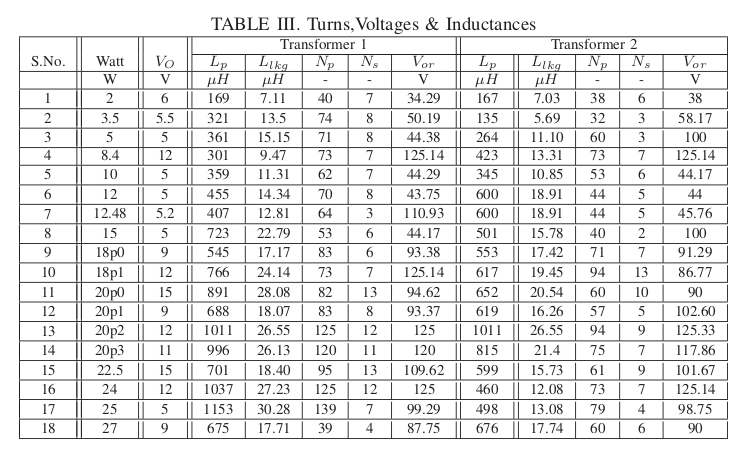
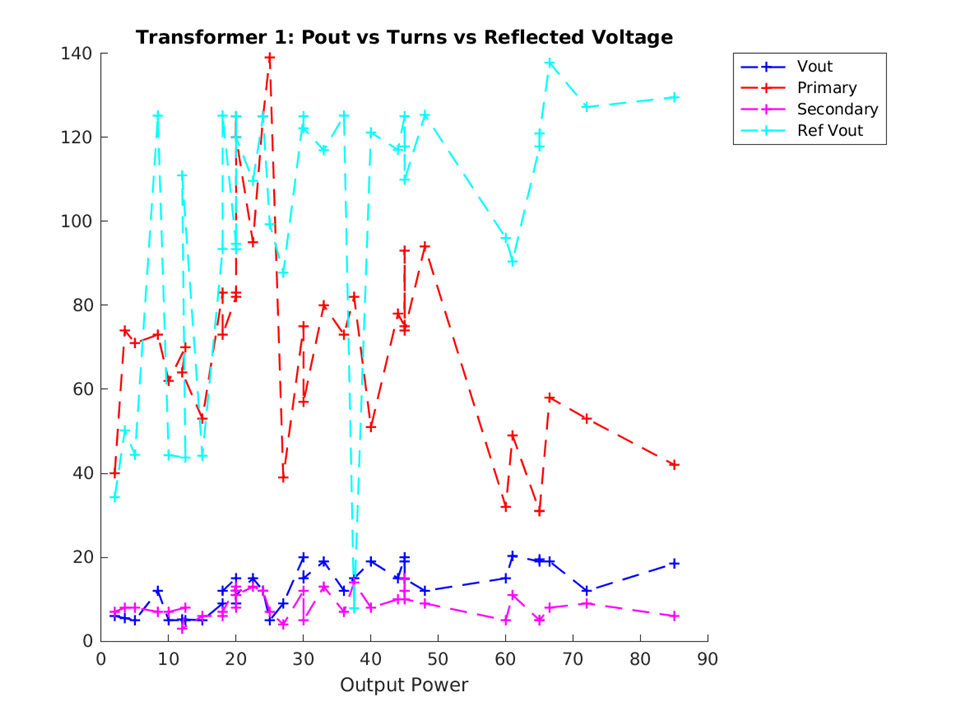
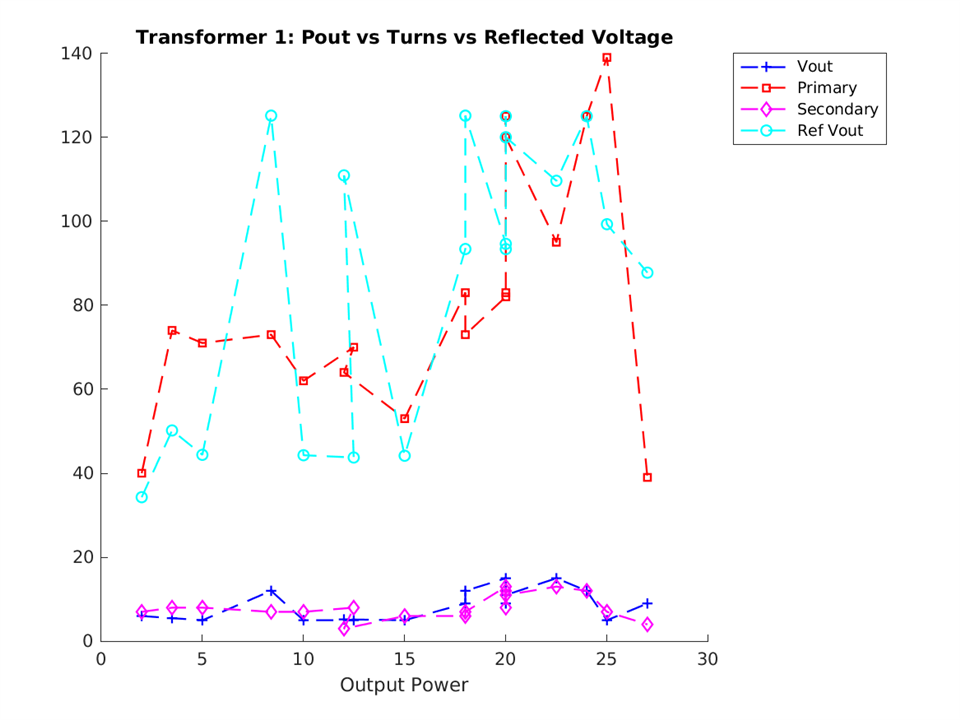
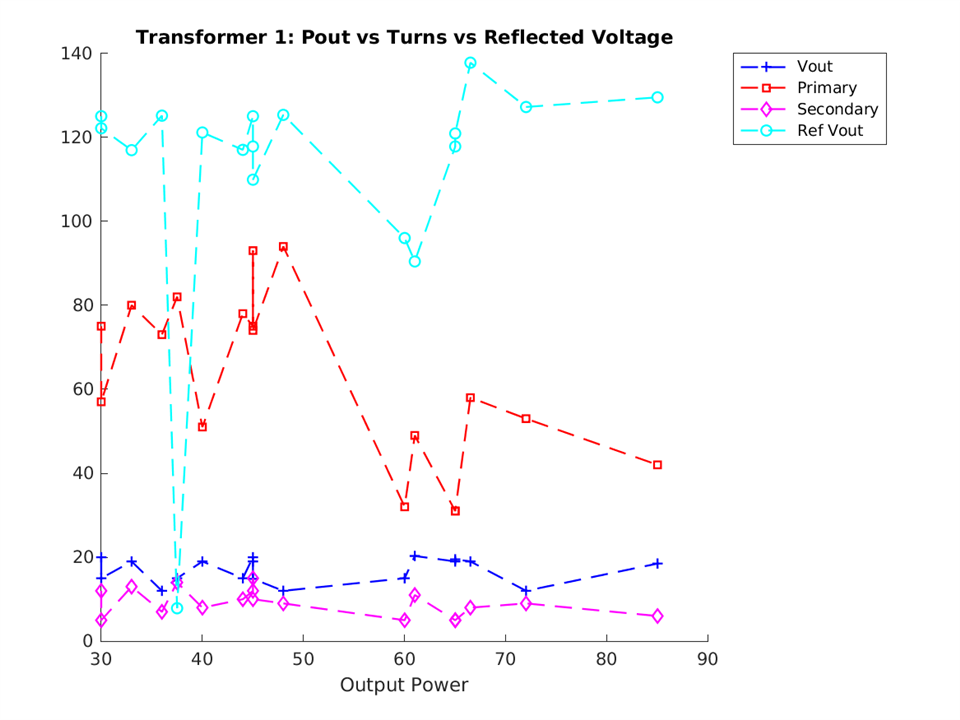
Table III lists the output voltages, VO from the market specifications on which the wattage, W is needed, along with transformer primary inductance (Lp ), leakage loss in the transformer Llkg , number of primary (Np ) and secondary (Ns ) winding turns, reflected voltage, Vor .
1.2 Loss Function Proposition
Though, some physicists may argue, transformers cannot store energy, it is well known that the primary of the flyback transformer stores energy (ξsp ), which is transferred to the secondary (ξts ) through flyback action. This stored energy provides energy to the load,and charges the output capacitor.
Here, I have introduced the loss function and energy function proposition with justification. The purpose of these loss functions as will be seen achieves three goals (i) Transformer losses can be represented using them (ii) Transformer core saturation can be determined from the losses itself via loss functions (iii) Due to the transformer losses/saturation, the instability in the control feedback design can be predicted.
The tabulated three kinds of losses Lcr , Lcp , Llkg , in Tables I and III, are the losses associated with the transformer. Thus, the energy function is
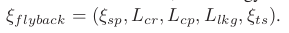
This simply means the energy stored in the flyback transformer is the function of stored energy in the primary ξsp , the above mentioned three losses and the energy transferred to the secondary ξts . The subscripts ‘sp’ mean Stored in the Primary, ‘ts’ means Transferred to the Secondary.
It is obvious for higher efficiency of the flyback transformer, the losses have to be minimized.
Let L be the loss function and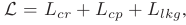 
which has to be minimized & the significance of Llkg is widely implemented in multi-resonant topologies.
Thus,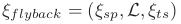 
and
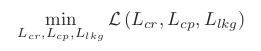
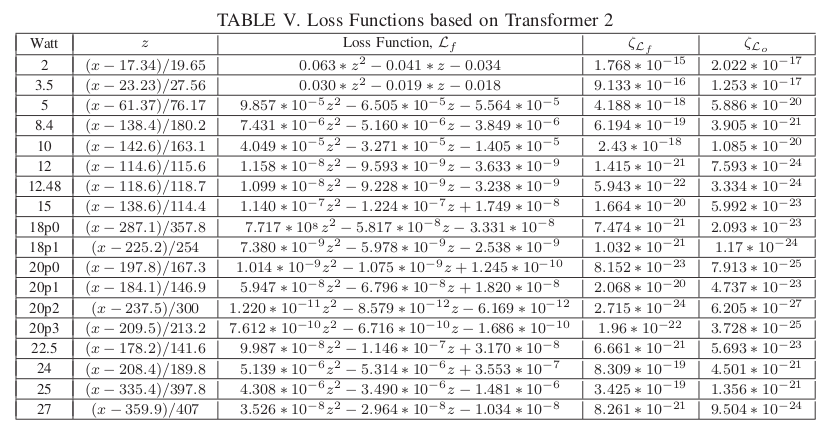
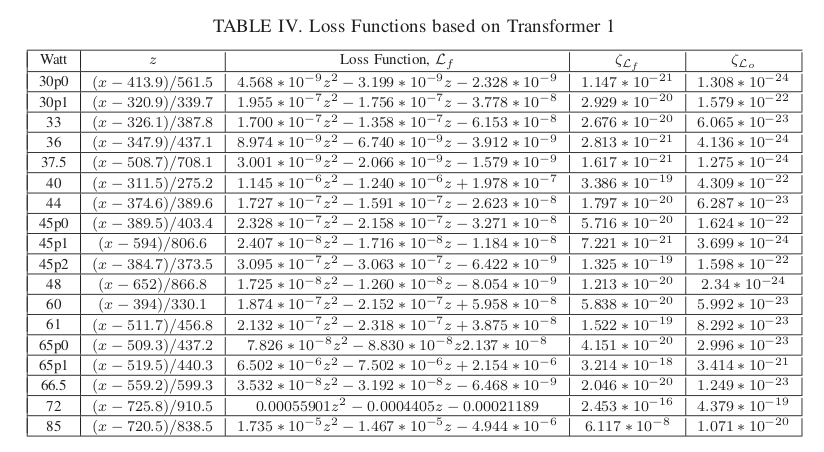
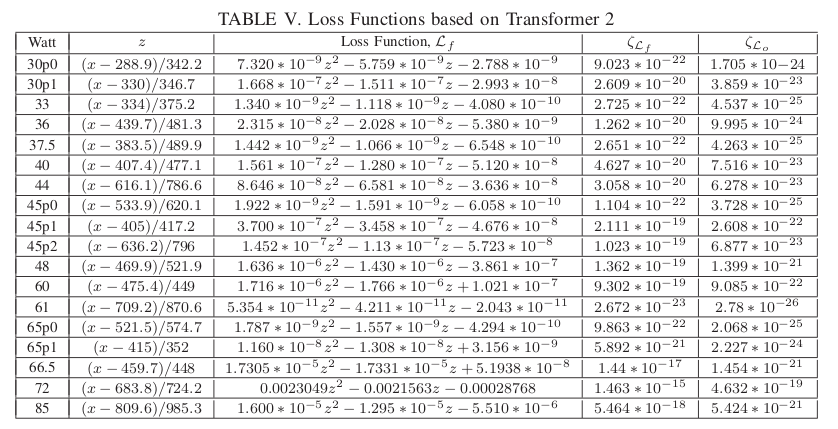
Let the original losses given in Table I be Lo , and now the objective is to have some mathematical function, which could decipher these losses. Let the proposed function which could mimic the original losses be Lf . As these would be numerical approximations, the errors in comparison to the original losses are denoted as ζLf and ζLo . This has been done for both transformers of each of the 36 wattage’s and are tabulated in Tables IV & V. These residual or errors represent how closely, the function is able to
represent the original loss.
1.3 Loss Function Derivation
Table IV & Table V lists the loss functions after spline interpolation with the errors from the plot of original loss function, ζLo and from the plot of loss functions, ζLf .
The loss functions obtained here are not unique, the author has tried other functions but the given function method appeared to be closely approximating and representing the actual losses.
From Table I, for transformer 1, Lcr = 4mW, Lcp = 48mW and from Table III, Llkg = 7.11μH. It can be noted that the units of Llkg are different, but here losses are not being added, rather the objective is the formation of some function, which could achieve above three goals. As the original losses are three in number, which have to be minimized, Lo is 1x3 non-square matrix = [4 48 7.11] of rank 1, on which hyperbolic secant spline interpolation is performed, i.e. (y = sech(Lo )). The query points are selected
based on the wattages and convinient random scale. The resulting curve is interpolated, fitted and the polynomial in degree 2 is obtained, so as not to achieve merely some complex mathematical formulation but to be used as Lyapunov function candidate and/or in quadratic performance index. As this is the derived function based on the orignal curve, it is called proposed loss function,Lf . Thus, corresponding to this particular matrix,Lf = 0.1536z 2 − 0.09921z − 0.08965. Here, z is centered and scaled around the
x-axis; for this particular matrix Lf , z = (x − 19.7)/24.55.
Theoretically, these loss functions are not 100% perfect as deviation from the prediction errors can be calculated using known method of root mean square error. The corresponding standard deviations are tabulated as residuals or errors as ζLf and ζLo .
1.4 Loss Functions & Lyapunov
The first objective is achieved in the subsection ‘B’ whether transformer losses can be represented by any mathematical function. Now, whether the same loss function could predict or determine core saturation (the other main concern in flyback transformer), this subsection explains this.
It is known since 1892 (as shown by Lyapunov) that certain other functions could be used instead of energy to determine stability of an equilibrium point, but no method for finding them is known till date. The proposed loss functions could be used as Lyapunov function candidates or in the quadratic
optimal control function.
As the proposed loss functions are based on practical calculations, which indicate if those limit values are passed, transformer can saturate and will cause instability in the feedback loop.
It is known from Lyapunov method if the first order derivative ≤ or < 0, it indicates stability or asymptotically stablility respectively.
The core saturation means instability, as it causes transformer instability & instability in the feedback
loop, thus, the validity of the proposed loss function can be justified to predict core saturation iff first
order derivative, 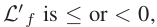, which indicates stability or asymptotically stablility.
All the 72 derived/proposed loss functions for transformer 1 (in Table IV) and for the transformer 2 for the same wattage (in Table V), are calculated for Ist order derivatives.
My IEEE CodeOcean capsule has two folders ‘t1_lf_derivative’ & ‘t2_lf_derivative’, where the first order differentiation of the loss functions has been shown and the respective loss functions are in folders -‘t1_lossfunctions’ and ‘t2_lossfunctions’.
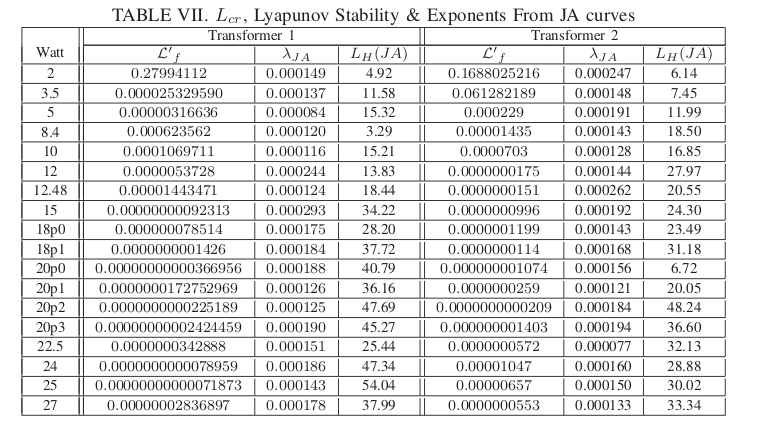
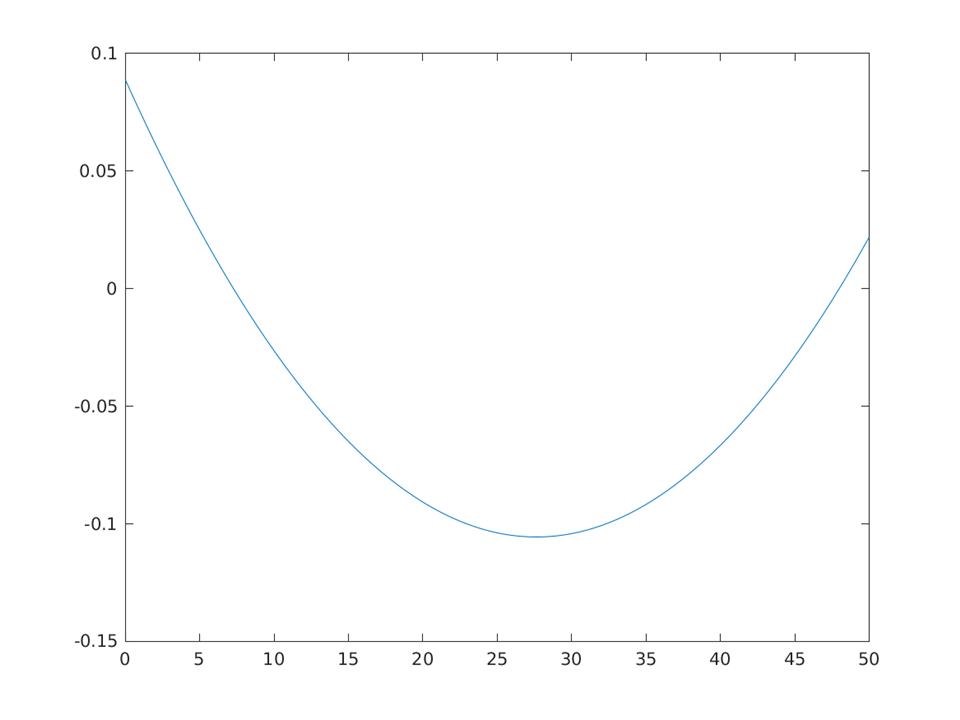
The value of Ist order differentiation obtained for all these 72 transformers is tabulated in Table VII, which shows all L′ f ≈ 0,and L′ f (60) < 0 = −0.000000588751. Thus, the proposition of loss function is valid, where the stability has been co-related with transformer saturation and feedback loop instability because all these are derived from practical calculated values of the working transformers.
1.5 Loss Functions & Matrices
As shown in subsection ‘C’, the losses can be written in matrix form. Similary, the coefficients of Lo and Lf can be written in matrix form, e.g. [0.1536 -0.09921 -0.08965].
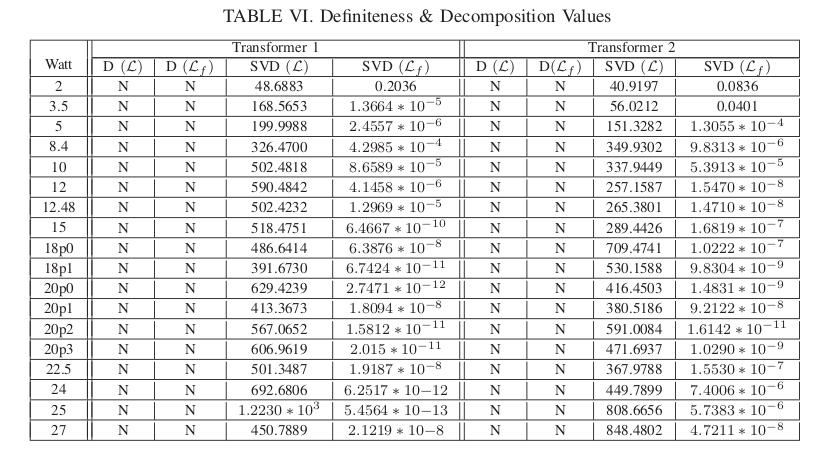
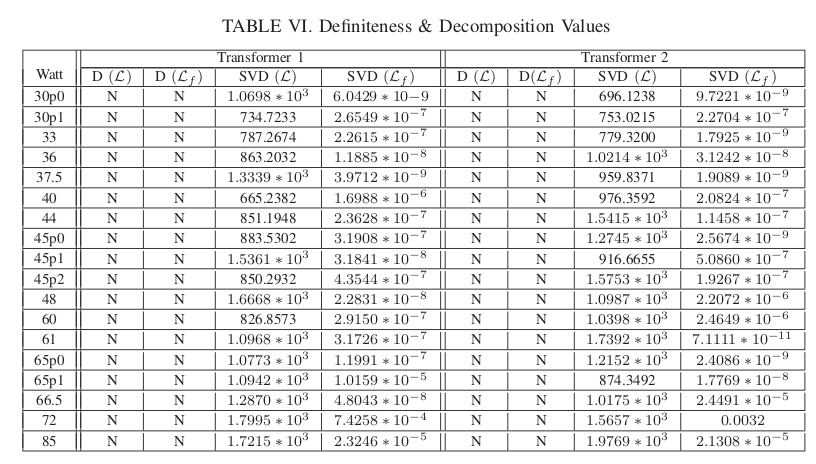
It is also known that (besides using L′ f ≤ 0, for predicting stability), the stability can also be predicted from the definiteness of matrices, that is, if the matrix is symmetric or symmetric positive definite, it is stable.
The Cholesky matrix decomposition is the known method to tell positive definiteness of matrix using Cholesky factorization.
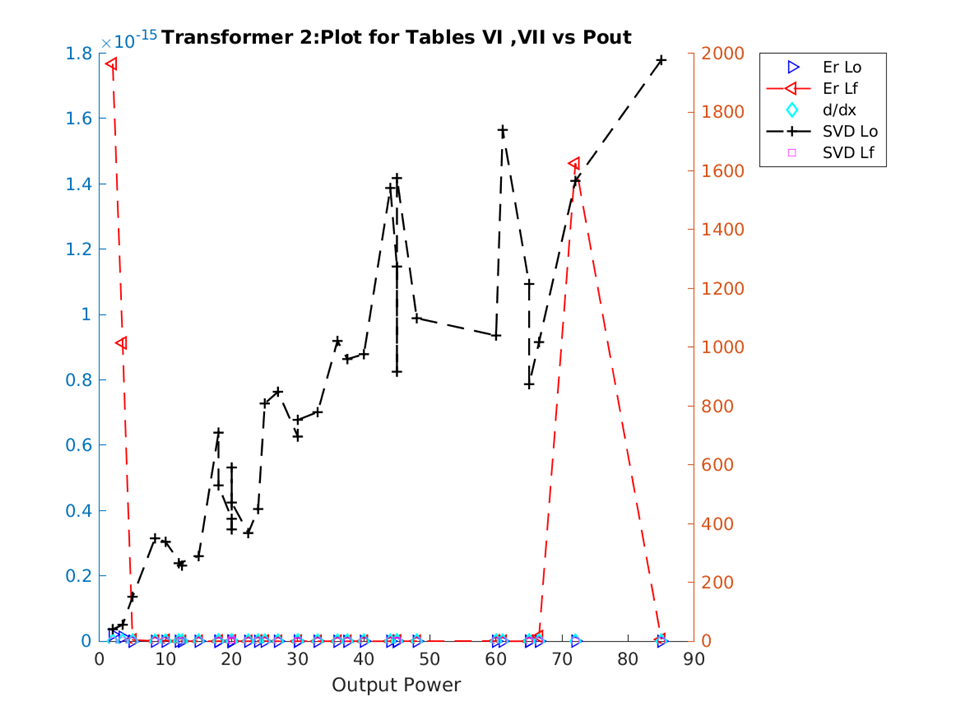
Table VI, lists the results after calculations from matrices of the loss function (Lf ) and original function (L). The definiteness of matrices whether the matrix is symmetric or symmetric positive definite, is given in column D (L) & D(Lf ). These are calculated using Cholesky matrix decomposition, and has been tabulated as yes (‘Y’) or No (‘N’).
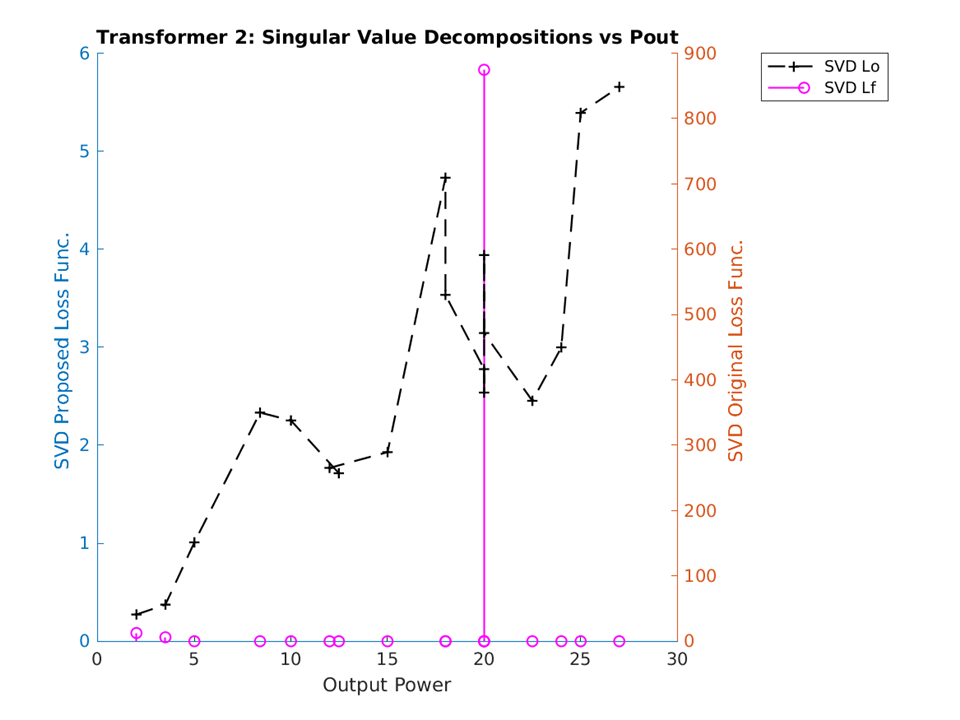
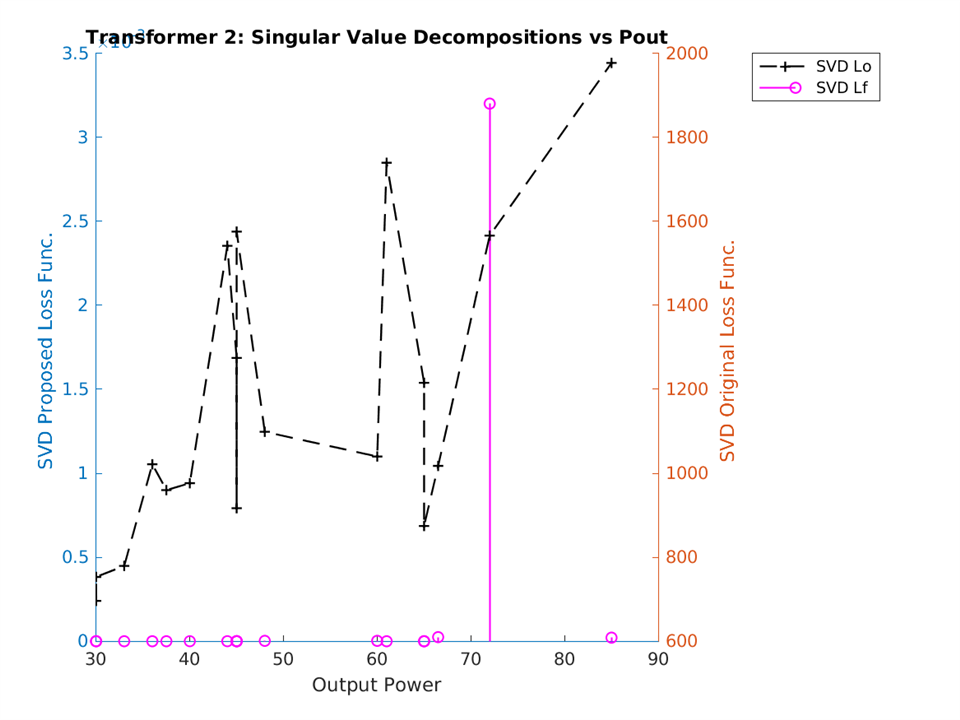
The results of SVD has not been used but obtained to be used for future research.
The singular value decomposition, SVD (L) of the original loss function,and, SVD (Lf ) of the proposed loss function, is also tabulated in Table VI.
1.6 Other experiment Considerations and practical suggestions
All the following transformers are flyback switching two-winding transformers, designed and implemented in practical industrial use, with estimated efficiency of 87%, with safety standard of EN 61000, Class 3, in adapter enclosure, to be operated in constant voltage mode with output voltage tolerance of ± 2%. Following other known considerations also has been taken into account.
All of the transformer core’s has an air gap in its limb to reduce the chances of magnetic saturation, which increases the magnetic field intensity, H, without changing any saturation flux density of the core material,thereby, increasing the working range of BH & the throughput of the flyback transformer.
In all of these transformers,the margin is half the required primary to secondary creepage distance ≈ 2.5 mm, and the margin winding is applied with layers of slit tape, wrapped in sufficient layers. The primary layers have been separated by at least one layer of polyester film tape,3M 1298,which reduces chances of interlayer voltage breakdown. The layer to layer insulation has been improved with epoxy. The maximum operating flux density has been chosen taking into account the peak flux density, core saturation, start-up, short-circuit, current limit and variable inductance values. In flyback transformer designing, the reflected output voltage, Vor is an important parameter as during diode conduction, it is reflected back to the primary, through transformer turns ratio, and is the secondary winding voltage.
These flyback transformers could be designed to operate in continuous (C) or discontinuous (D) or borderline mode, which is decided by parameter current waveform Kp , which simply means whether the magnetic field in the core over one complete switching cycle is zero or not. The mode of operation used in 72 transformers is given in Table II, listed in the 6th & 12th column under m as ‘C’ or ‘D’, which corresponds to continuous or discontinuous mode respectively.
For continuous mode, Kp ratio, allows the margin for wider leading edge current spike, which is caused by the discharge of the drain node capacitance of MOSFET. For discontinuous mode, Kp is the ratio of MOSFET off time to the conduction time of the diode connected with transformer secondary.
1.7 simulation set up parameters of the battery load (along with formulae used in non-linear battery),
The load is taken as li-ion battery,where the load battey model has been implemented with state of charge (SoC), self-discharge resistance, temperature-dependent leakage resistance, five time-constant, τ dynamics, deterioration of battery performance over repeated charge and discharge cycles.
As this is comparative study on 72 transformers with different voltage ratings, the exact voltage is variable but the charging voltage of each cell is 4.2 V(tolerance of around ± 50 mV per cell). Let the voltage Vb across battery be Vb = vt (S, T ), where S is the state of charge.
In this study,the load battery is modeled with five parallel RC sections with 5τ time constants,τ1 , τ2 , τ3 , τ4 , τ5 , = (S,Tb ) where S is vector [0, .25, .75, 1], and Tb is temperature in kelvin with vectors [273.15, 298.15,323.15].
The self-discharge leakage resistance, Rblkg (Tb ) has vector [8000, 7000, 6000].
As practical batteries deteriorate over repeated charge and discharge cycles, the vector of discharge cycle is [100, 200, 300].
1.8 Jiles-Atherton model
The practical implemented transformer values - Ns ,Np ,Llkg ,Lp ,Ls , losses,core’s effective cross-sectional area & mean length path with air gap length, had been put in each of these 72 simulations.
Let, G, is the variable gain, used to trace curve when the magnetisation, saturation, Bm is in opposite
direction,
and G= Kδ − α(Man − Mirr ).
The three coefficients in the equation are α, c, K.
(i) inter-domain coupling factor, α (0.0001 < α < 0.001);
(ii) coefficient for reversible magnetization, c, 0 < c < 1
(iii) bulk coupling coefficient, K.
In the initial start, parameters like field strength and flux are all zero. The coefficients c, K, α matrix [0.1 165 0.0001] are fractionally perturbed as ∆p = [6 1.5 1.5], after 300 input Vs cycles i.e.,after 300 times complete revolution of 360◦ .
1.9 Lyapunov exponent
It is obvious that when three coefficients will be adjusted to mimic load perturbation, BH curves will be obtained and during the complete perturbation of the load, numerous trajectories of hysteresis curves will be obtained. It is known that Lyapunov exponent characterize the rate of separation of infinitesimally close trajectories.
Two Lyapunov exponents have been derived for future research use
(i)λJA ,the Lyapunov exponent, which is calculated from BH curve of core loss, which by definition is eddy and hysteresis (listed inTable I, in mW)
(ii) From simulation, that is, hysteresis losses LH (JA) in mW, after JA perturbation simulation.
Let the BH curve obtained after 300 input cycles with 3 perturbations has N points (h1 , b1 ), ..., (hN , bN ).
For the point (hi , bi ), i = 1, ..., N on BH axis
Then, a single value of λJA is obtained from polynomial curve fitting using Vandermonde matrix.
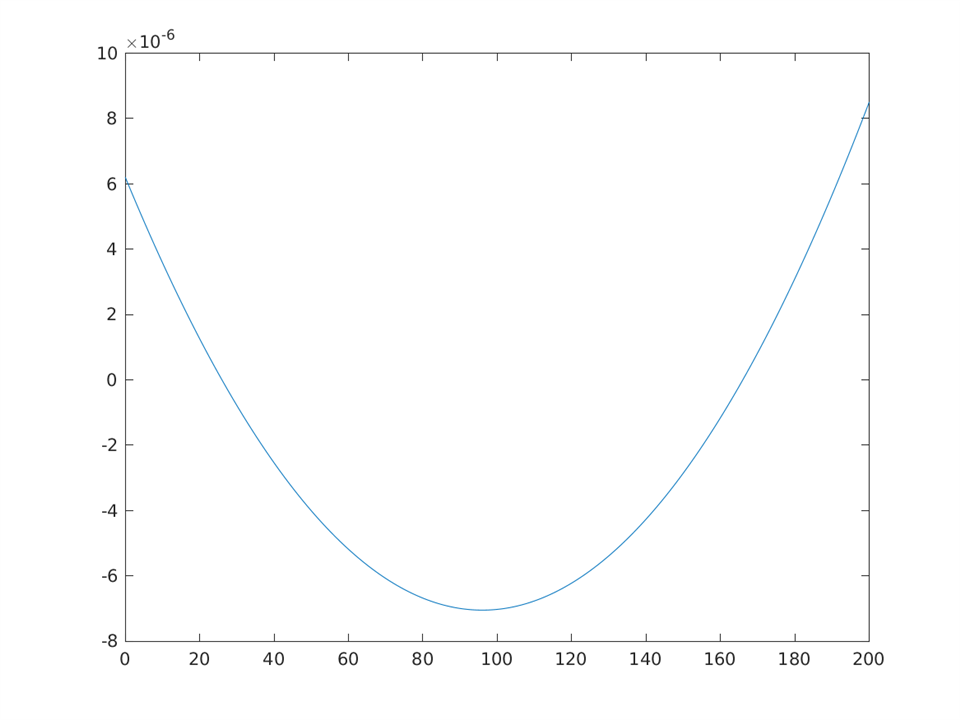
Table VII, lists the value at the first order derivative, L′ f and the λJA and LH (JA) in mW.
1.10 Conclusion
I had successfully performed and tabulated manual calculations of the flyback transformer designing.
I have successfully related my idea of representing the transfomer losses with the loss function and its use in predicting core saturation and control feedback loop instability.
Some of the derived results has not been used in this work but kept for future research use.
| {gallery}Paper, Part- 1 |
|---|
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
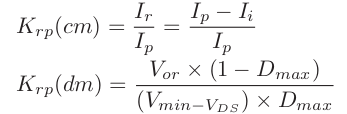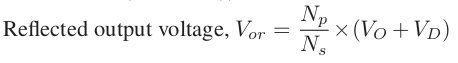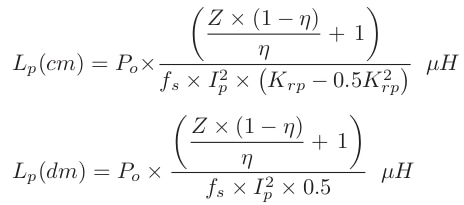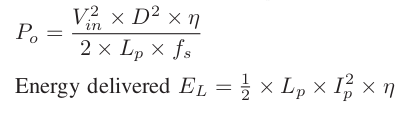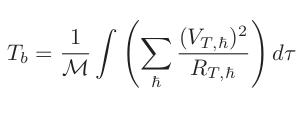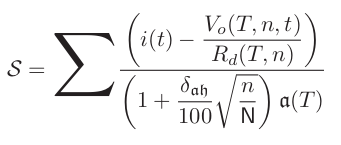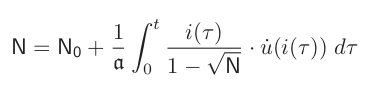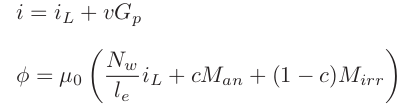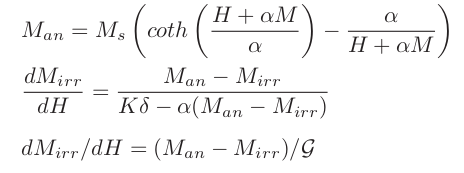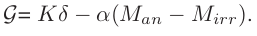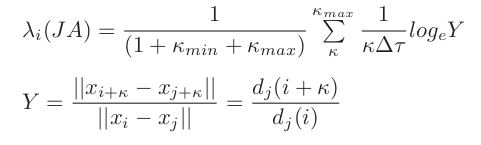2. Paper, Part- 2
In this paper, the comparative analysis of 36 different flyback transformers from 2W to 27W is being presented. These two-winding transformers are operated in continuous/discontinuous mode, switched from MOSFET controlled by microcontroller from 41 kHz to 97 kHz. Eighteen wattage’s which are used internationally at different voltage and current ratings have been analyzed on fifteen different cores - EPC13, EF12.6, EFD15, EE13, EE16, EEL16,EE19, EEL19,EFD20, EE25,E20/10/6, PR18x11,EEL22,RM6S/I, RM8/I.
2.1 Results
1. The first observation is that, even if the voltage or current rating in increased in decimal points, the same transformer core cannot be used as it increases transformer leakages, and the number of primary layers gets increased, which has to be done oppositely for higher efficiency. The number of primary layers, should be such that it meets the primary current density limit.
2. If the maximum operating flux density,BM is not restricted to the levels, it generates more audible noise.
The size of the magnetic core is a function of the switching frequency and the output power.
3. The more, the output voltage is reflected, Vor , the more the leakage inductance, and, the peak & RMS currents of the secondary side,duty cycle of MOSFET,transformer secondary side copper losses are increased, which, reduces efficiency of the power supply.
4. Improper packaging/construction increases common mode emissions.
5. The AC copper losses resulting from skin effect can be reduced with the use of smaller AWG wire by using multiple strands wound in parallel. Increasing Lp reduces Ip(pk) , which reduces primary clamp losses.
6. operating mode
Transformers ranging from 2W to 10 W have been operated in discontinuous mode, whereas from 12W to 27W in continuous or discontinous mode or in both modes for comparative studies.
The transformers operated in discontinuous mode are smaller in size but have higher losses and lower efficiency due to higher RMS currents, as compared to those operated in continuous mode.
12W & 12.48W has been designed with same core EE16 at Vo = 5 & 5.2 , in both C & D modes,
and other core PR18x11 in only m = C.
For EE16,
- Lp (12.48) D ≈ 0.89Lp(12) C ,
- Llkg (12.48) D ≈ 0.89Llkg (12) C ,
- Bm (12.48) ≈ Bm (12),
- Vor (12.48) >> Vor (12).
For PR18x11,
- Lp (12.48) = Lp (12),
Llkg (12.48) = Llkg (12), - Vor (12.48) ≈ 1.04 Vor (12).
Thus, for ∆ = 0.2V, the comparisons can be seen.
15W has been designed in both C & D with cores PM18x11 & RM6S/I, with exactly same specifications.
- The flux densities, Bm (C) ≈ 0.96 Bm(D) but Bp (C) ≈ Bm (D).
- The inductances, Lp (C) ≈ 1.44 Lp(D) ;
- Llkg (C) ≈ 1.44 Llkg (D).
- The number of turns Np (C) ≈ 1.3 Np (D),
- Ns (C) ≈ 3 Ns (D).
- The SV D(L)) C ≈ 1.8 SV D(L)) D .
18W has been designed in both C & D with cores EEL16 and EFD20 but with Vo = 9 &12 respectively.
- The flux densities, Bm (C) ≈ 1.04 Bm (D)
- Bp (C) = Bm (D).
- The inductances, Lp (C ≈ 0.7 Lp (D) ;
- Llkg (C) ≈ 0.7 Llkg (D).
- The SVD (L) C ≈ 1.24 SVD (L) D .
Eight 20W transformers are designed with Vo = 9V,11V,12V,15V, all with m = C.
Five transformers use same core E20/10/6 but Bm 6= Bp 6= BAC , even for slight output voltage difference ∆ = 2V at the same output power.
Also, Lp ,Llkg are not equal.
Similarly, for three transformes with core EEL19, Bm 6= Bp 6= BAC .
For T1 :
- Lp (9) < Lp (15) < Lp (11) < Lp (12) ;
- Llkg (9) < Llkg (11) < Llkg (12) < Llkg (15);
- [λJA (9) ≈ λJA (12)] < [λJA (15) ≈ λJA (11)] ;
- LH (JA)(9) < LH (JA)(15) <LH (JA)(11) < LH (JA)(12);
- SV D(L)(9) < SV D(L)(12) < SV D(L)(11) < SV D(L)(15).
For T2 :
- Lp (9) < Lp (15) < Lp (11) < Lp (12) ;
- Llkg (9) < Llkg (15) < Llkg (11) < Llkg (12)
- λJA (9) < λJA (15) < λJA (12) < λJA (11) ;
- LH (JA)(15) << LH (JA)(9) < LH (JA)(11) <LH (JA)(12)
- SV D(L)(9) < SV D(L)(15) < SV D(L)(11) < SV D(L)(12).
24W has been designed in both C & D with core EEL19, with exactly same specifications.
- The flux densities, Bm (C) ≈ 0.96 Bm(D) but Bp (C) ≈ Bm (D).
- The inductances, Lp (C) ≈ 2.25 Lp(D)
Llkg (C) ≈ 2.25 Llkg (D). - The number of turns Np (C) ≈ 1.7 Np (D),
- Ns (C) ≈ 1.7 Ns (D).
- The SV D(L)(C) ≈ 1.54 SV D(L)(D).
Besides above comparisons, the peak currents Ip in continuous mode flyback transformers are lower than discontinuous mode.
For m = C,the ratio Krp < 1, which makes primary inductance higher than discontinuous mode as Krp > 1.
The upper limt of Lp in continuous mode is approximately four times that discontinuous mode,that is,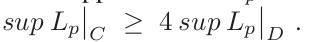 
The control feedback loop of flyback transformers tends to destablize in continuous mode as compared to discontinuous mode, because MOSFET’s duty cycle, shifts the right half plane zero and complex pole pair,so it needs expertise to design stabilization loop.
The insufficient gain or phase margins or bandwidth causes the transformer & PCB to oscillate, and, output voltage to overshoot with large ripple.
7. JA & loss function
The loss functions are different for the same wattage even at slightly different voltage level, e.g. in 12W & 12.48 W (5V & 5.2V). And the residual errors are in femto (10−15) or zepto (10−21 ) scale.
The coefficients of the loss functions are also in micro-scale range because these are associated with losses,which are in mW or μH.
It can also be noticed that the anhysteretic magnetization of JA is defined using hyperbolic cotagent,which is product of hyperbolic sine and hyperbolic secant.
And the proposed loss functions, Lf , are more appropriately defined by hyperbolic secant of the original loss function L, rather than any other function,
Here, in these experiments, the coefficients are not manually adjusted to set desired gradients or intercepts but adjusting coefficients,perturbations also affects hysteresis & other losses.
8. Definiteness & Decomposition Values
The loss functions form matrices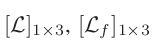 
of rank 1 & thus SVD computes this matrix approximation, returning a single value rather than decomposition into three matrices.
The definiteness is used to predict stability.
From Table VI, neither any Lf nor L is symmetric or symmetric positive definite.
Thus,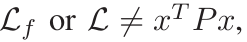 
as ‘P’ is real symmetric matrix.
But this do not imply that,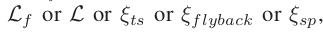 
, will deviate from stability, i.e., causing saturation, on connecting load (5 τ battery), as here the flyback transformer is non-linear, which inherent has more than one stability point along the hysteresis curve, & the hysteresis converges along the microzigzag curve.
The validity of the proposed loss functions has been stated earlier.
The first order derivative shows :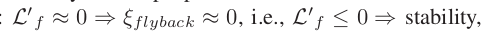 
that is, the energy loss function is decreasing (Table VII), and hence stability, no saturation.
Thus, all loss functions are valid, where the stability has been co-related with transformer losses,saturation, feedback loop instability, Lyapunov stability/exponent, and this study proves, it is possible and the proposition is valid.
9. lyapunov exponent
In the Sec. II(B), [c, K, α] = [0.1 165 0.0001] is fractionally perturbed with ∆p = [6 1.5 1.5], after 300 input Vs cycles.
Thus, three trajectories are obtained, which are infinitesimally close. And Lyapunov exponent λJA is used to characterize the rate of separation of these three infinitesimally close trajectories in phase space to distinguish different attractors or varying coefficients. It is known, +λJA ⇒ divergence and chaos,and −λJA ⇒ convergence.
From, Table VII, all λJA ≈ 0, in the micro-scale, which shows, they are stable and again shows the validity of the underlying principle, but if the flux densities exceeded, the λJA > 0, and will cause instability in the control loop along with core saturation.
10. Plots are given under 'codeocean'
Fig. 2 : Explains the legends used in Fig. 3 - Fig. 11.
Fig. 3 : The plots for transformer 1 is w.r.t left-y-axis, & transformer 2 is w.r.t right-y-axis. Both y-axis have same scale.
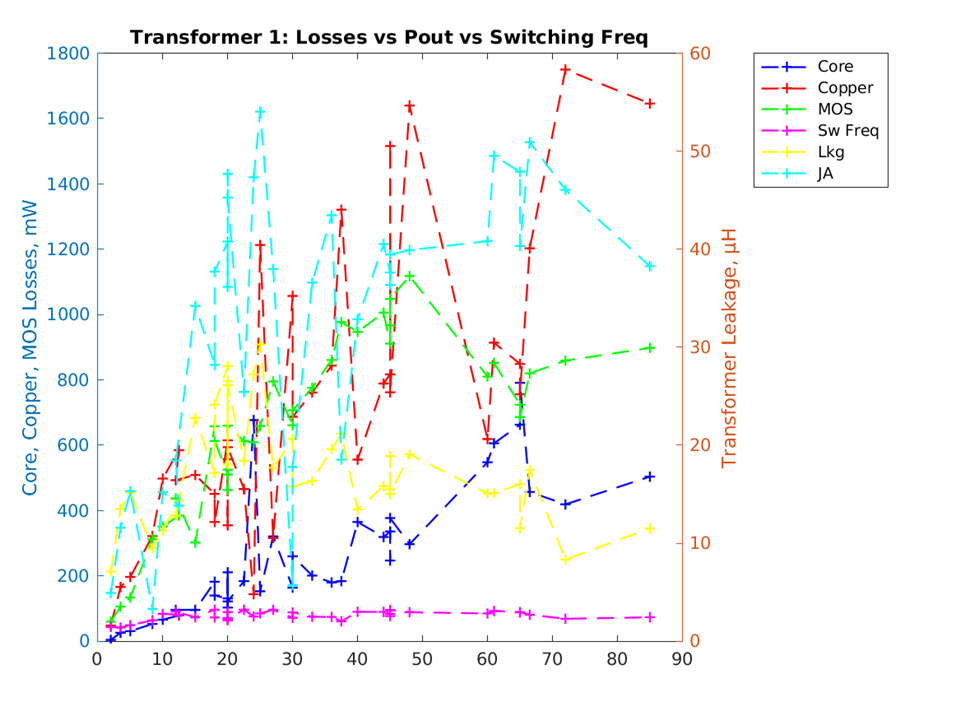
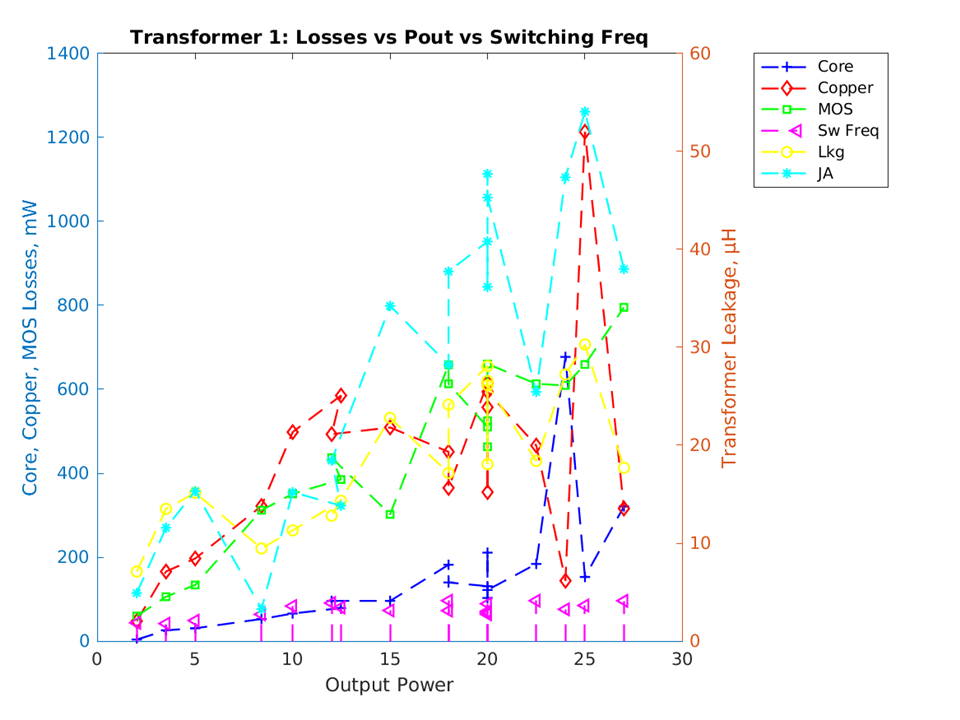
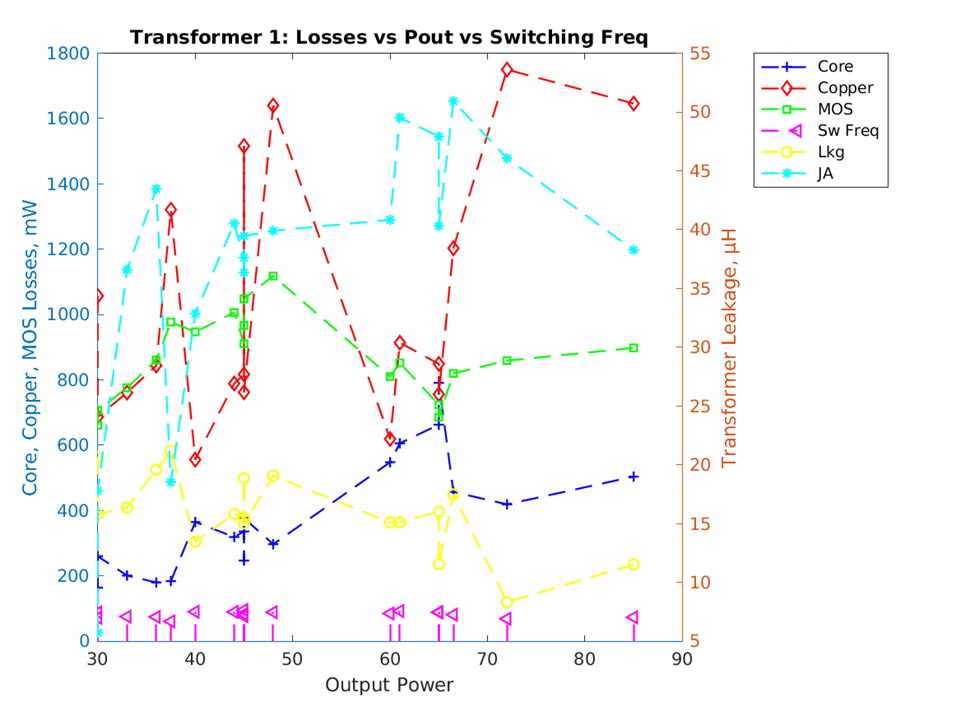
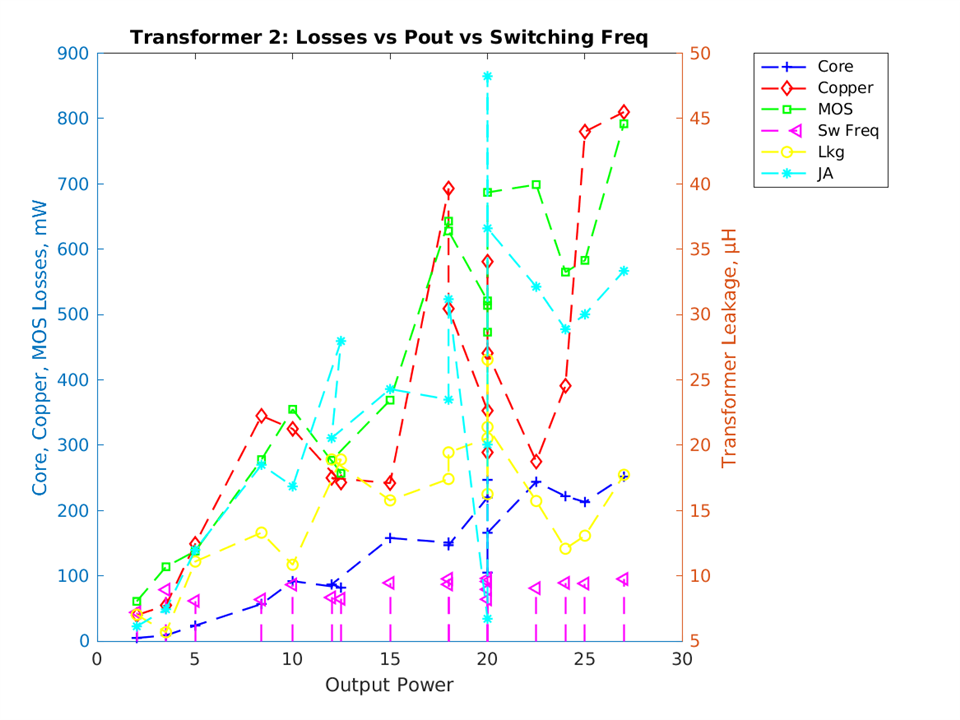
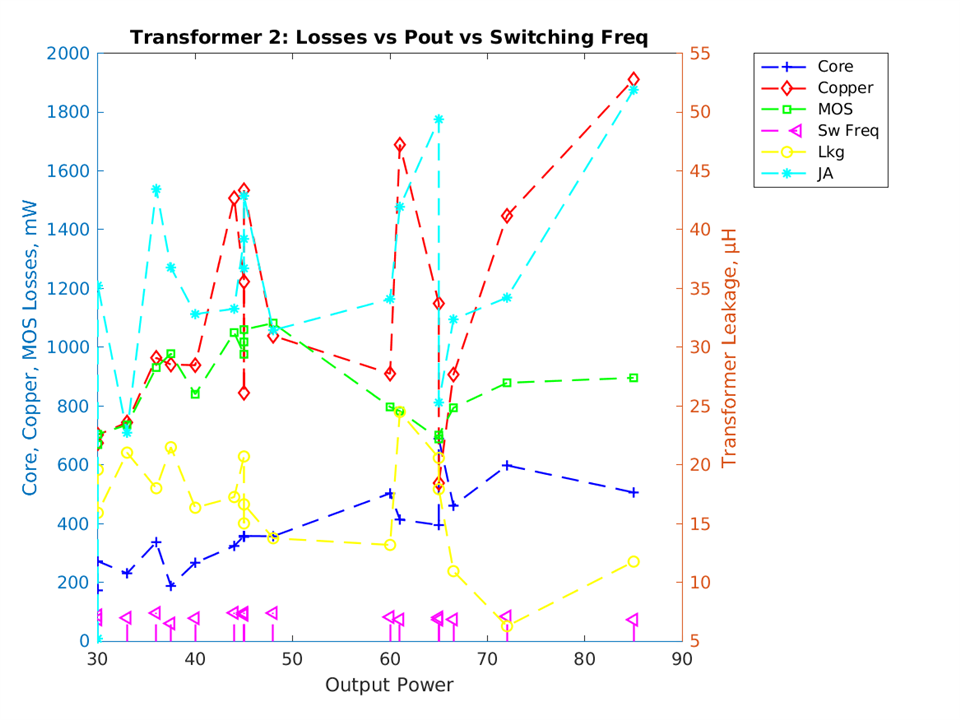
Fig. 4 : The plots for transformer 1 losses in mW - core, copper, MOSFET & hysteresis losses from JA perturbation simulation are w.r.t left-y-axis. The transformer leakage losses in μH is w.r.t right-y-axis.
The transformer switching frequency is in kHz, uses left-y-axis scale plotted as points(arrow).
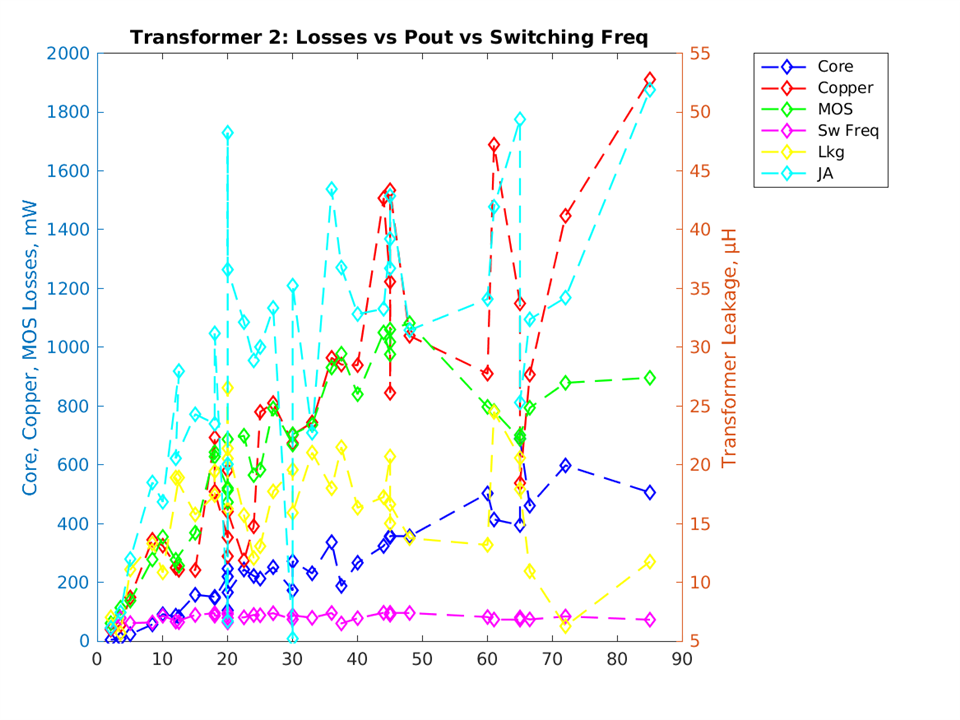
Fig. 5 has similar structure for transformer 2.
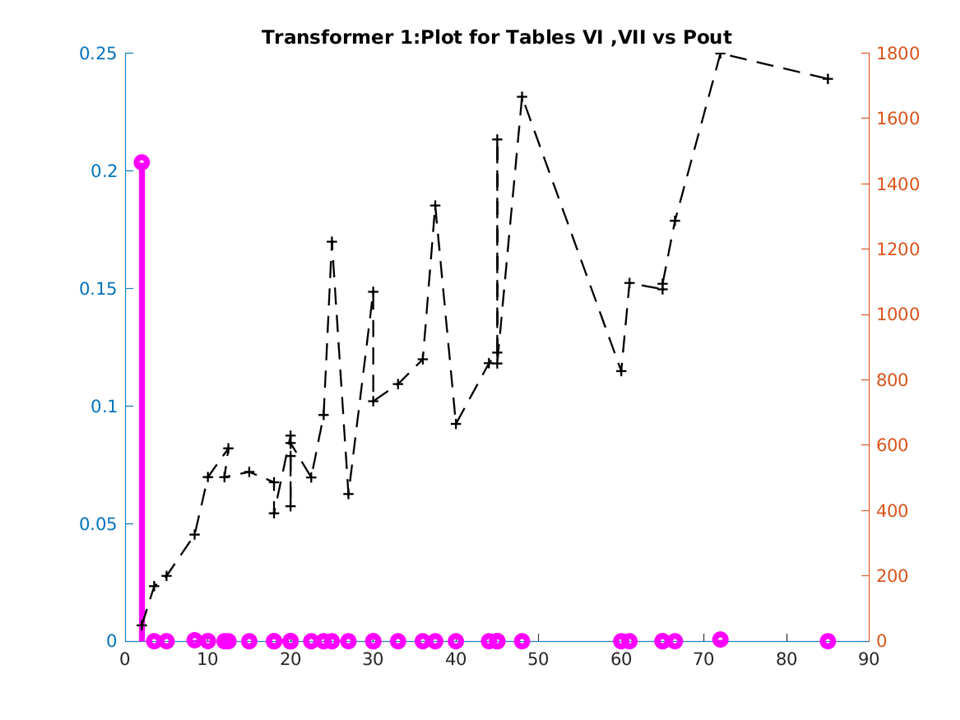
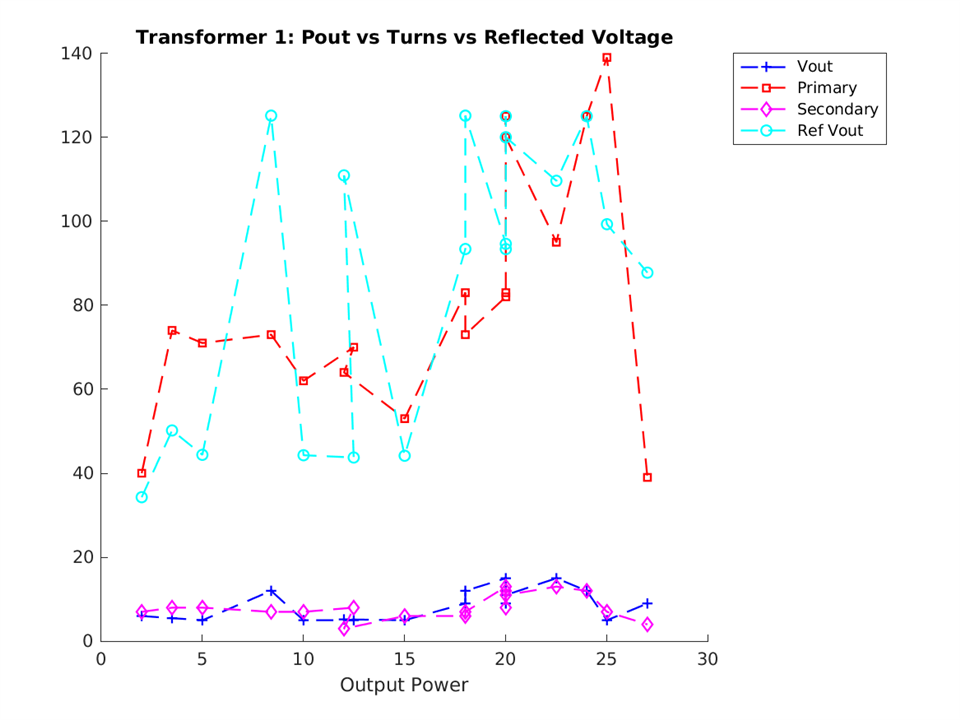
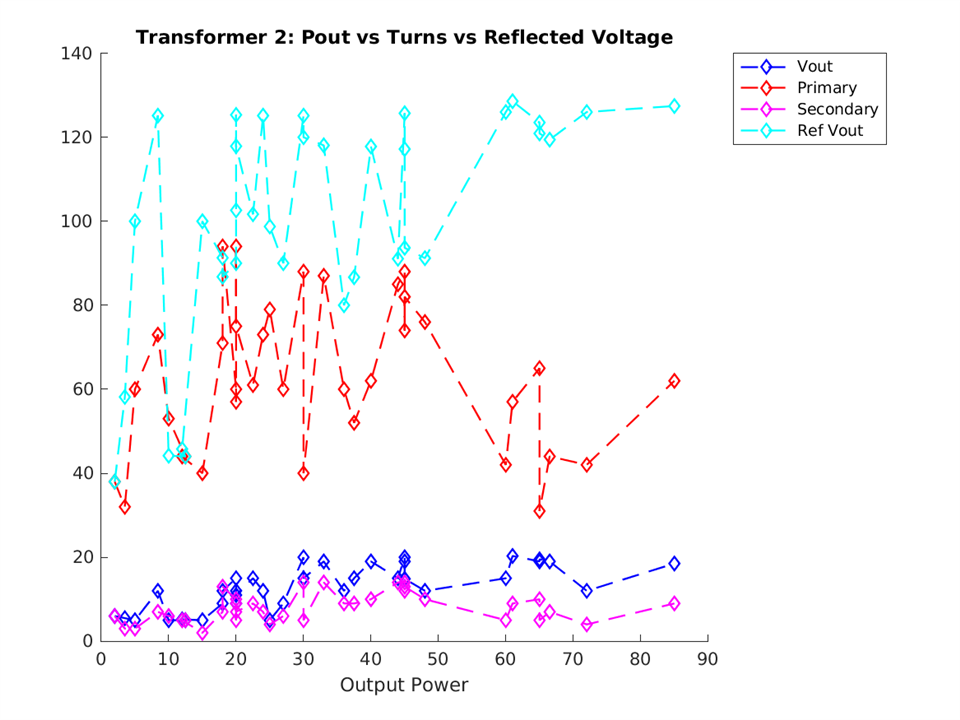
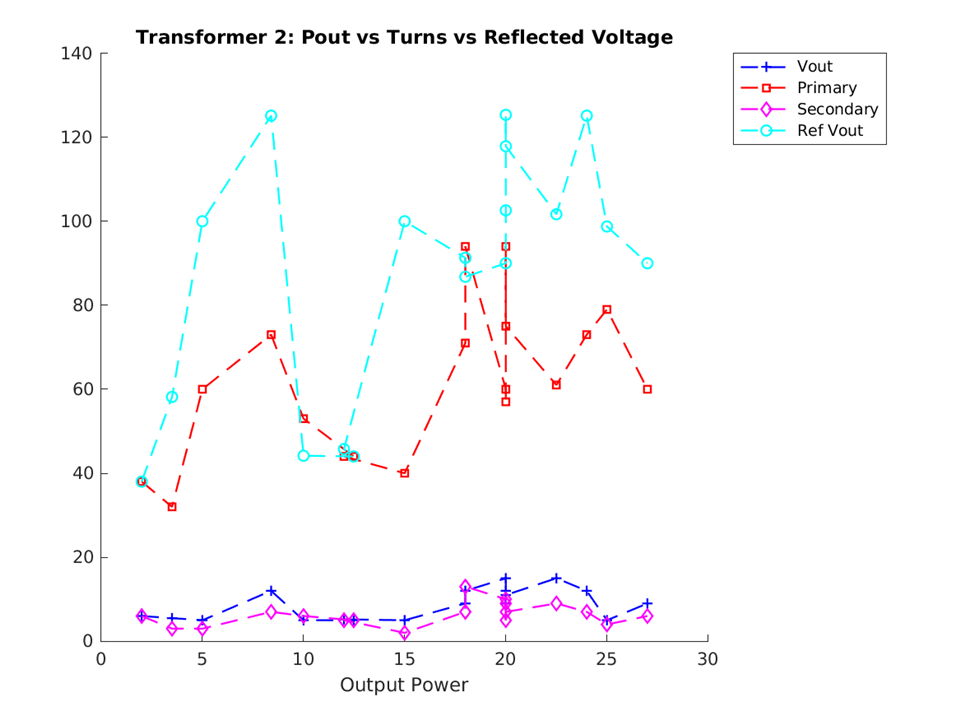
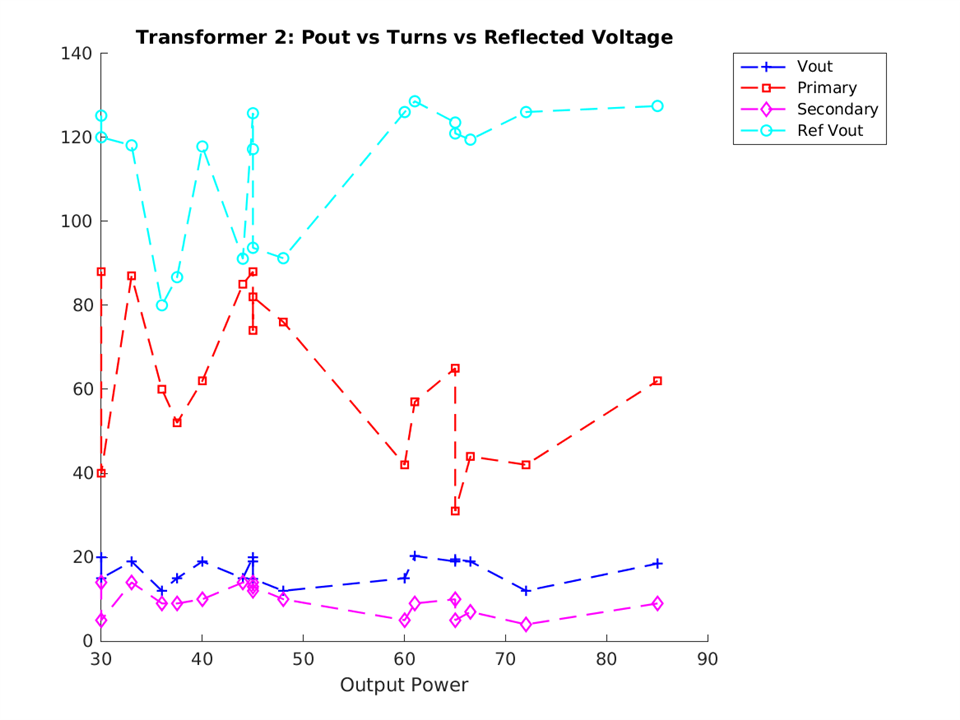
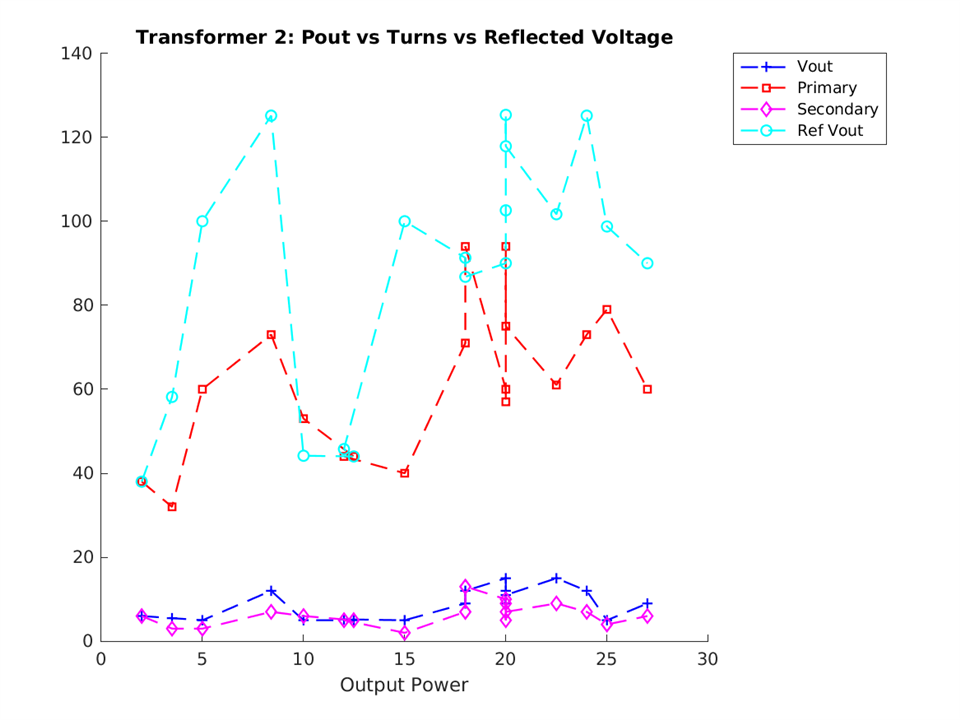
Fig. 6 & Fig. 7, shows the plots of output voltage, reflected output voltage, number of primary & secondary turns, for transformer 1 & transfomer 2 respectively. It can be seen that the reflected output voltages,Vor are even ≈ 20 times higher than as, in Vout = 5V ; ≈ 21.33 times higher than as, in Vout = 5.2V ≈ 11 times higher than as, in Vout = 12V ; ≈ 6 times higher than as, in Vout = 6V, and soon. These Vor if other core is used to design at the same voltage level.
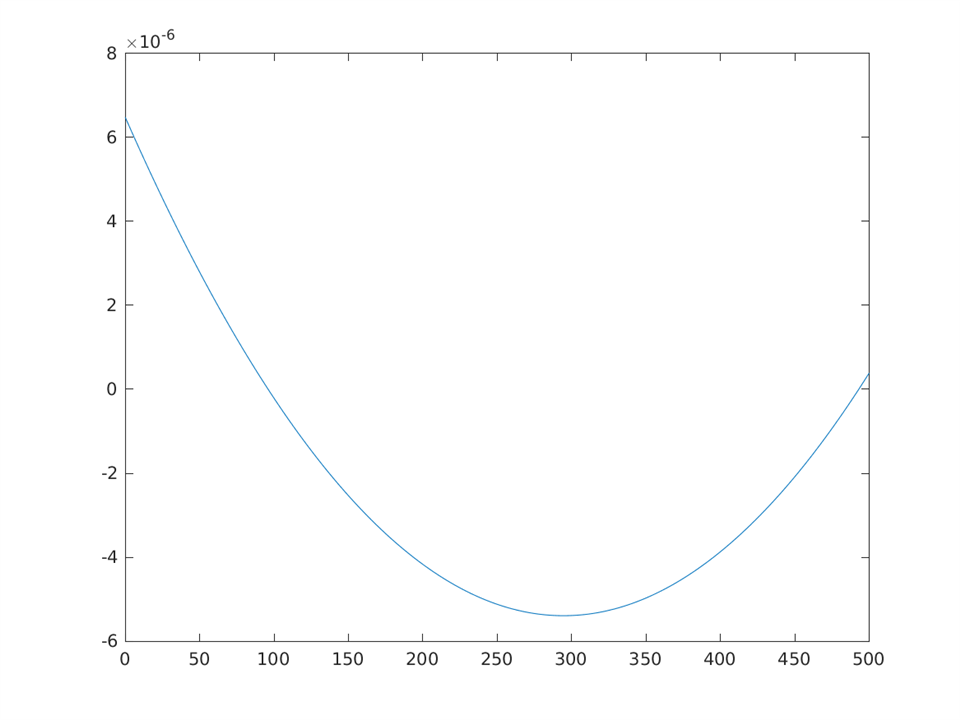
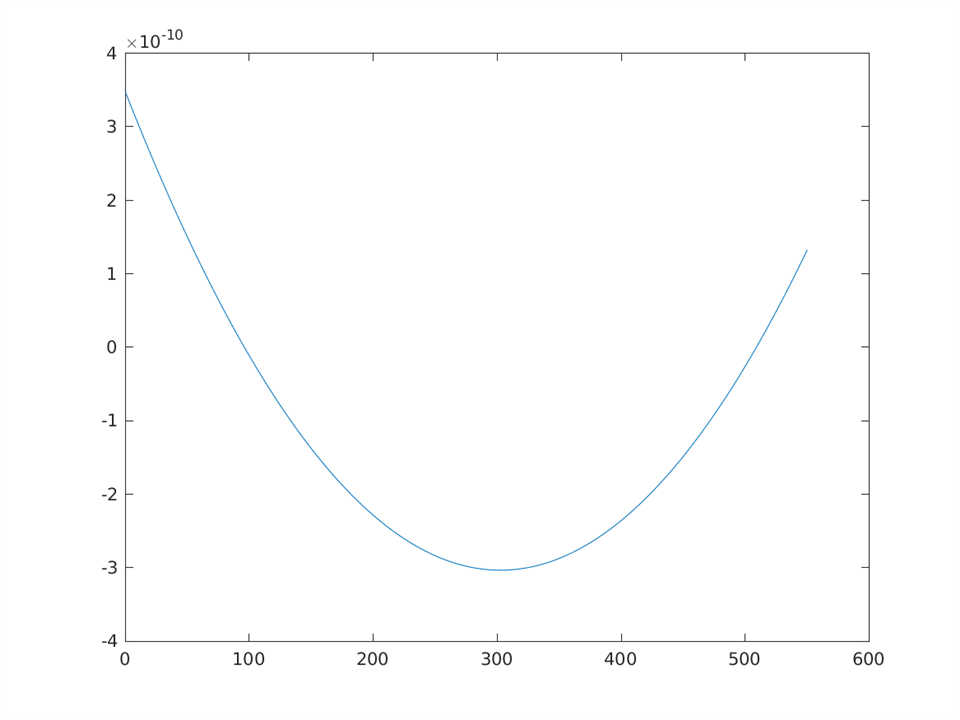
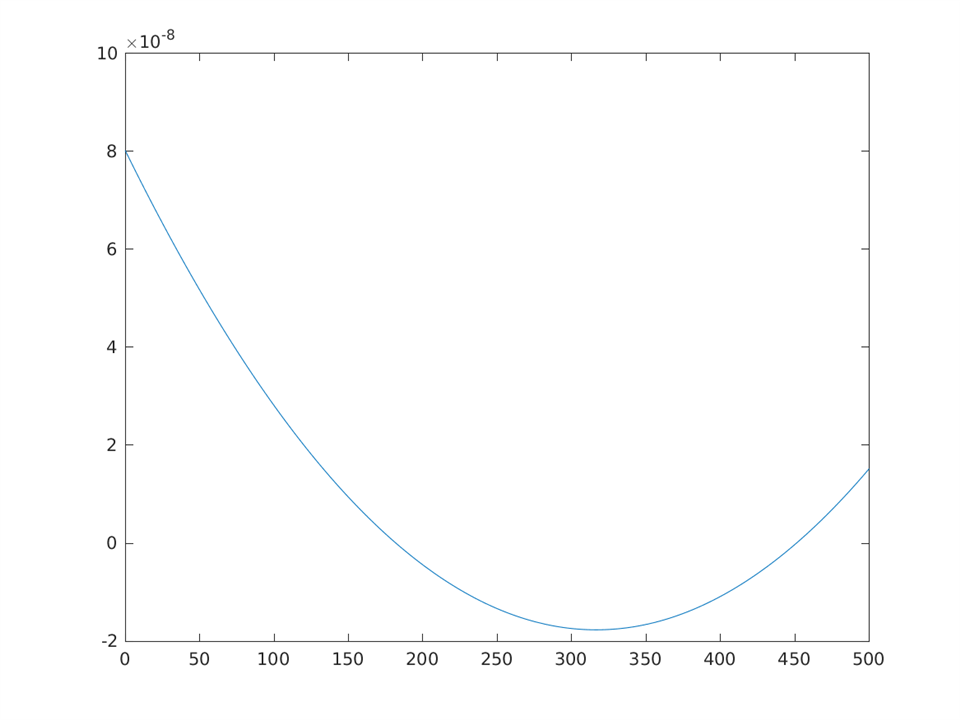
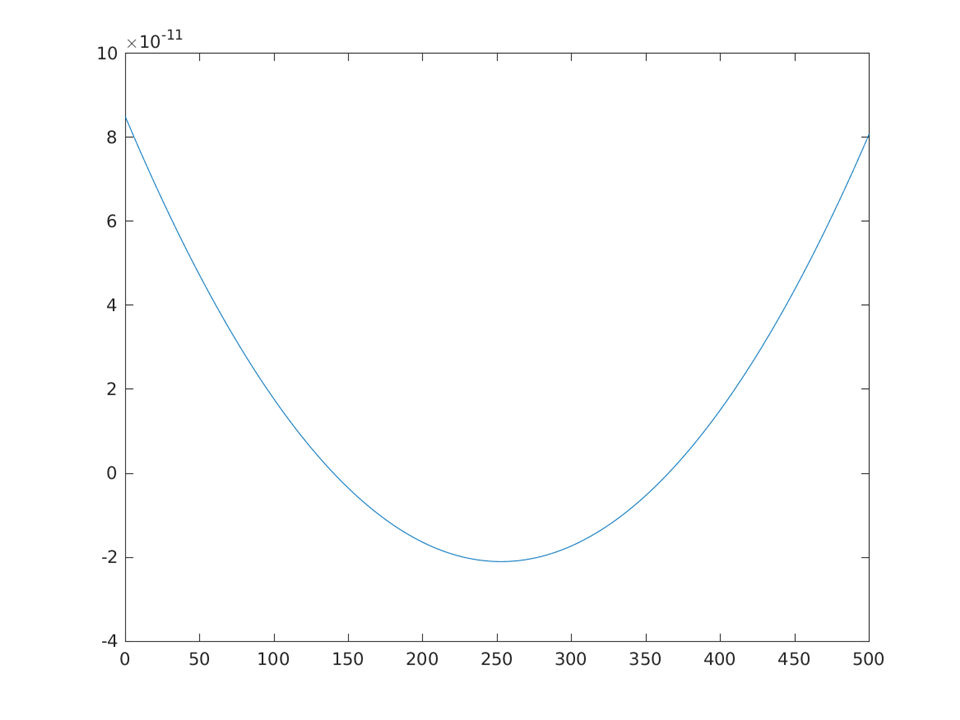
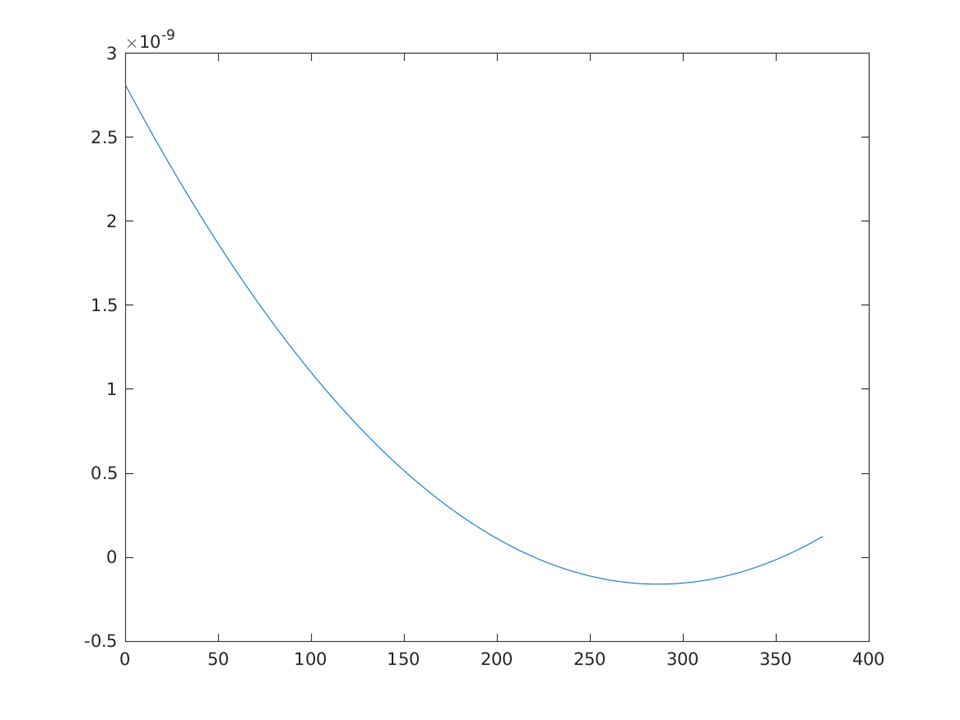
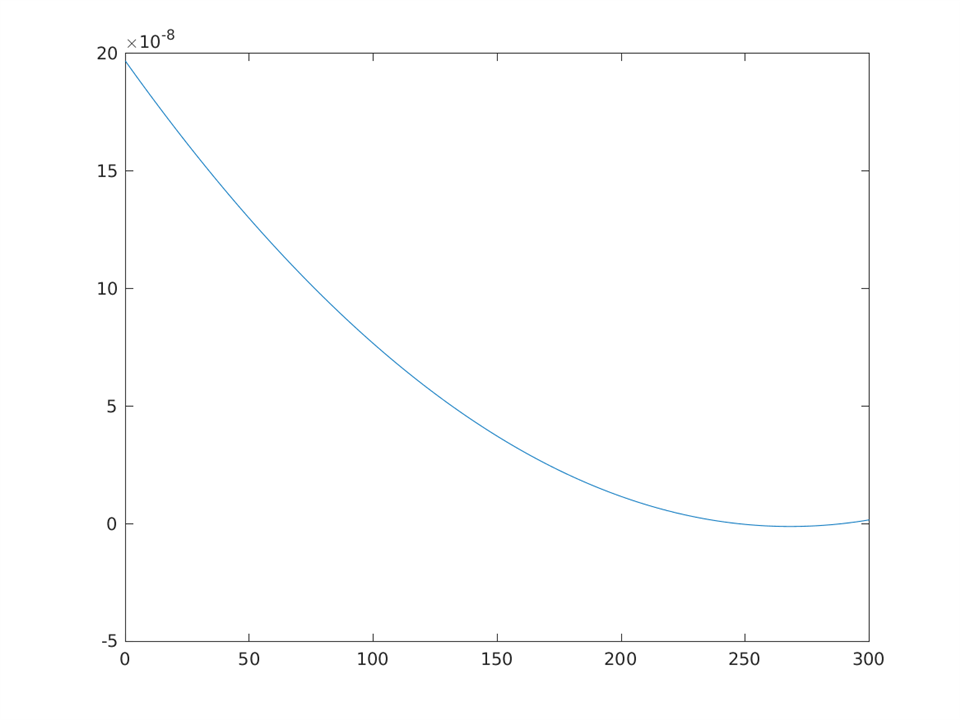
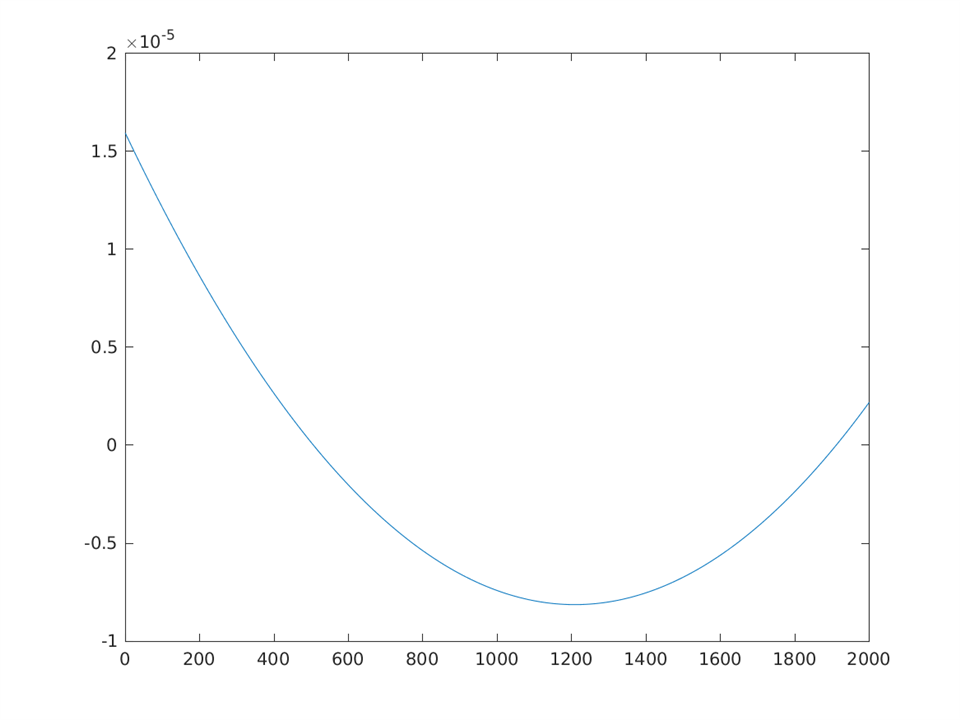
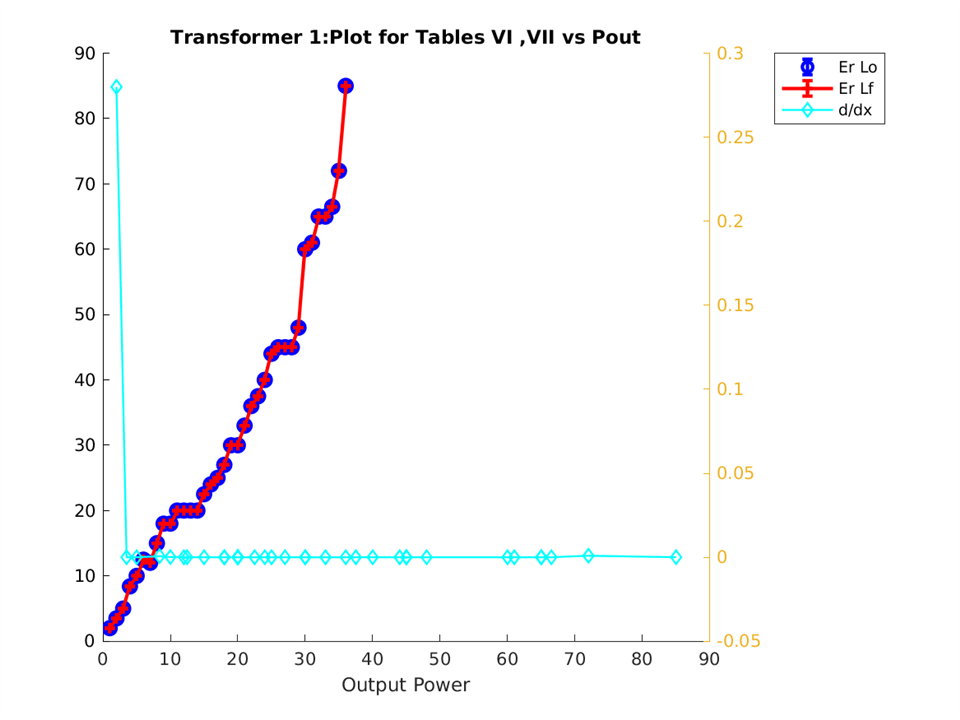
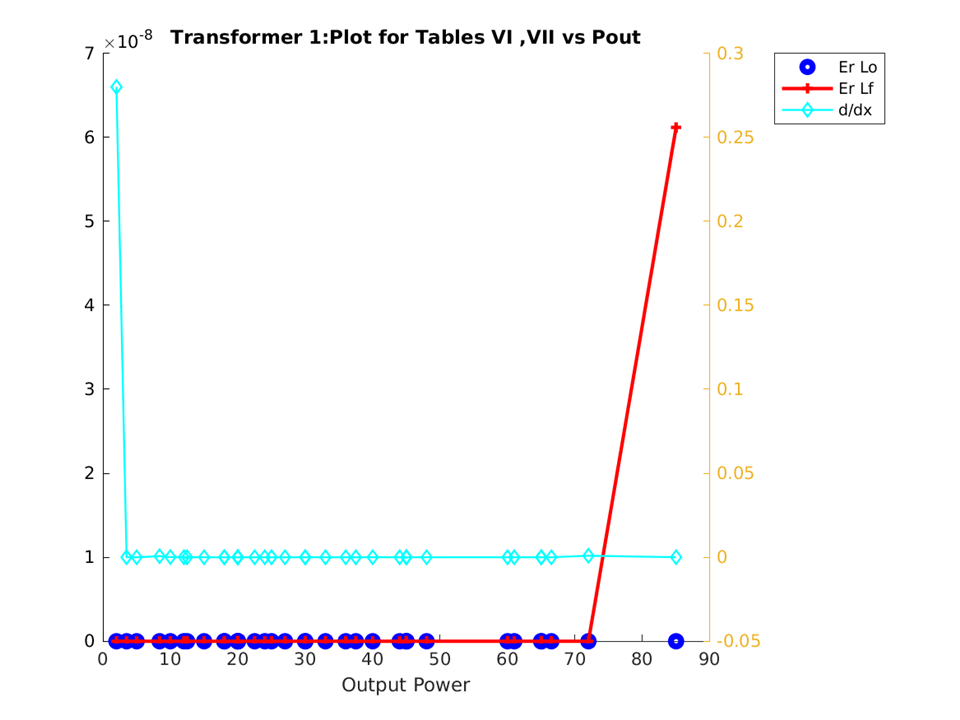
Fig. 8,9 : The plots for transformer 1,2 - residual errors of original loss function (ζLo ) & proposed loss function (ζLf ) are w.r.t left-y-axis. The Lyapunov first order derivative (L′ f ), are w.r.t right-y-axis. The left-y-axis & right-y-axis have different scales. The elevance of plotting these is to explore the co-relation between them.These again the complete different trajectory, if the other core is used. For transformer 1, there exists bijective mapping between both the residues, as these errors are in one-to-one relation. But for transformer 2, there is deviation at many points, and no bijective mapping exists.
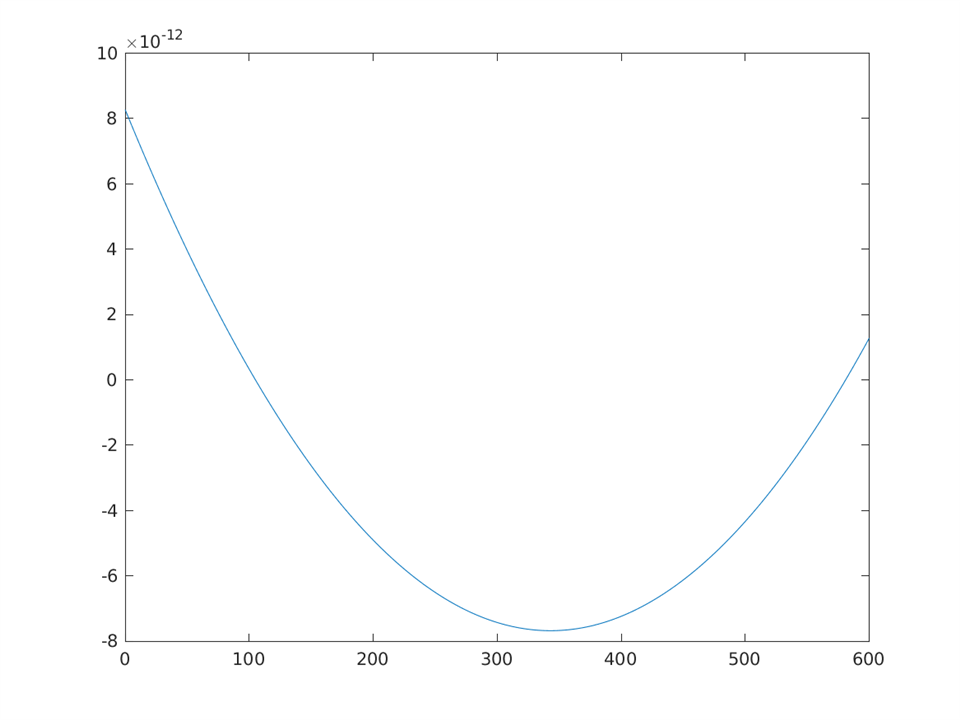
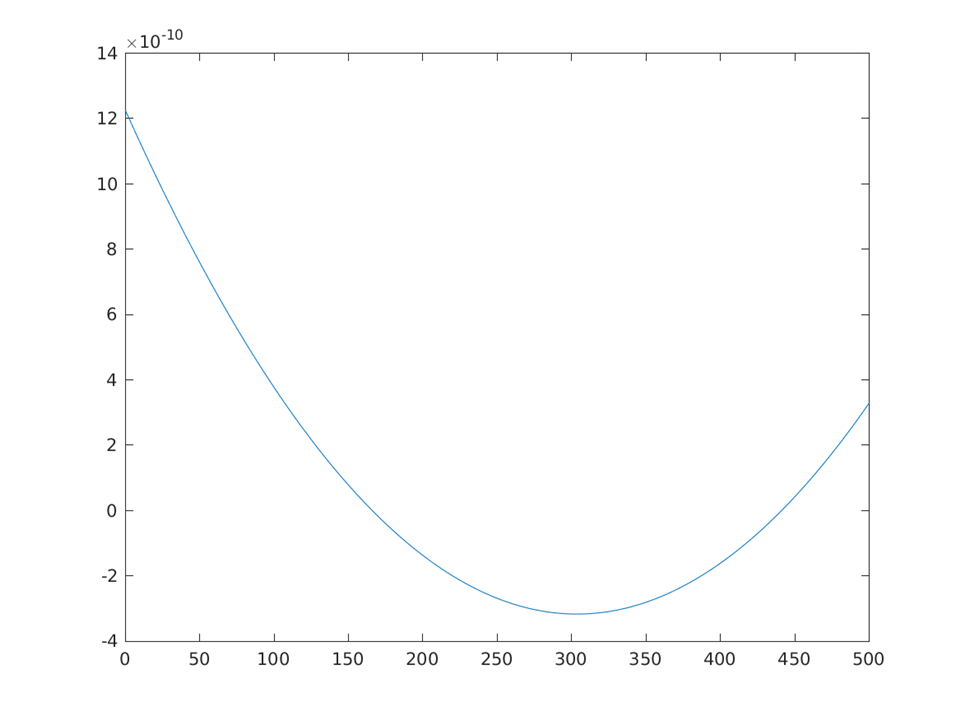
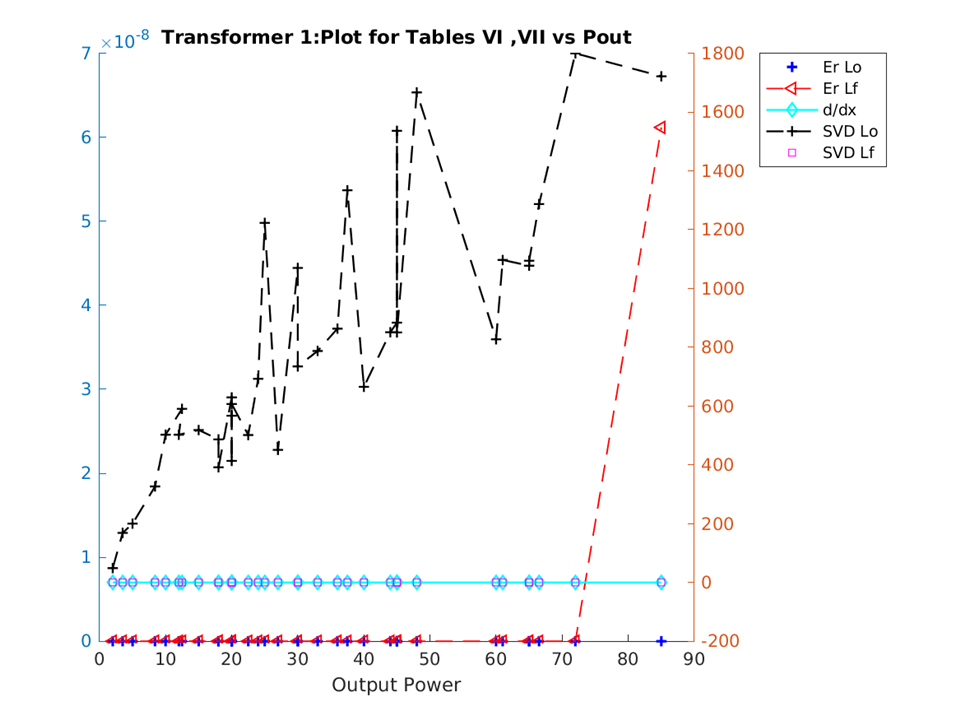
Fig. 10, 11 : The singular value decomposition of original loss function SVD(L) are w.r.t right-y-axis and has bigger scale, than the singular value decomposition of proposed loss functions SVD(Lf ), which are plotted w.r.t left-y-axis, and has micro-scale. There is huge difference in the values between them.
All the above plots (3-11) prove the variation in the parameters (which are in mW or μH) do not increase or decrease, at the same rate w.r.t increasing output wattages or even at the same output power but at different output voltages with ∆ = 0.2 V or 2 V! ⇒ highly non-linear.
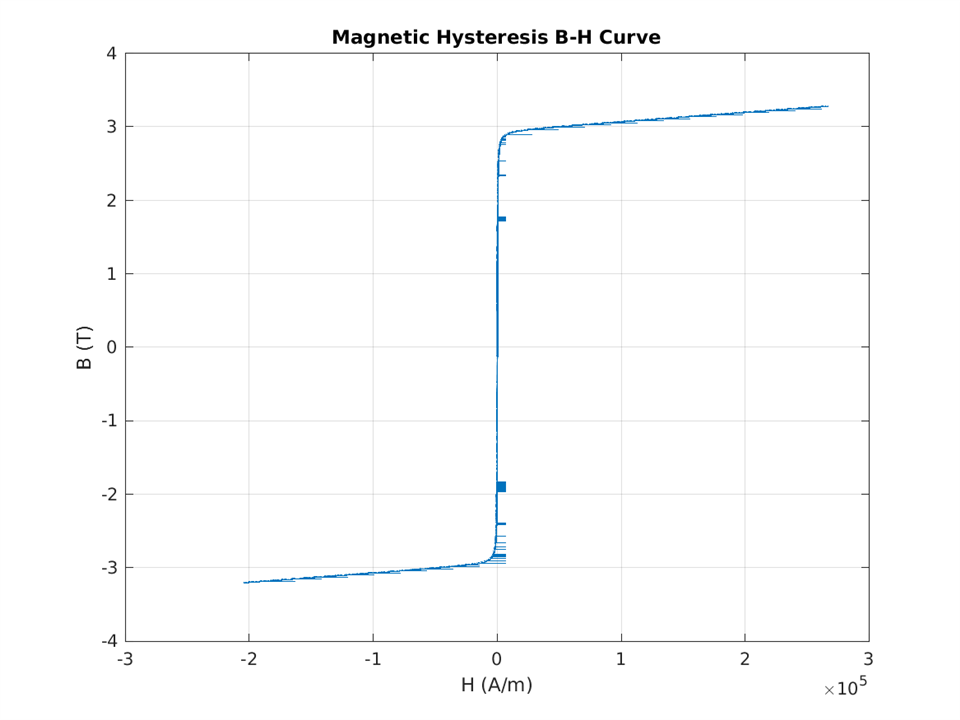
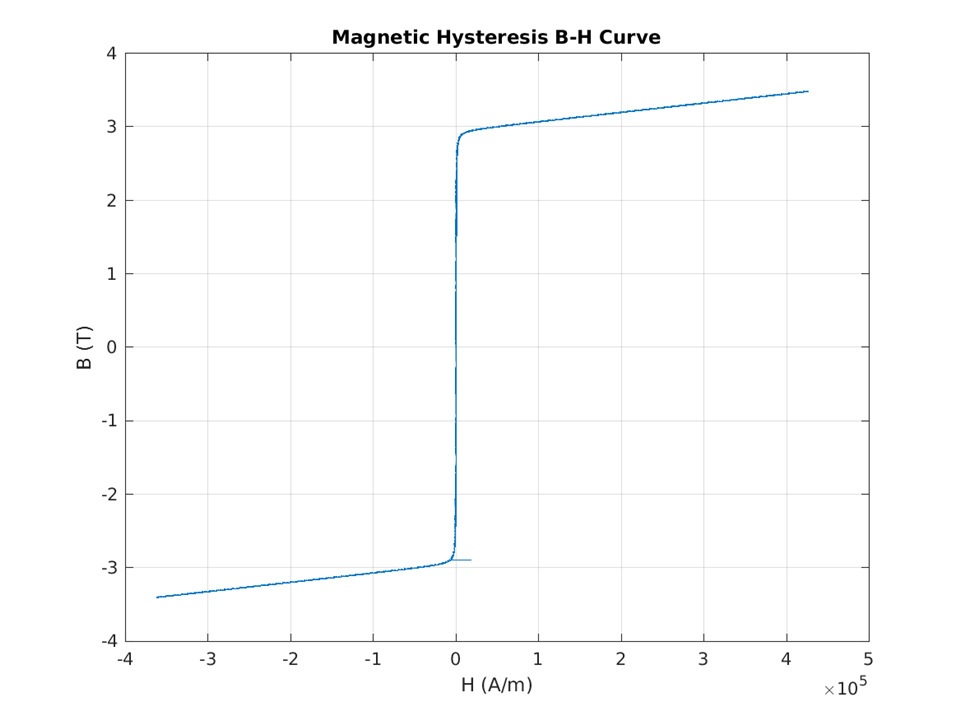
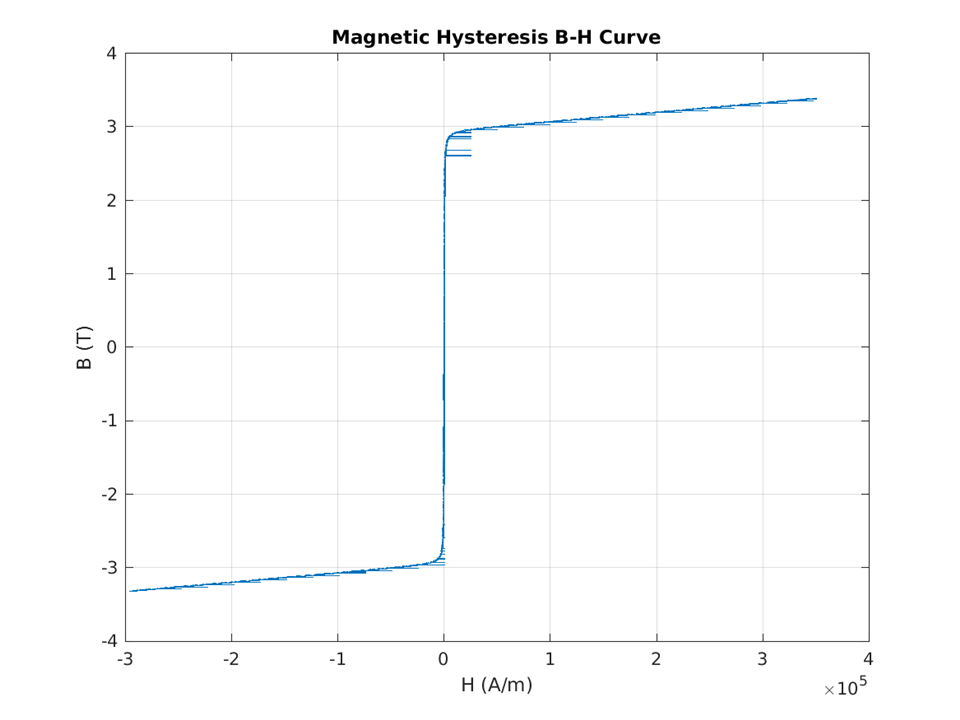
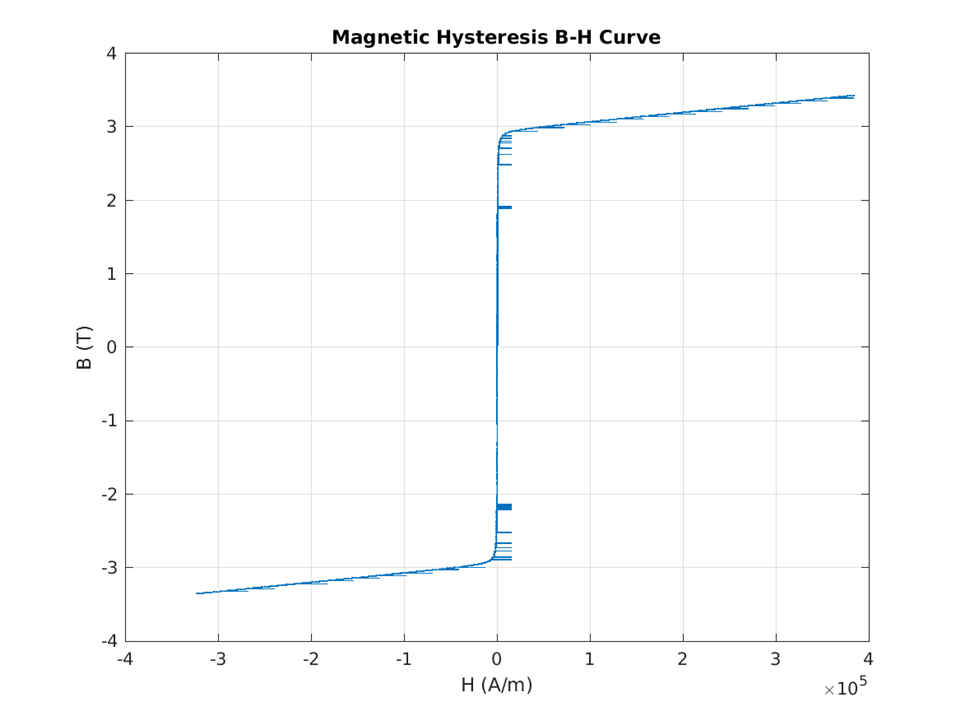
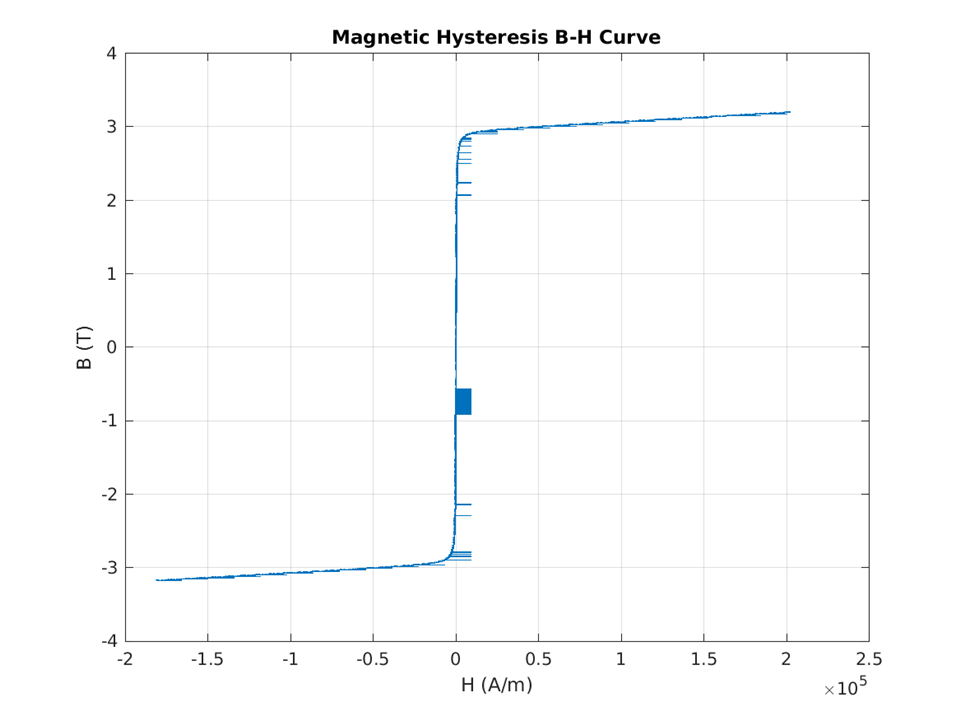
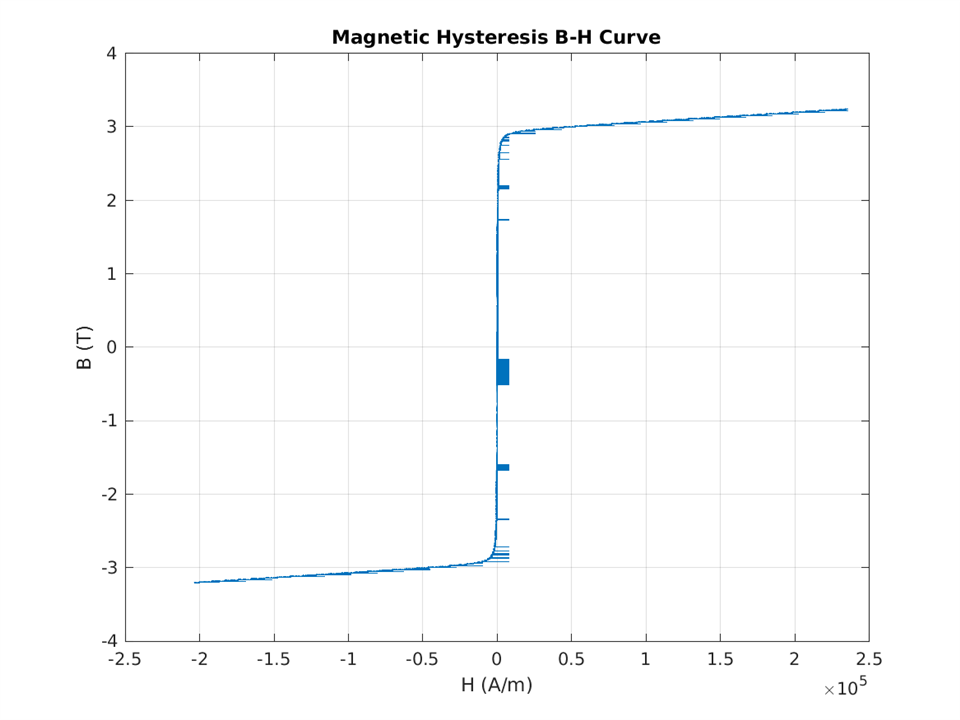
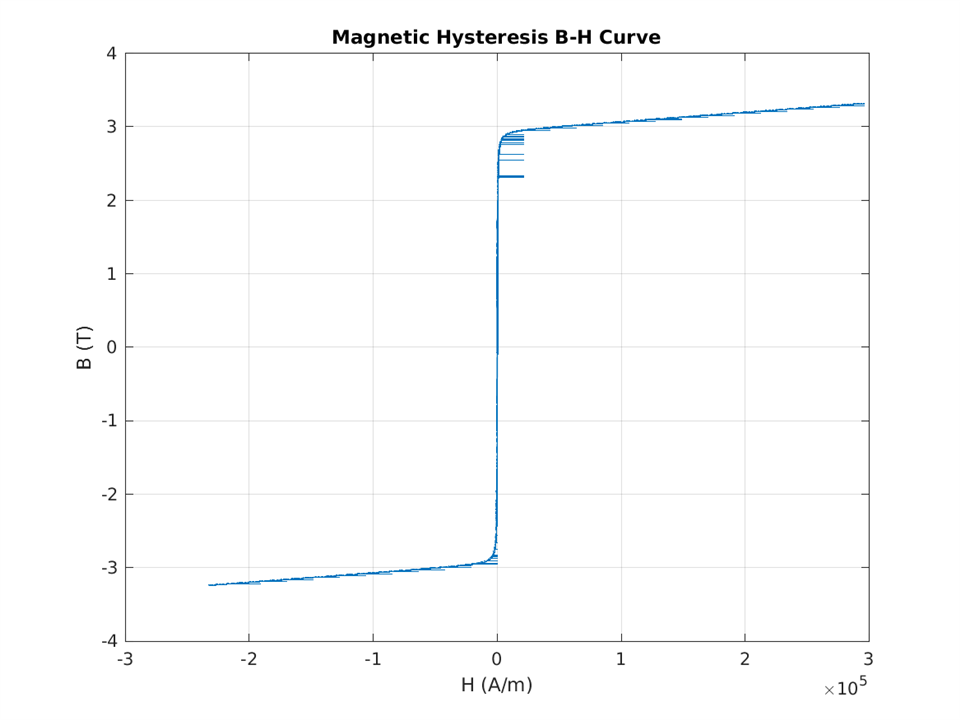
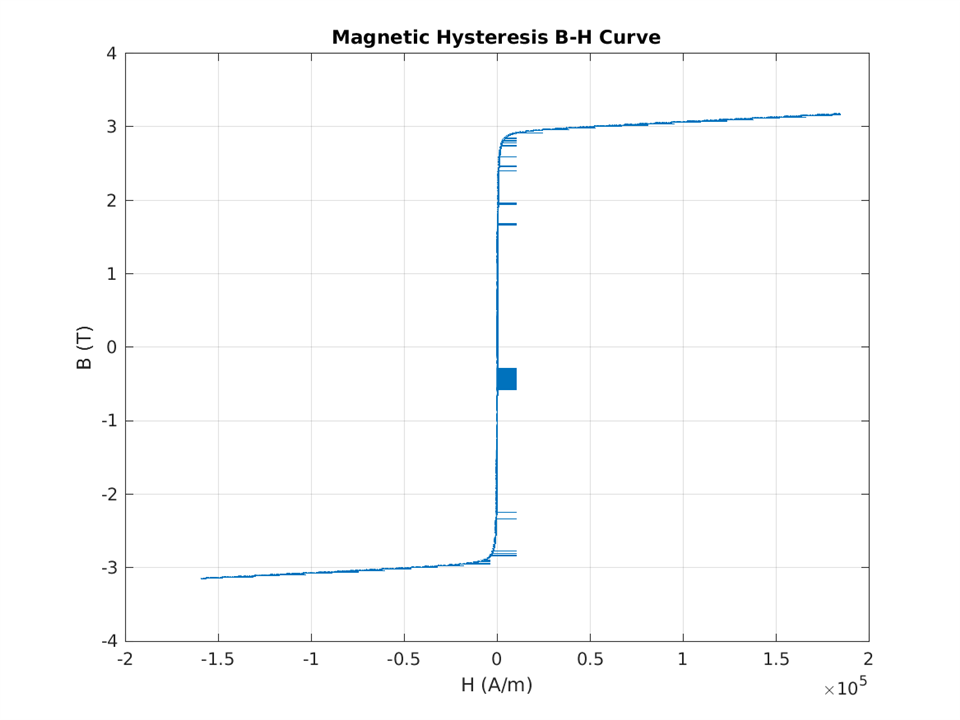
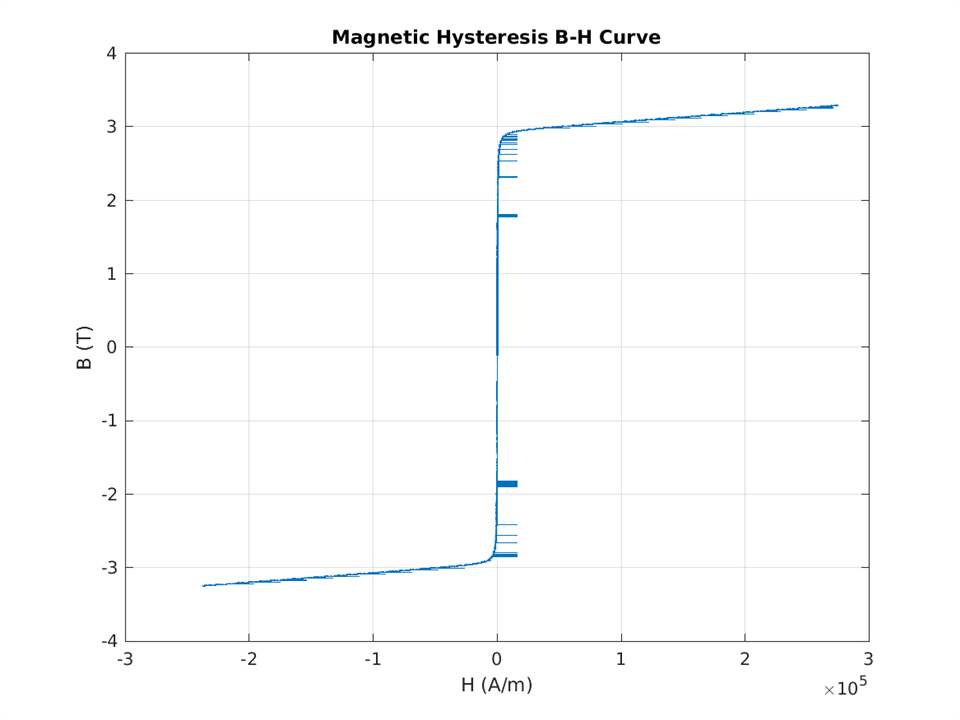
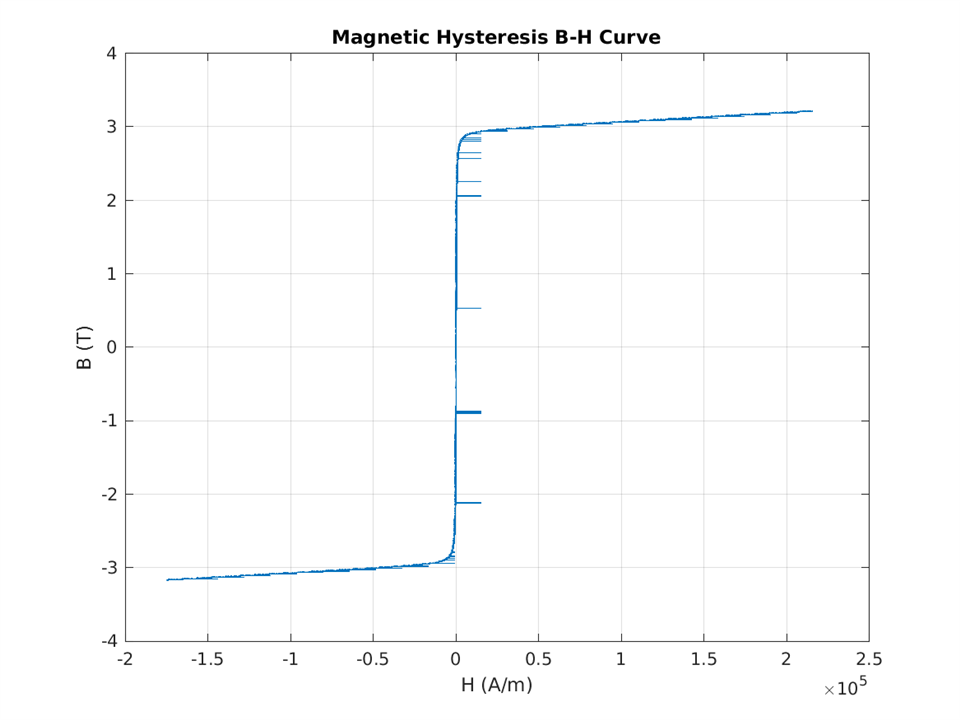
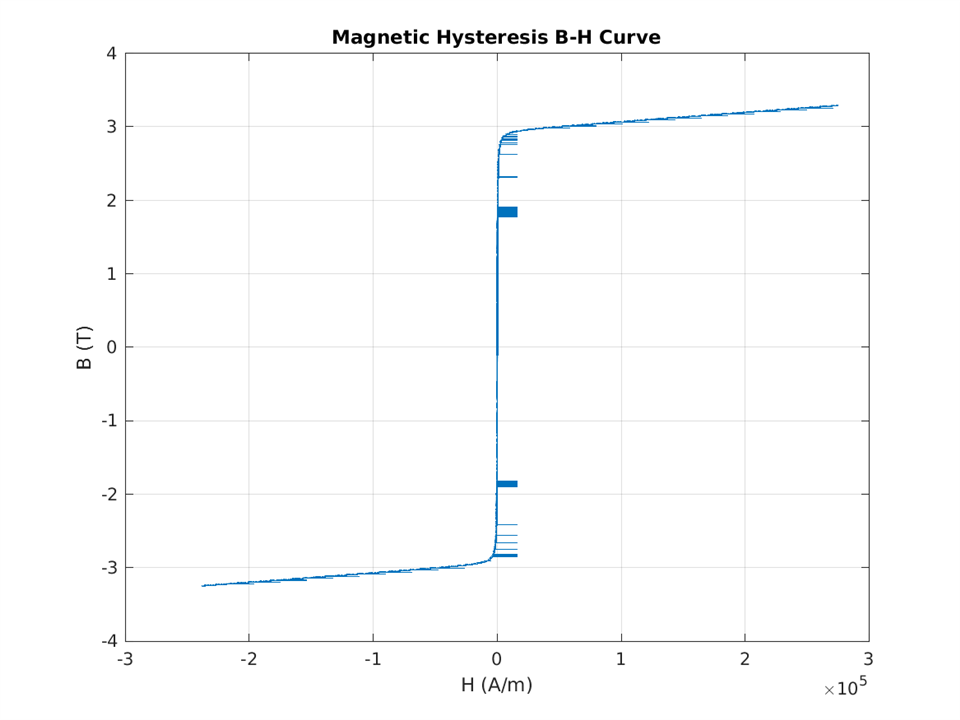
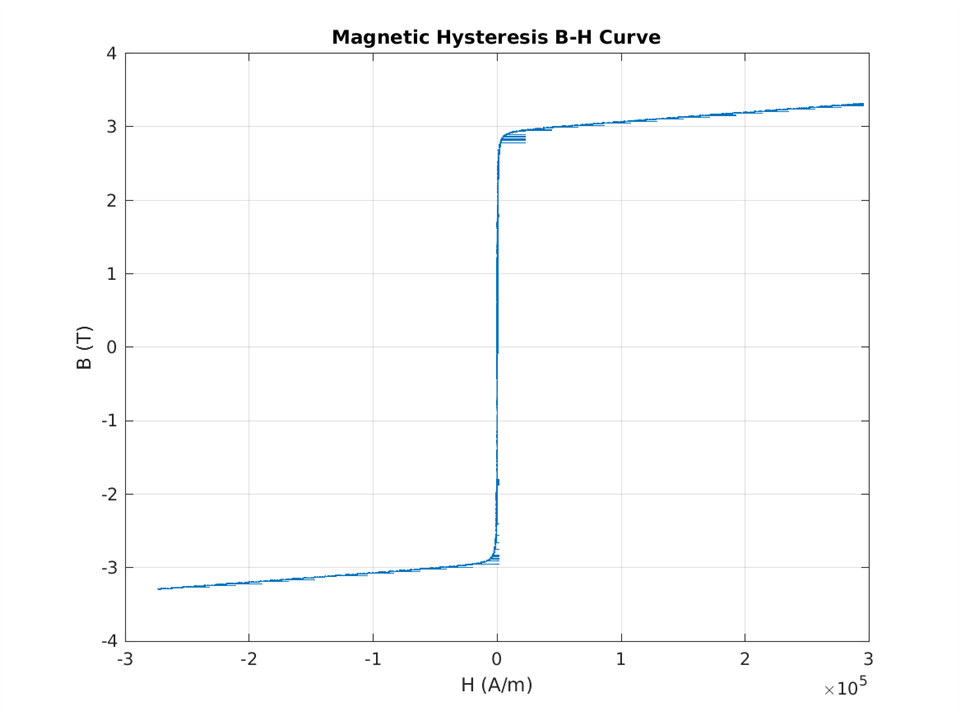
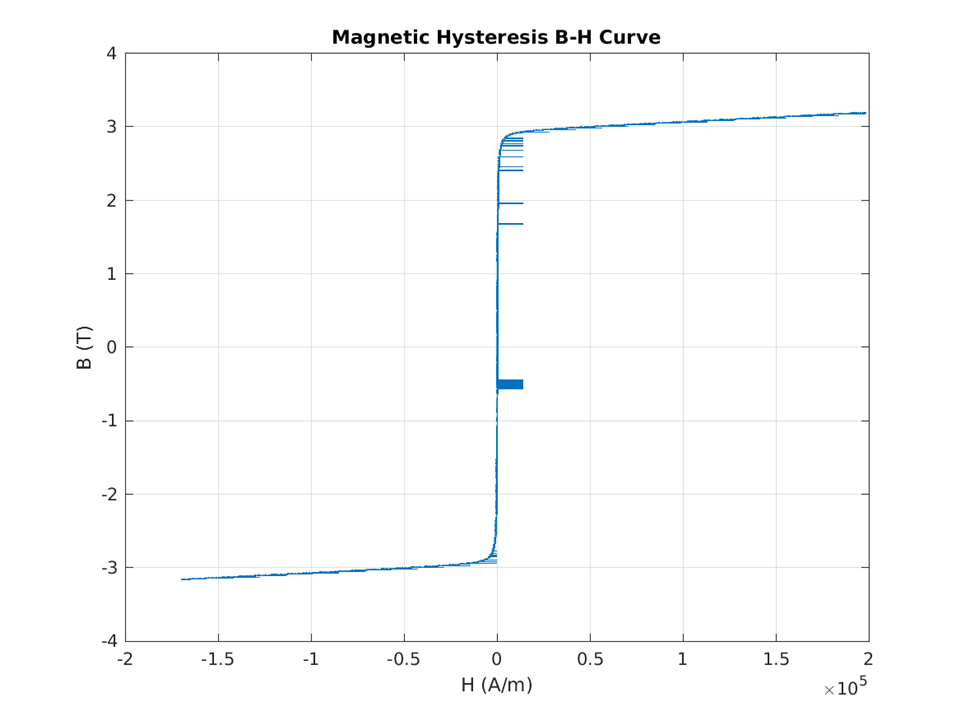
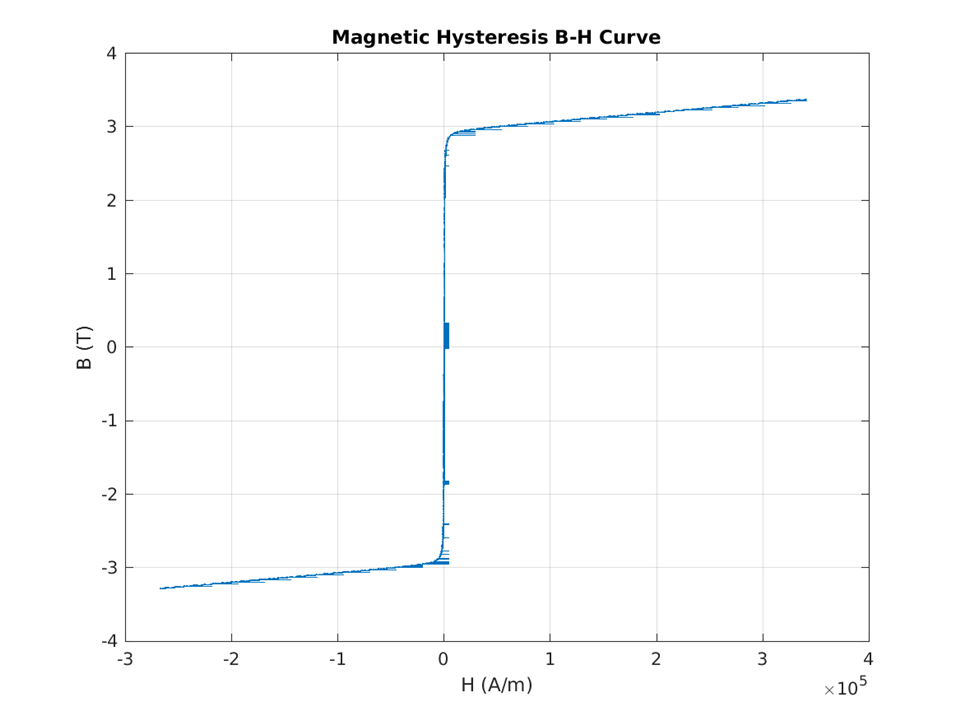
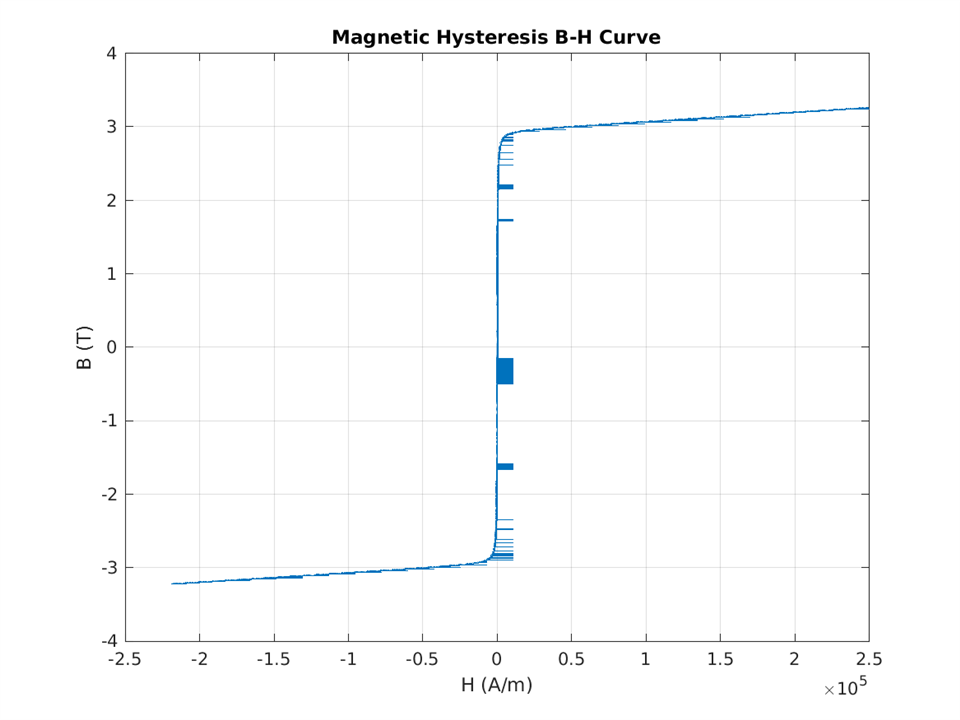
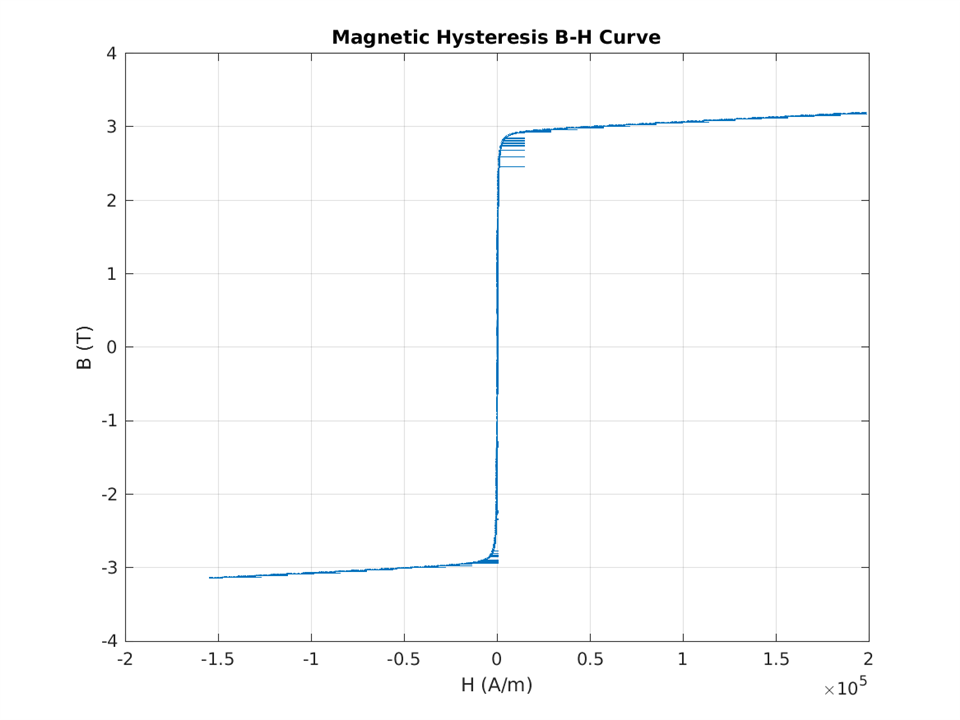
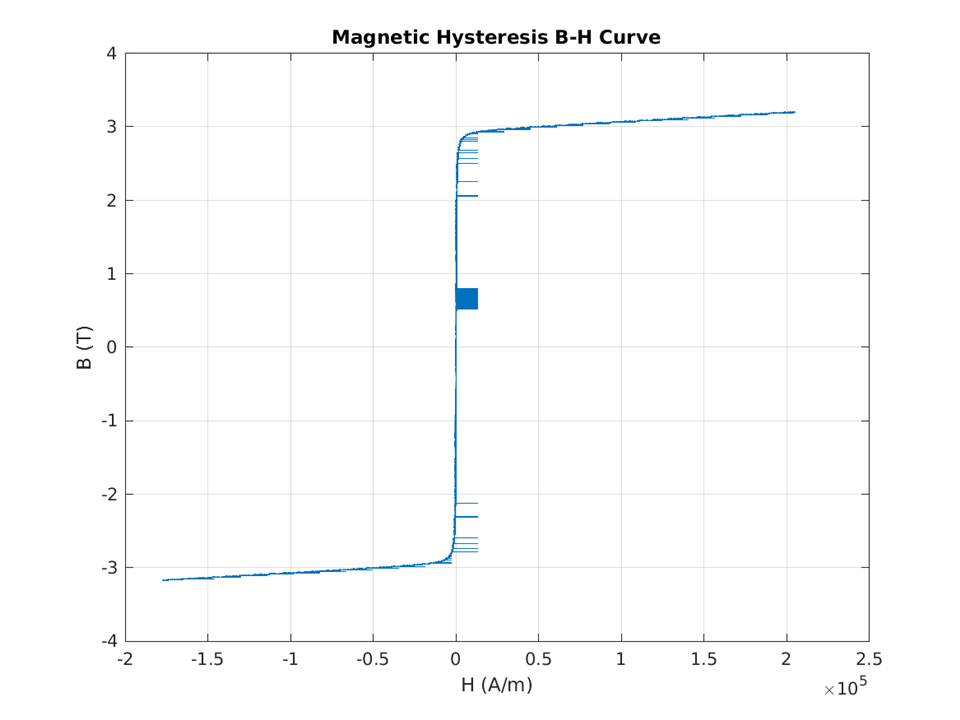
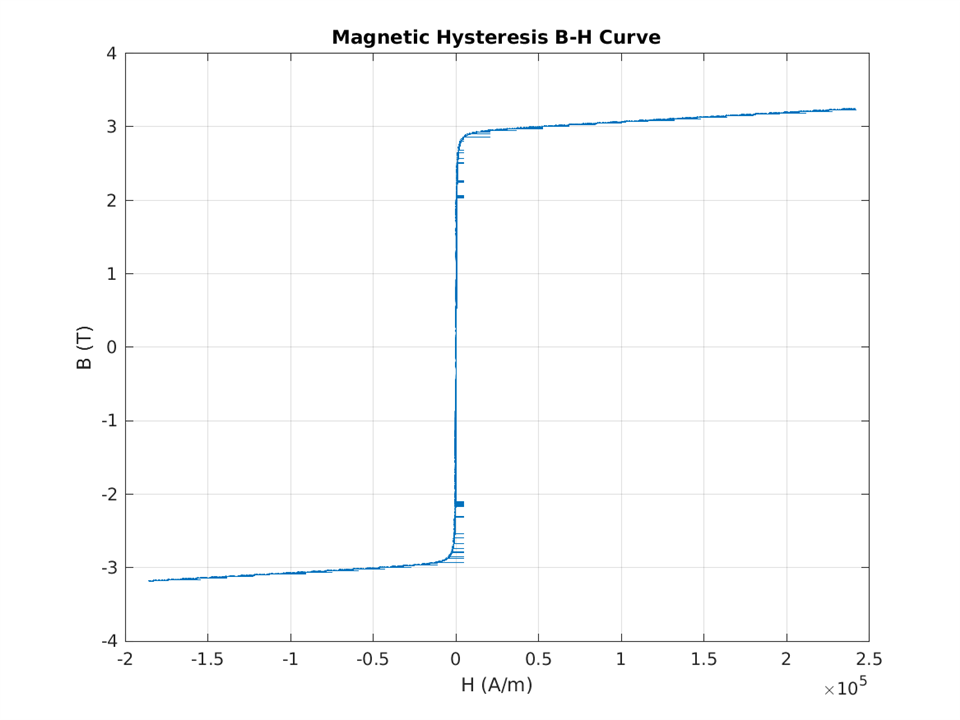
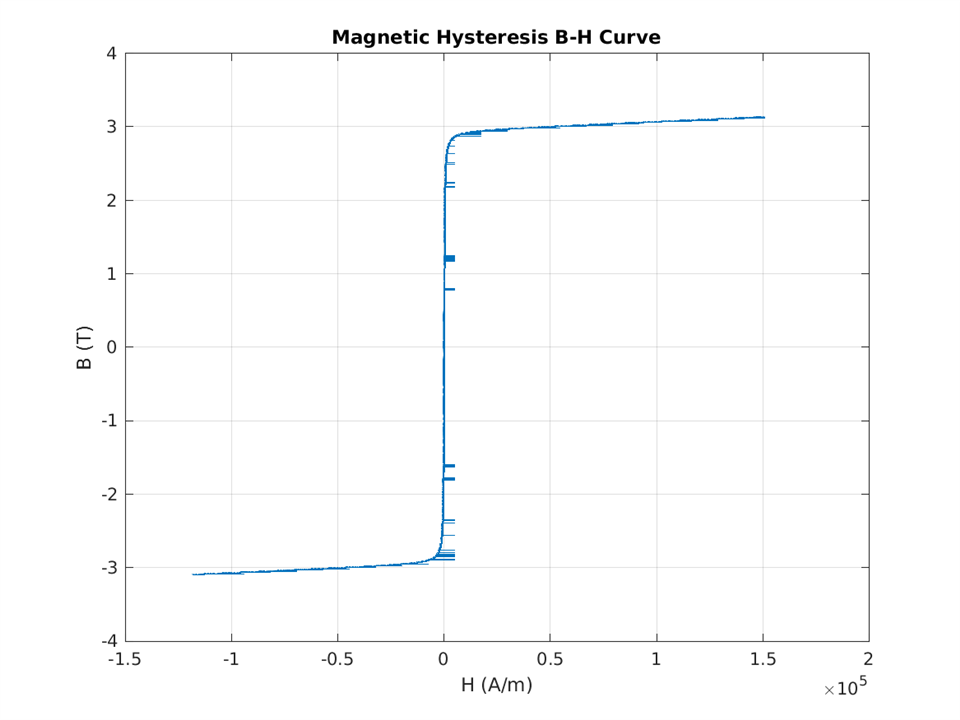
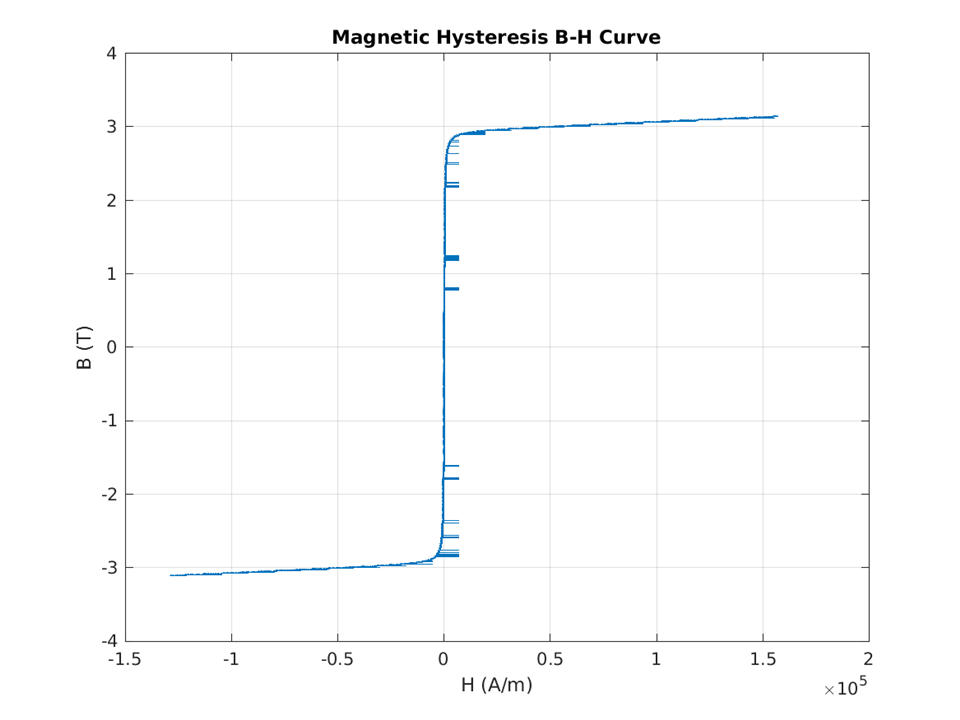
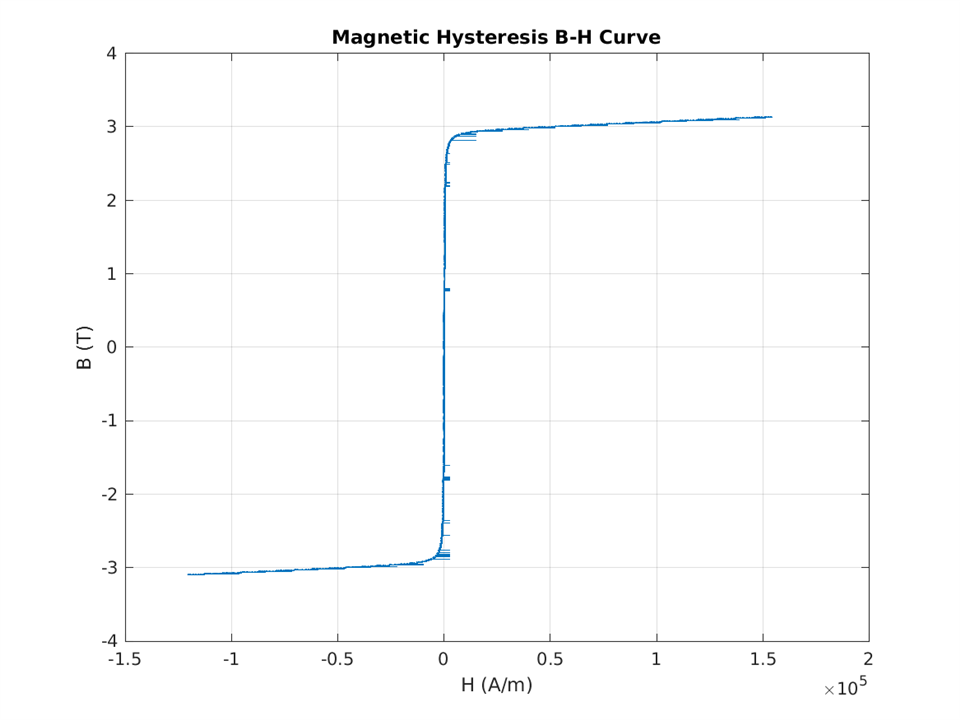
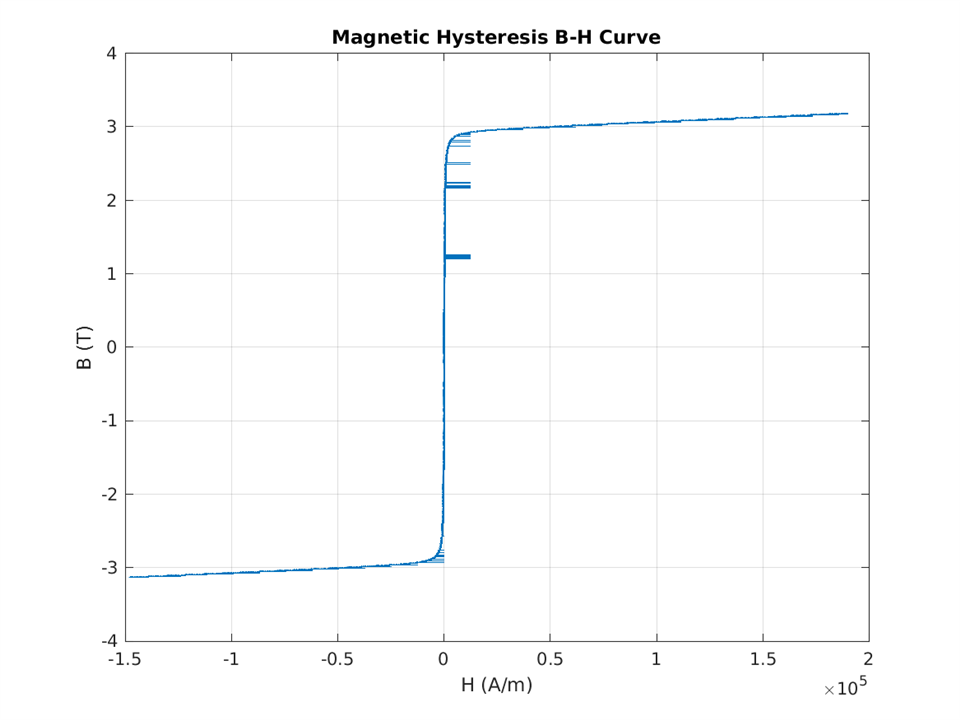
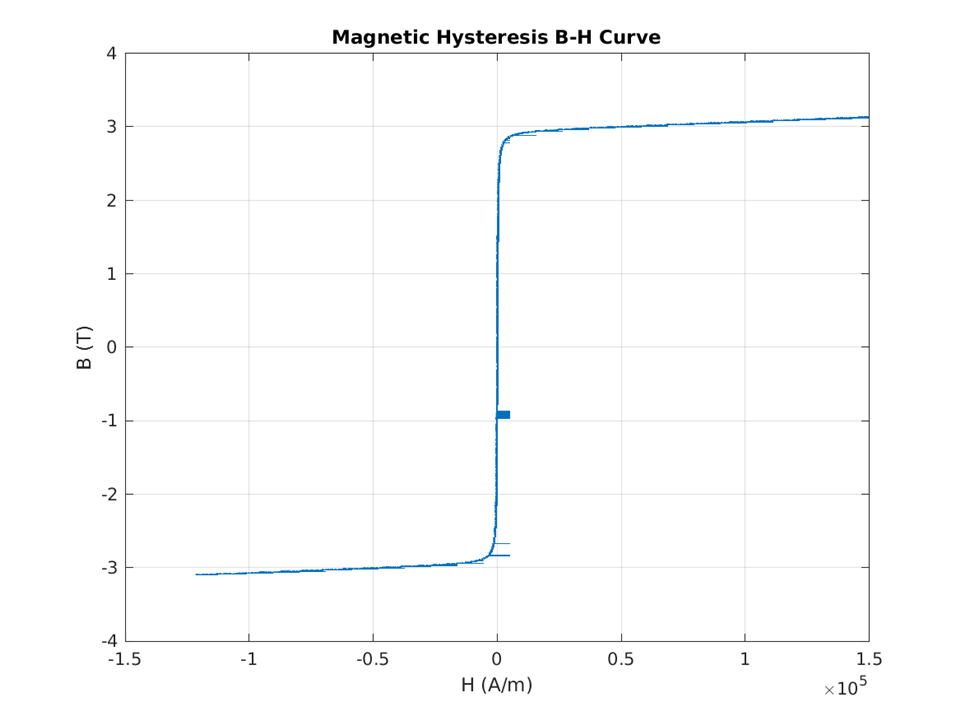
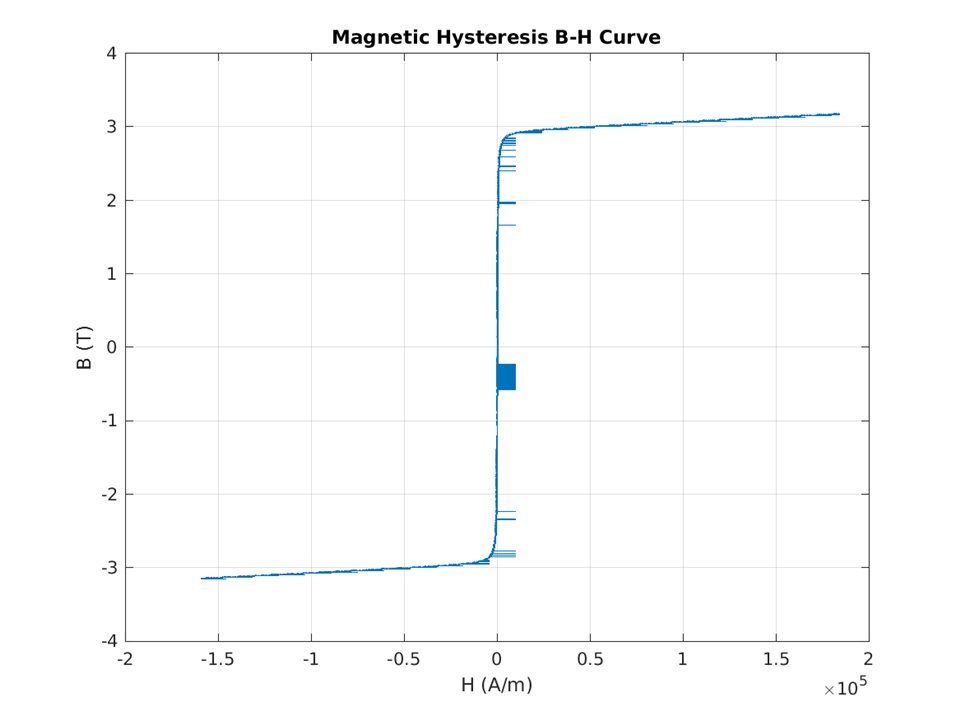
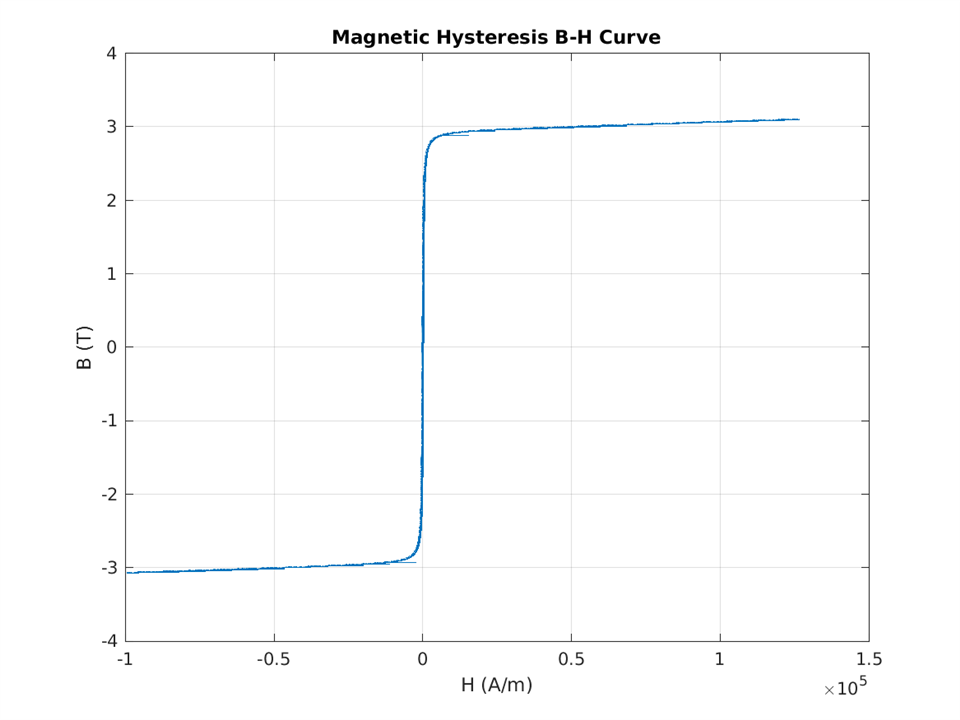
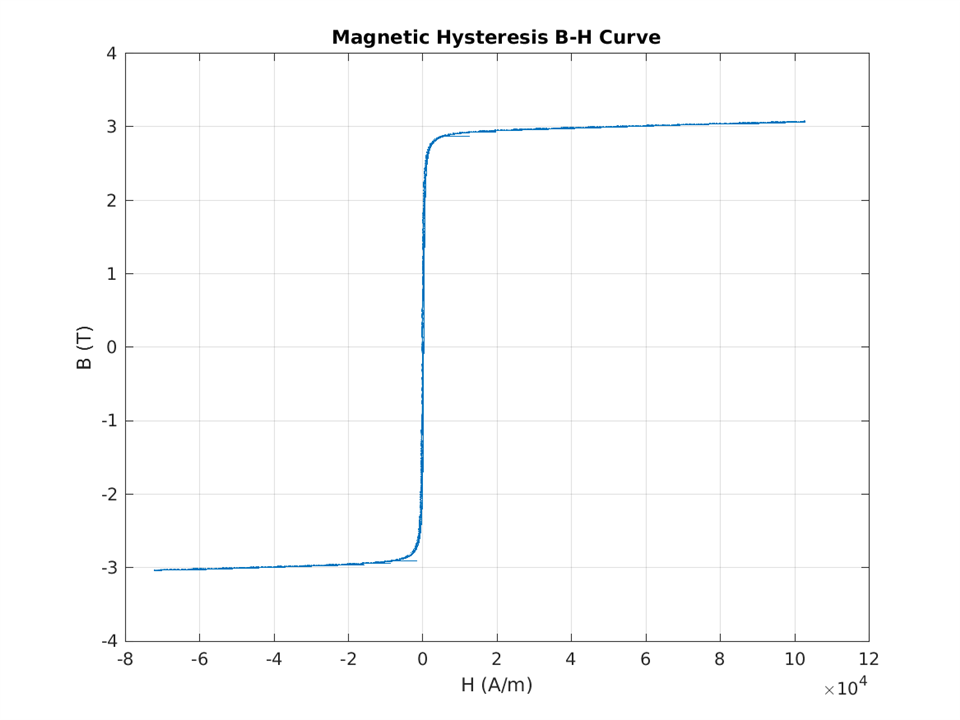
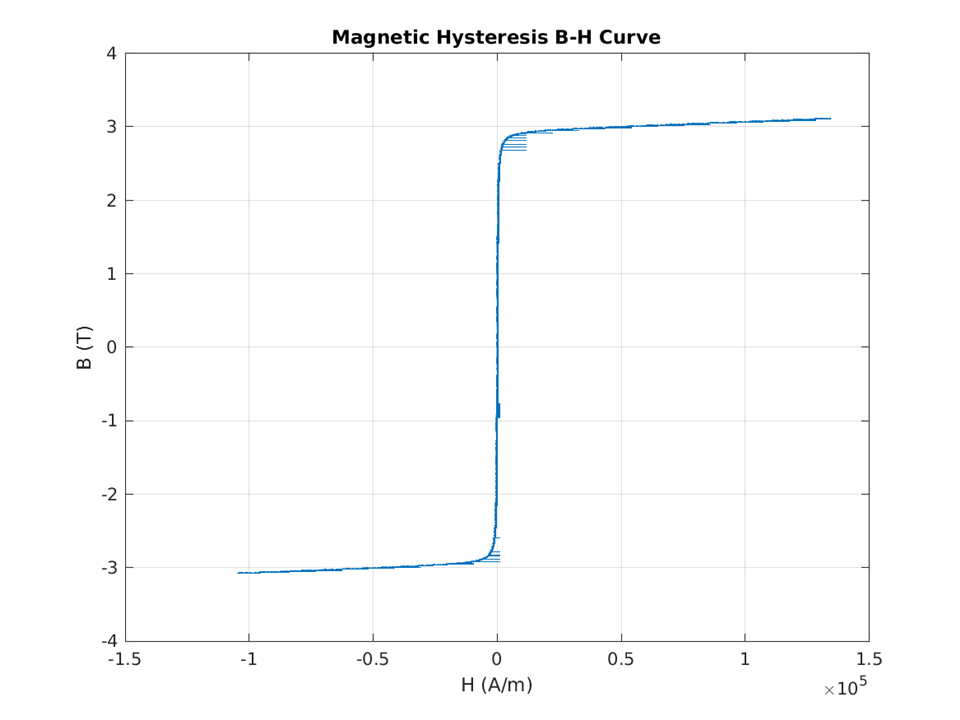
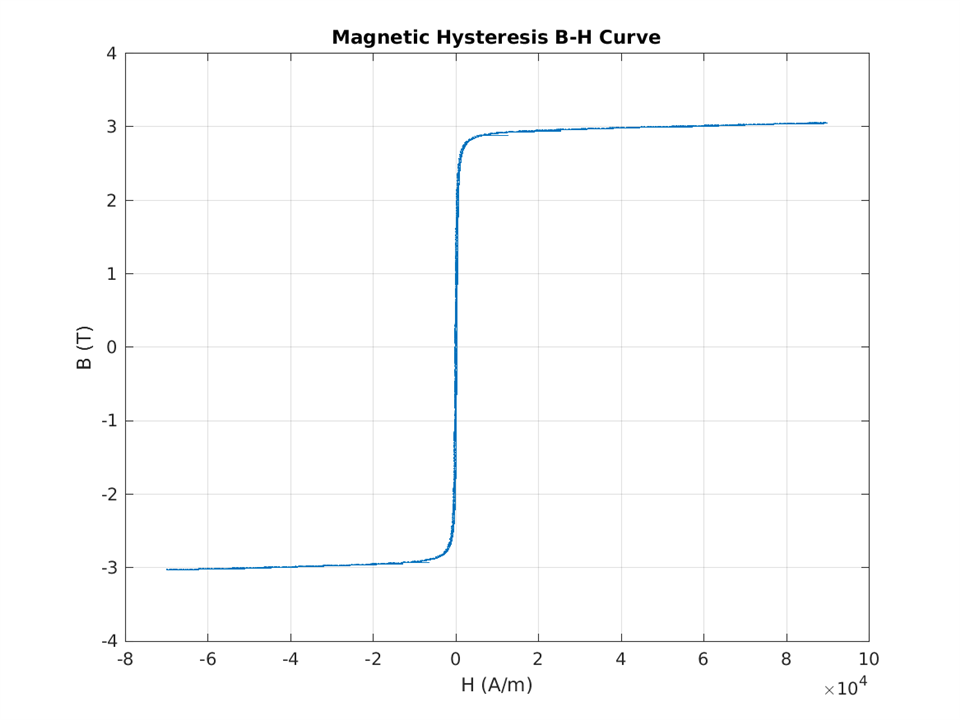
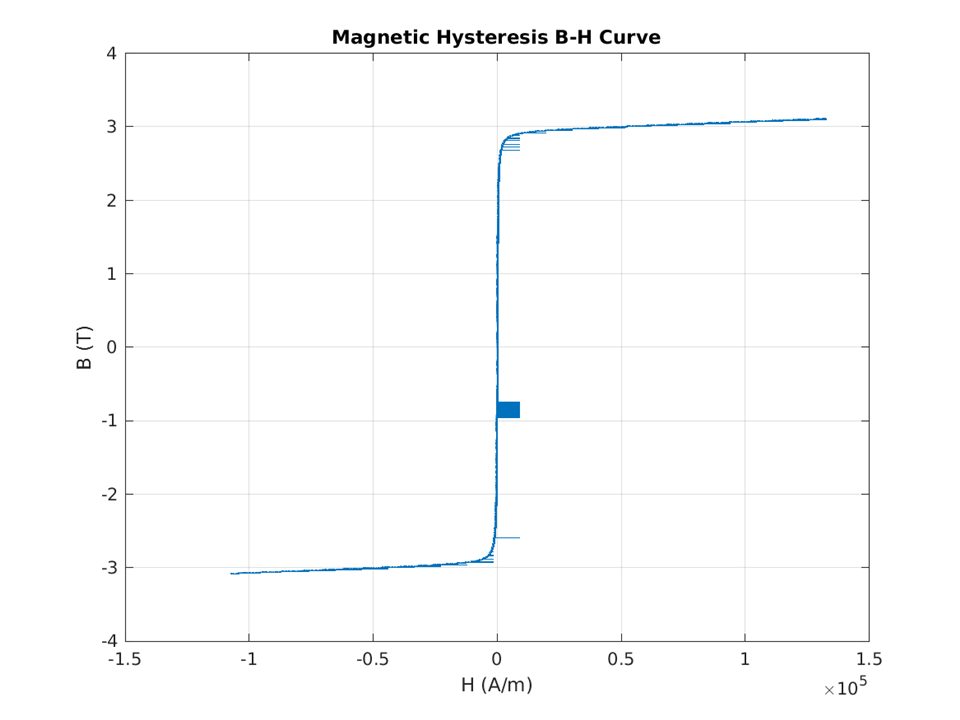
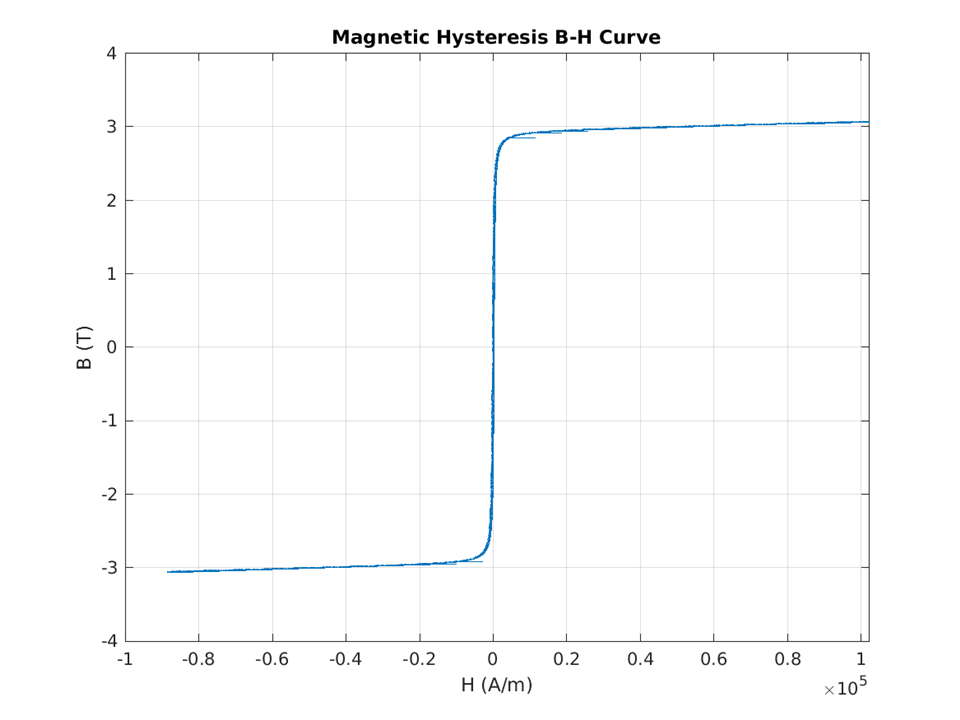
Fig. 12 : shows the typical BH curve after JA perturbation simulation .
Two kinds of hysteresis curves - one without perturbation, i.e., nominal curves, and the other after perturbation are obtained from simulation.
Bnominal and Hnominal are the hysteresis curves without any perturbations.
The data files for these can be found on Mendeley data or IEEE Dataport, and are not plotted.
All the other 36 curves obtained after perturbations can be found with data, code and graph of the curves .
11. Conclusion
I had presented different comparative results.
The proposed loss functions also form hyberbolic secant relation with the original loss function,
For even 0.2 voltage difference, the trajectories for the other core or even with the same core at different switching frequencies or variation in the load at the same wattage, the dynamics is unpredictable and do not follow linear relation, and do not increase or decrease at the same rate.
The proposition that the loss functions can be derived in this manner is valid and proved to be in line with non-linear theory, i.e., energy function, Lyapunov stability and even the Lyapunov exponent is found to be in line, with the assumption, proposition and existing theory.
However, this study still lacks generalized loss function, as proposed functions are related to a particular wattage and voltage.
| {gallery}Paper, Part- 2 |
|---|
 |
 |
 |
 |
 |
 |
 |
 |
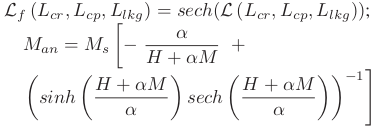3.Paper, Part- 3
In this paper, the comparative analysis of 36 different flyback transformers from 30W to 85W is being presented.These two-winding transformers are operated in continuous/discontinuous mode, switched from MOSFET controlled by microcontroller from 60 kHz to 97 kHz. Eighteen wattage’s which are used internationally at different voltage and current ratings have been analyzed on fifteen different cores - EEL22, EE25, EEL25,EF25, EE28, EER28, EER28L, EE35, RM8/I, ETD29/16/10,ETD34/17/11, E25/13/7, PR30x19, ATQ27/18, RM12/I.
1. operating mode
All the transformers ranging from 30W to 85W are operated in continuous mode.
30W has been designed with core EE25 in ‘C’ at 20V & 15V.
- Lp (15) ≈ 0.76 Lp(20),
- Llkg (15) ≈0.76 Llkg (20)
When 30W is designed with EEL22 & RM8/I,
- Lp (15) ≈ 0.81 Lp (20),
- Llkg (15) ≈ 0.81 Llkg (20).
Six Transformers of 45W have been designed with EEL25 at Vo = 19V,20V, 14.85V, in continuous
mode.
For T1 :
- Lp (14.85) < Lp (20) < Lp (19) ;
- Llkg (20) ≈ Llkg (14.85) < Llkg (19).
For T2 :
- Lp 19 <Lp (14.85) < Lp (20),
- Llkg (19) < Llkg (14.85) < Llkg(20) .
This shows, even if the same core is used in the similar m = C, the variations are not linear.
Four 65W are designed for Vo = 19V & 19.5V but with four diff. cores.
For T1 :
- Lp (19.5) ≈0.72 Lp (19),
- Llkg (19.5) ≈ 0.72 Llkg (19).
For T2 :
- Lp (19.5) ≈ 0.87 Lp (19),
- Llkg (19.5) ≈ 0.87 Llkg (19).
Thus, for ∆ = 0.5V , the comparisons can be seen.
Besides above comparisons, the peak currents Ip in continuous mode flyback transformers are lower than discontinuous mode. For m = C,the ratio Krp < 1, which makes primary inductance higher than discontinuous mode as Krp > 1. The upper limt of Lp in continuous mode is approximately four times that discontinuous mode,that is
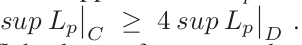
This is same as part-2
2. JA & loss function
The loss functions are different for the same wattage even at slightly different voltage level, e.g. 65 W (19V & 19.5V).
And the residual errors are in femto 10−15, or zepto 10−21. scale.
Rest all results are similar in conclusion as in part- 2.
One being the proposed loss functions also form hyberbolic secant relation with the original loss function, and the second one, for even 0.5 voltage difference, the trajectories for the other core or even with the same core at different switching frequencies or variation in the load at the same wattage, the dynamics is unpredictable and do not follow linear relation, and do not increase or decrease at the same rate.
The reflected output voltages also do not correlated in the same way as in the Part-II of this study.
| {gallery}Paper, Part- 3 |
|---|
 |
 |
 |
 |
 |
 |
 |
|
4. CodeOcean
The plots were generated using MATLAB on CodeOcean in 2022.As during that time, in the plots, I hadn't appended the legend on the plot about transformer wattage, except the plot was saved with transformer wattage; it would be difficult to go through gallery but on the reference link, one can see the code and the plot name.
4.1 Transformer 1
| {gallery}Transformer 1 |
|---|
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
4.2 Transformer 2
| {gallery}Transformer 2 |
|---|
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
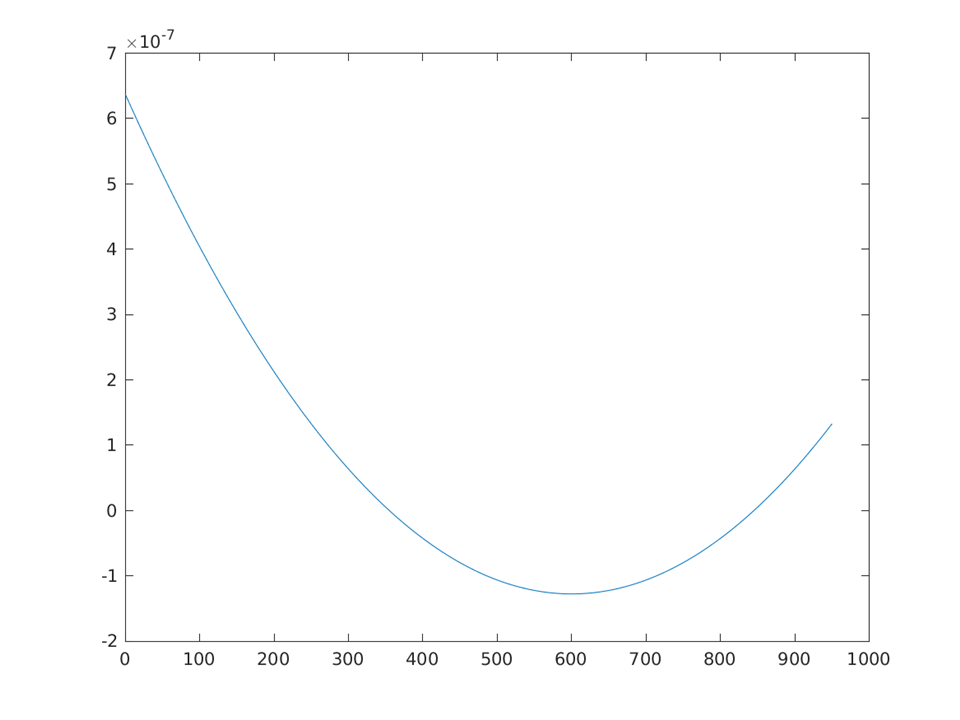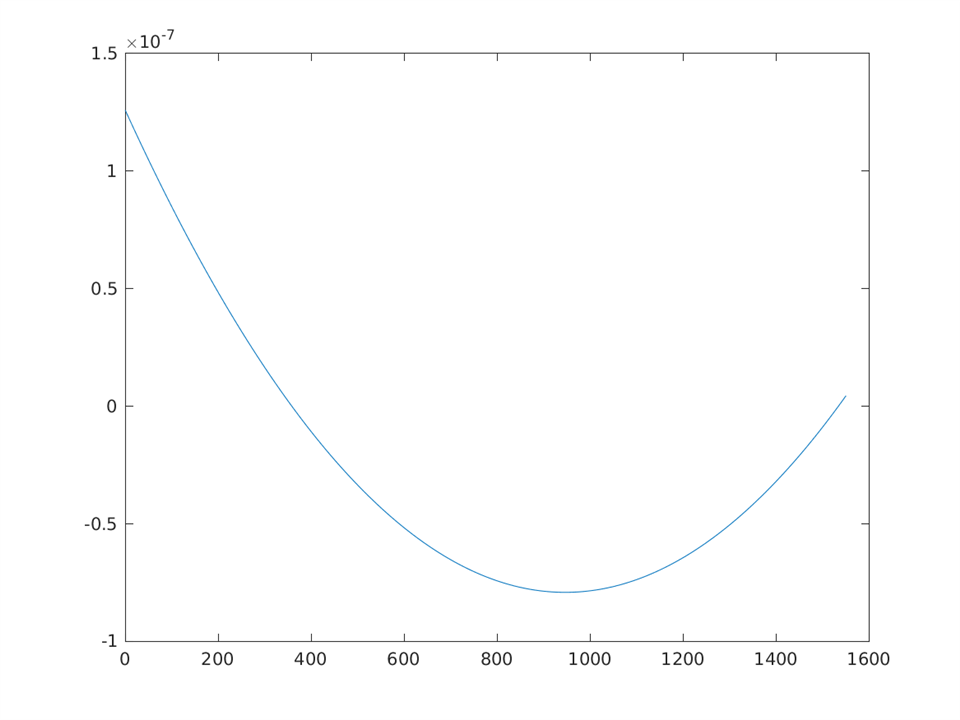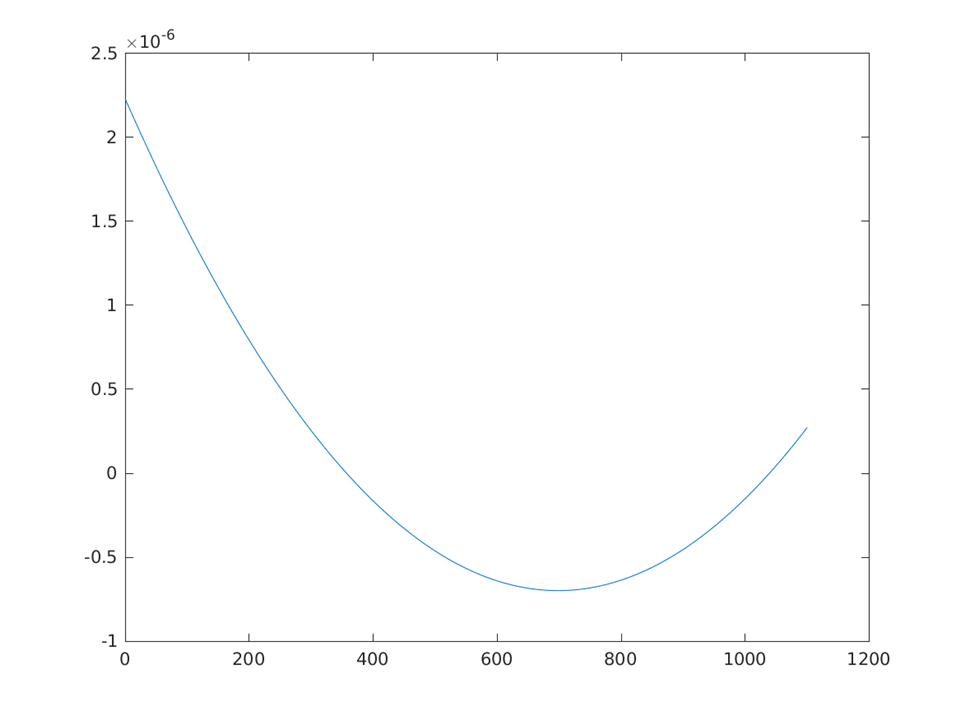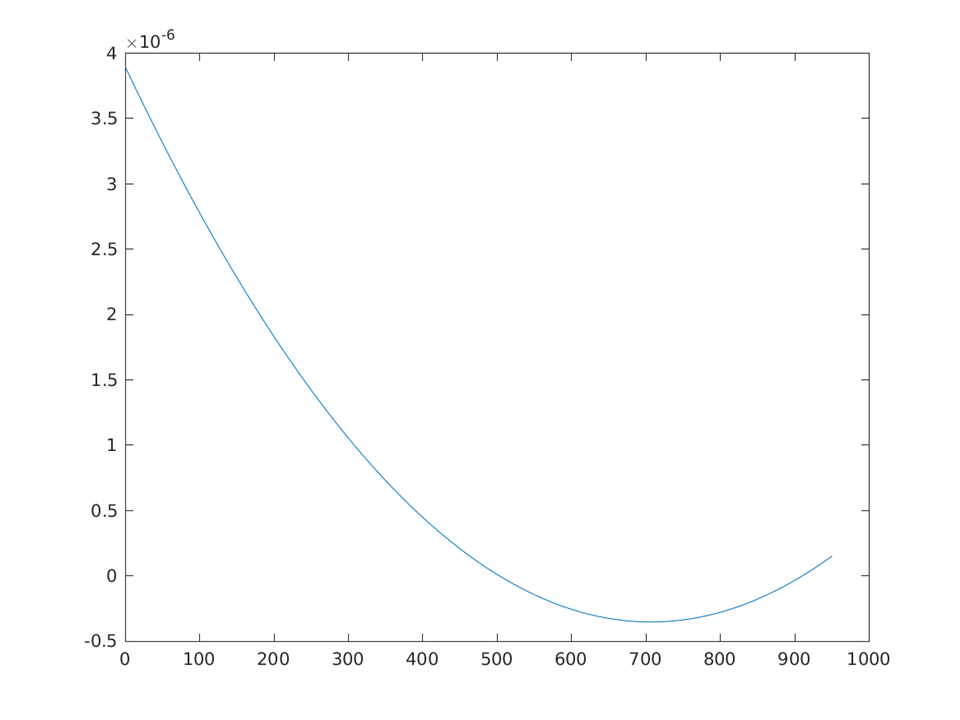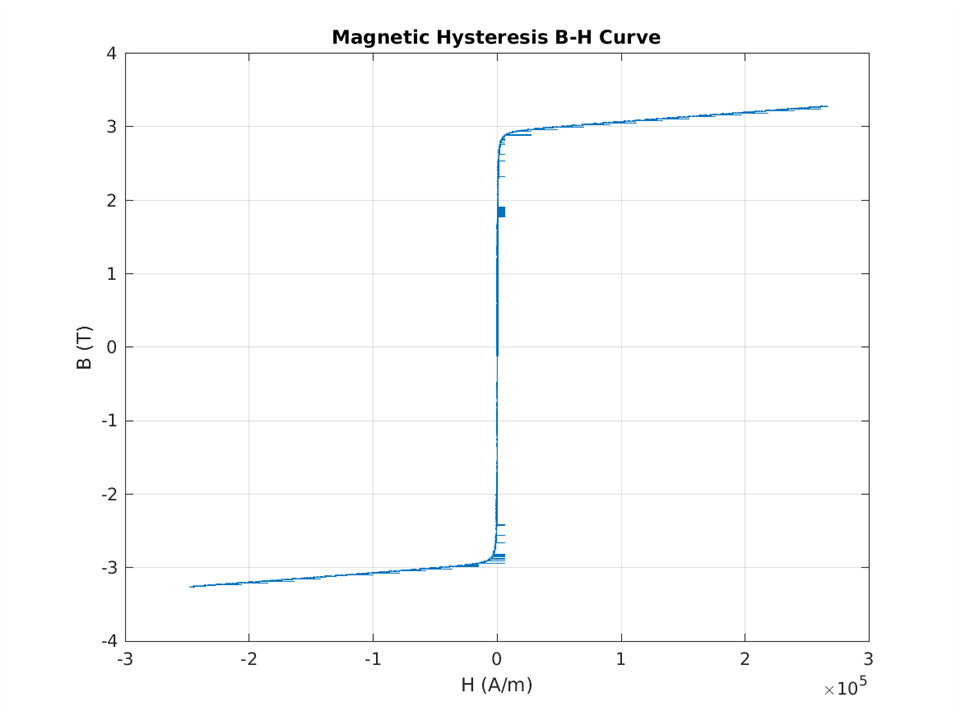
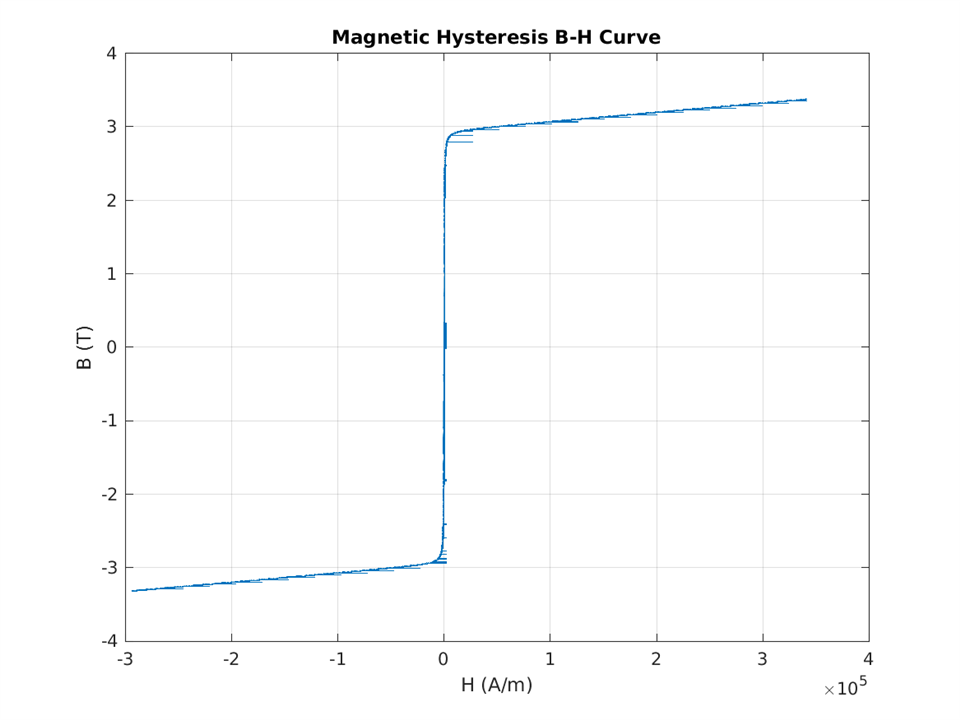
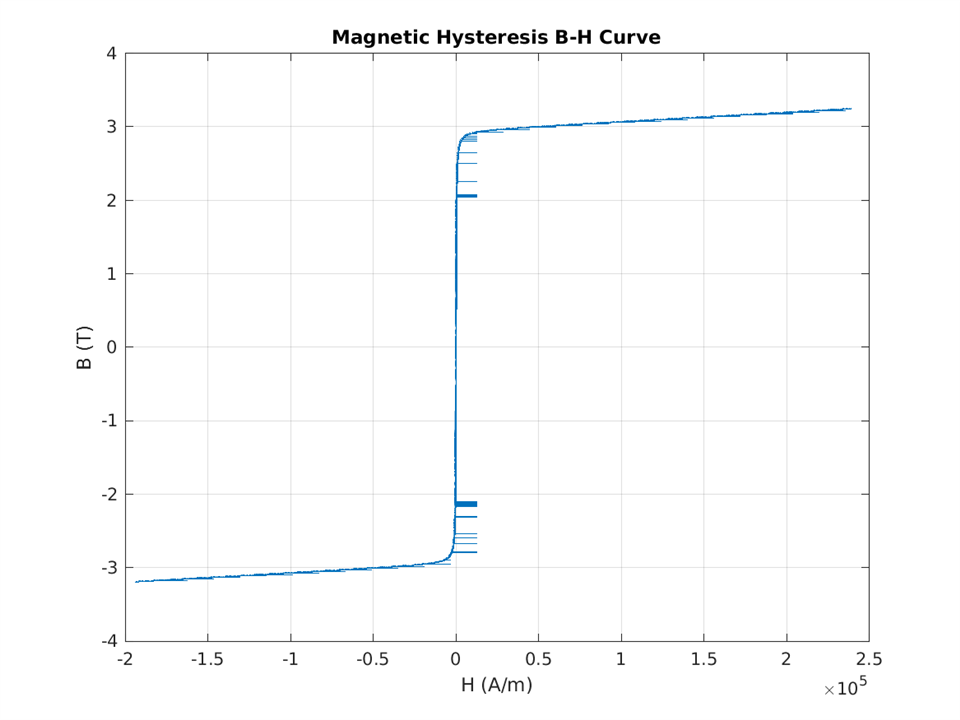
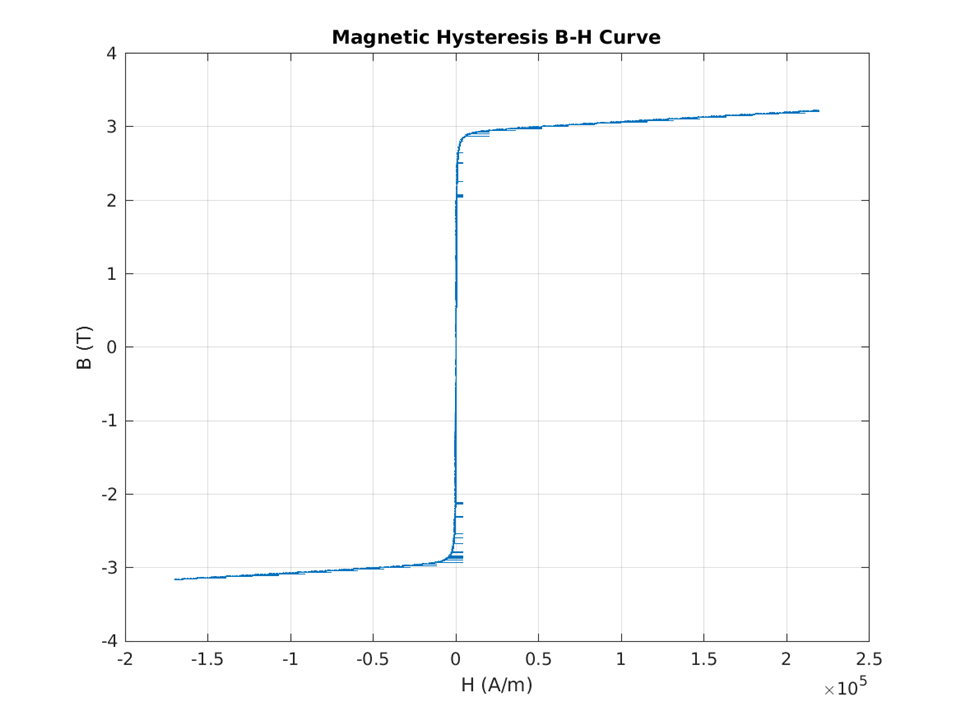
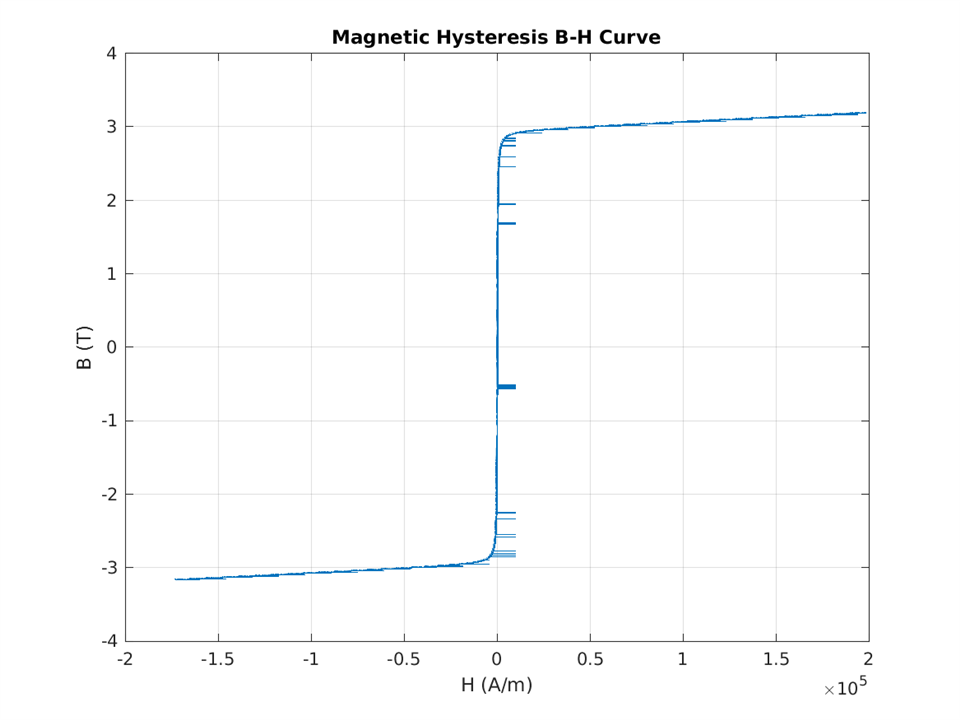
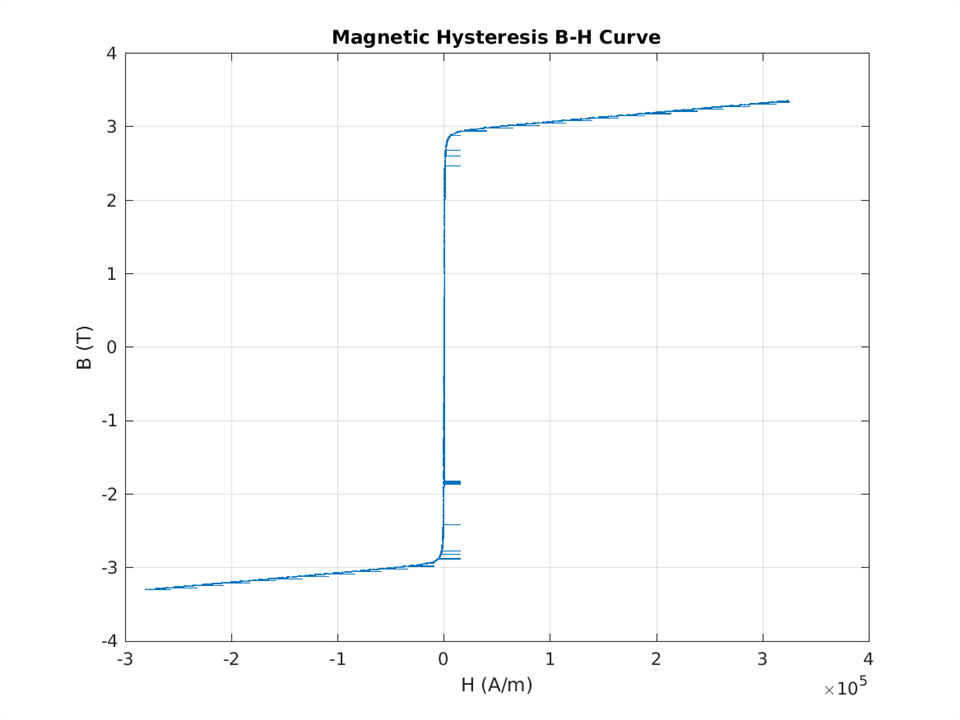
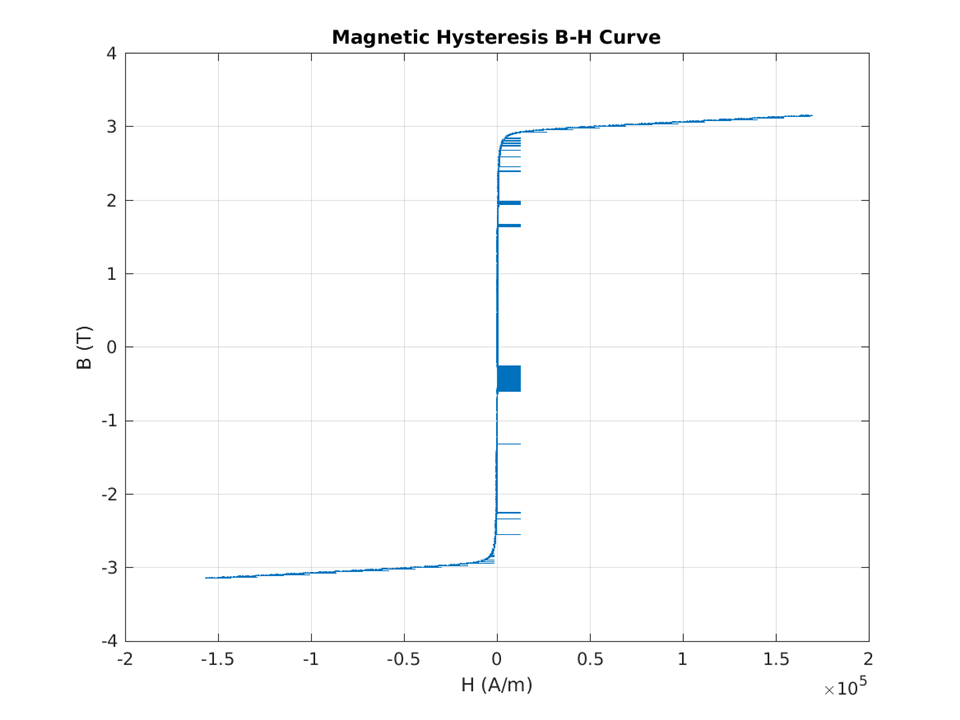
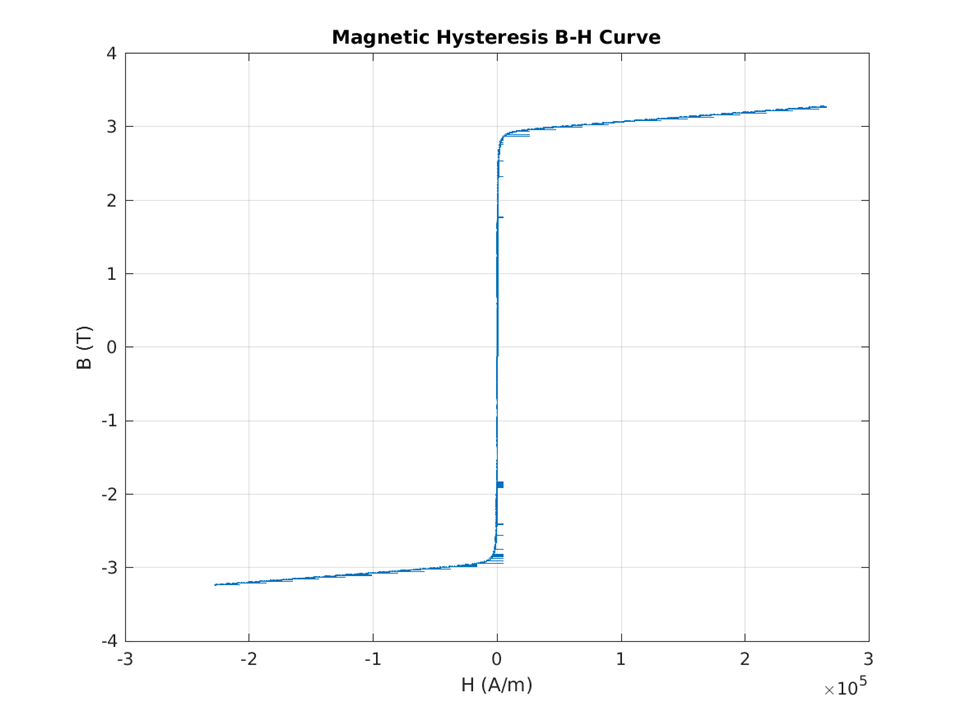
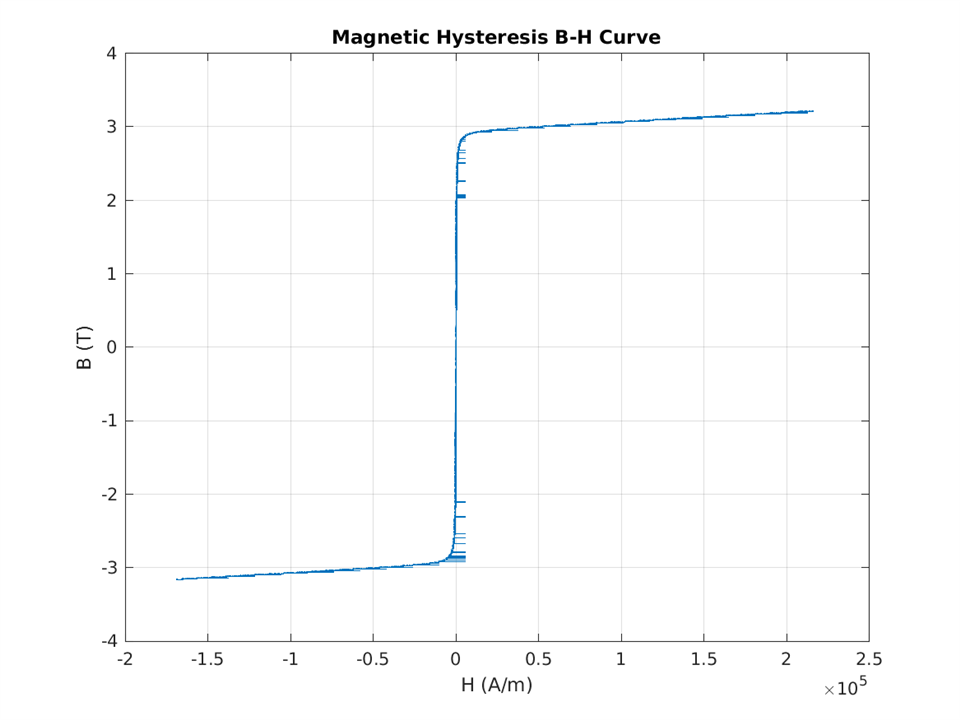
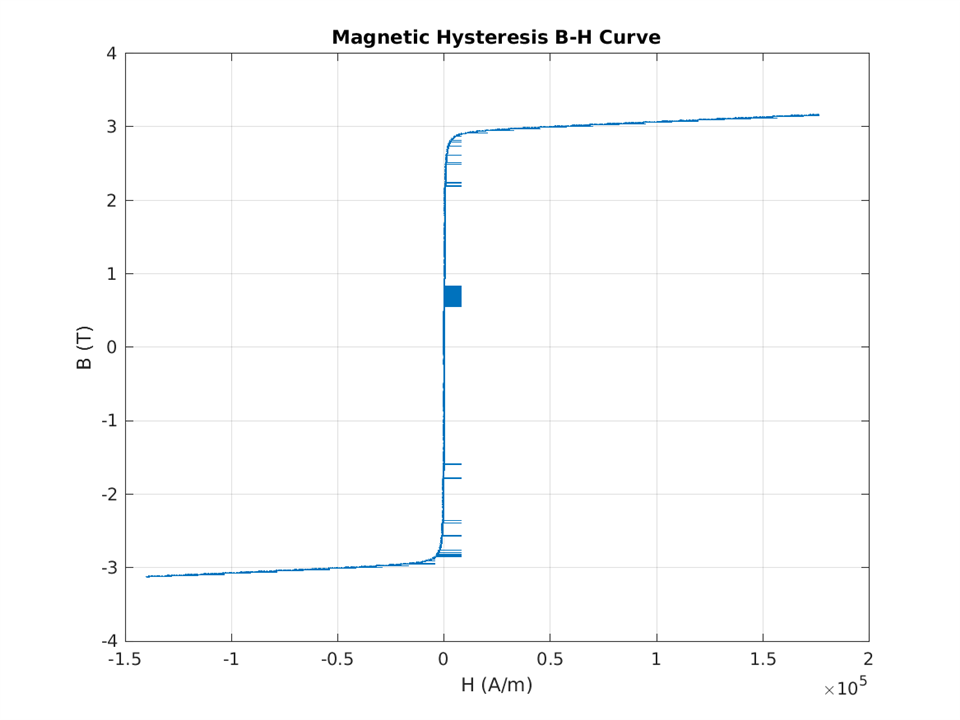
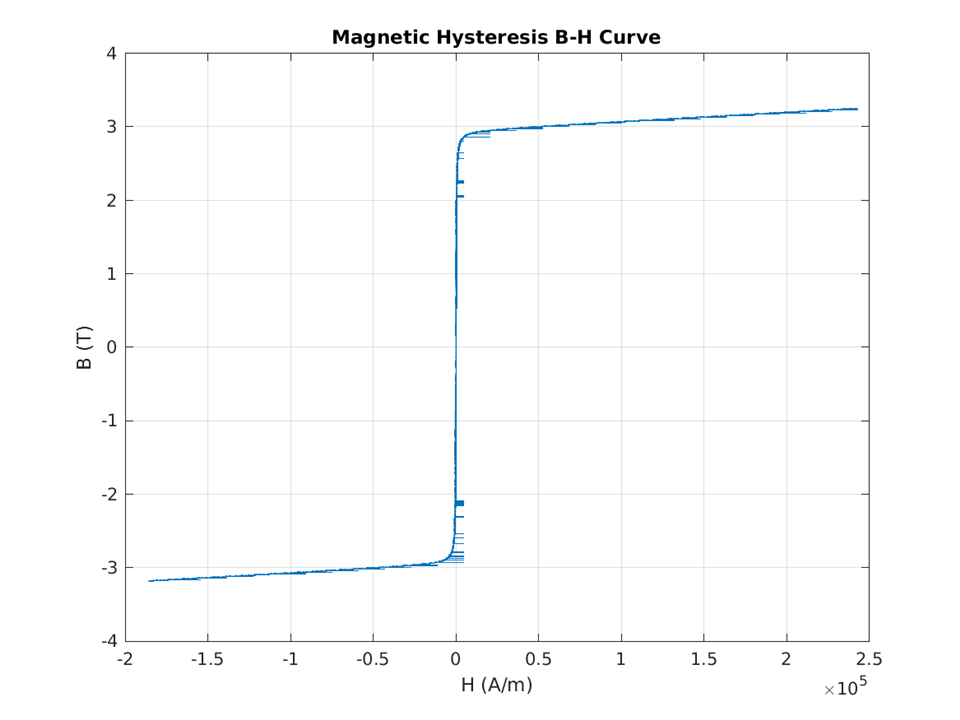
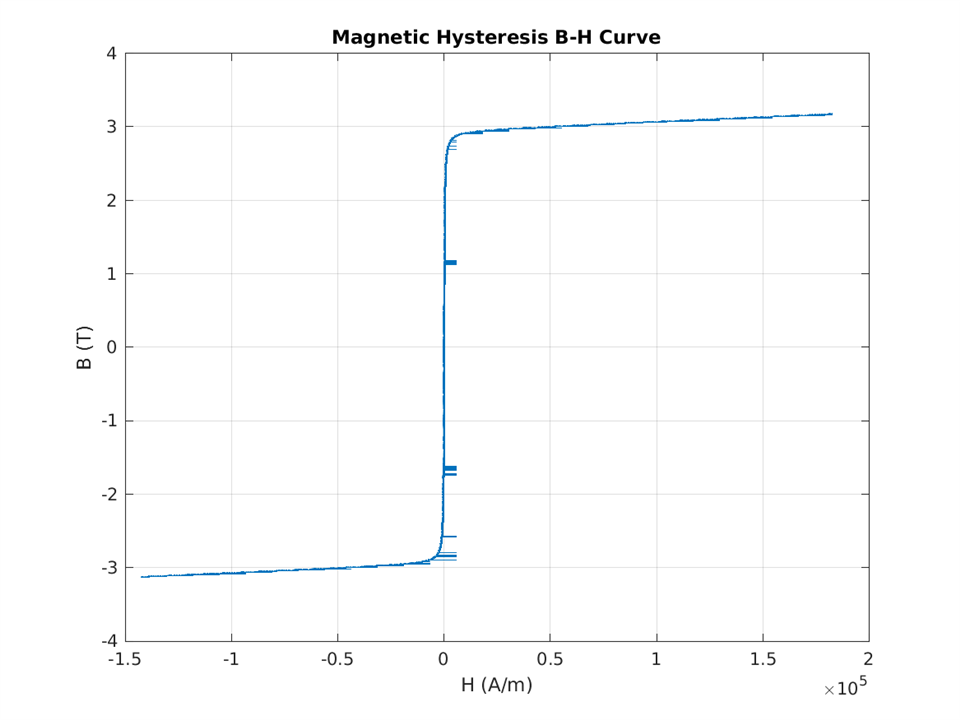
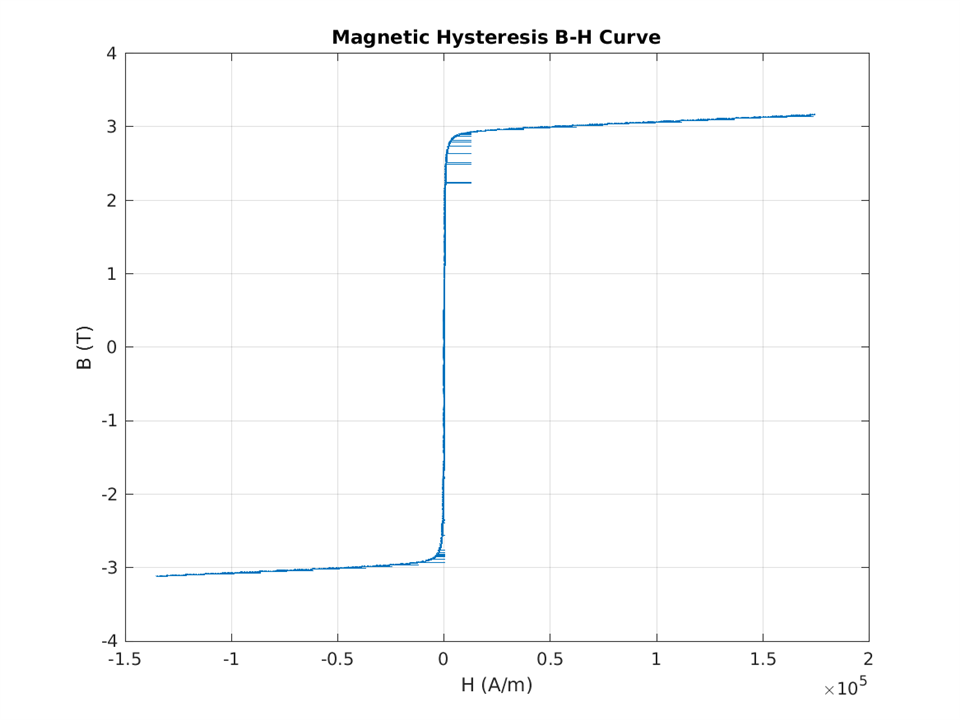
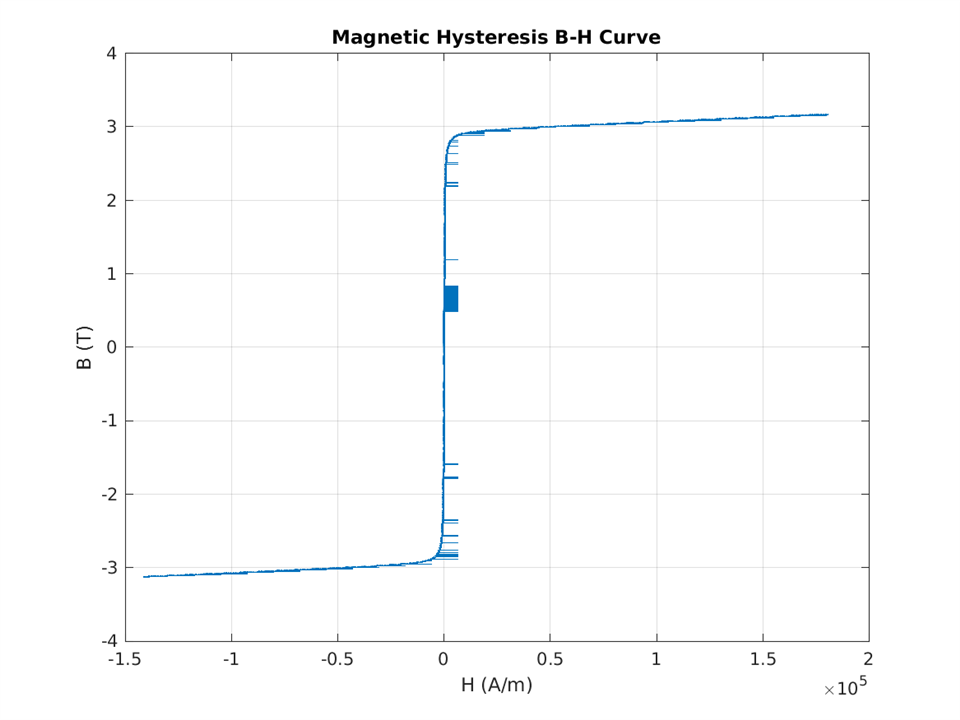
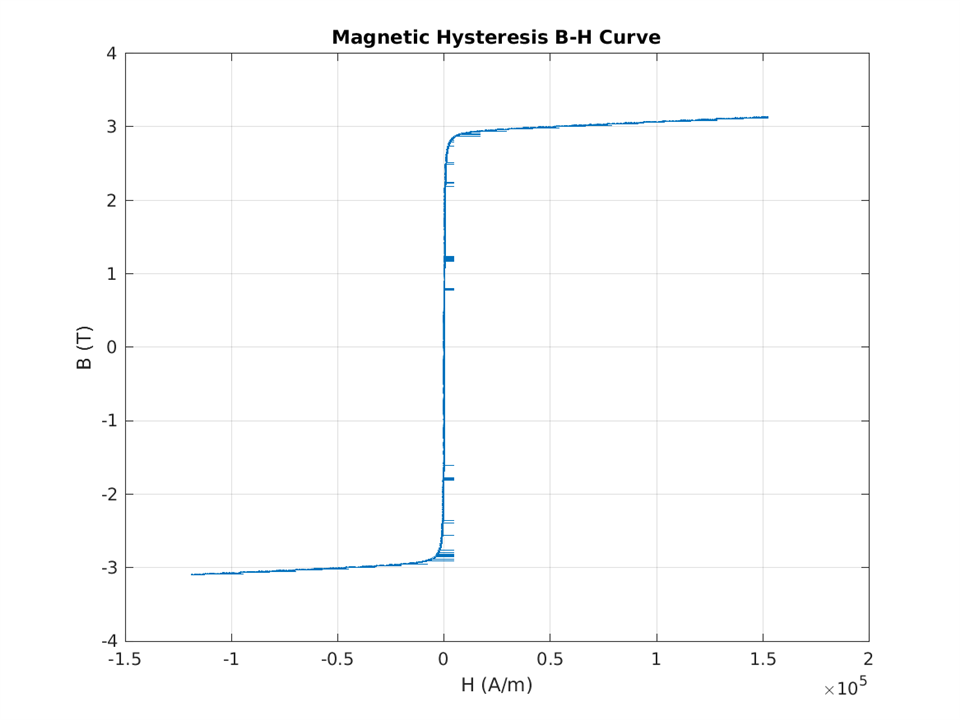
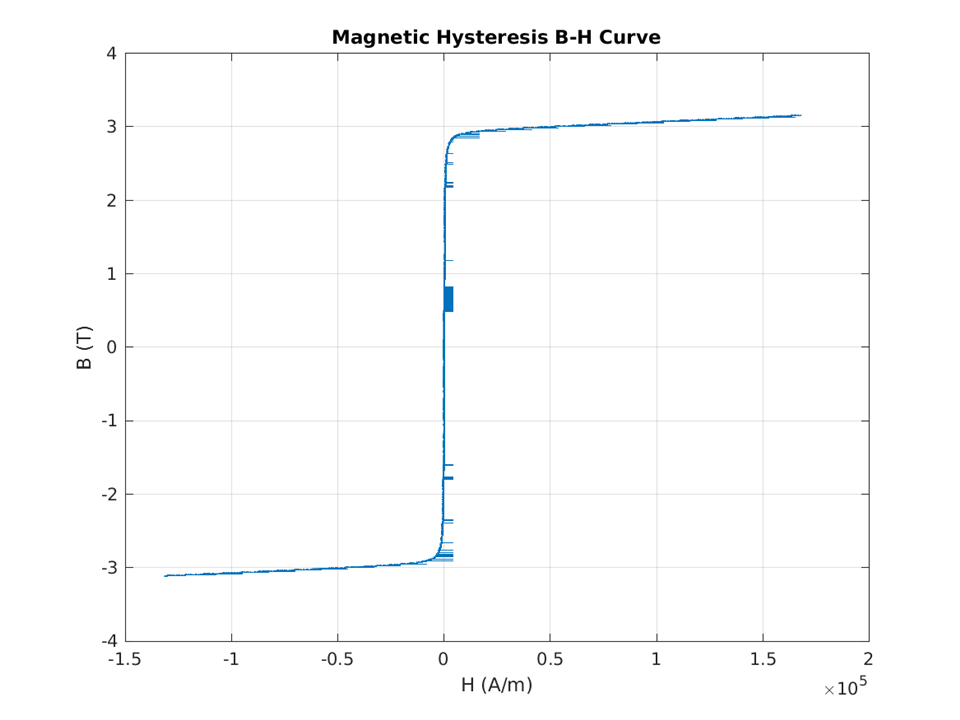
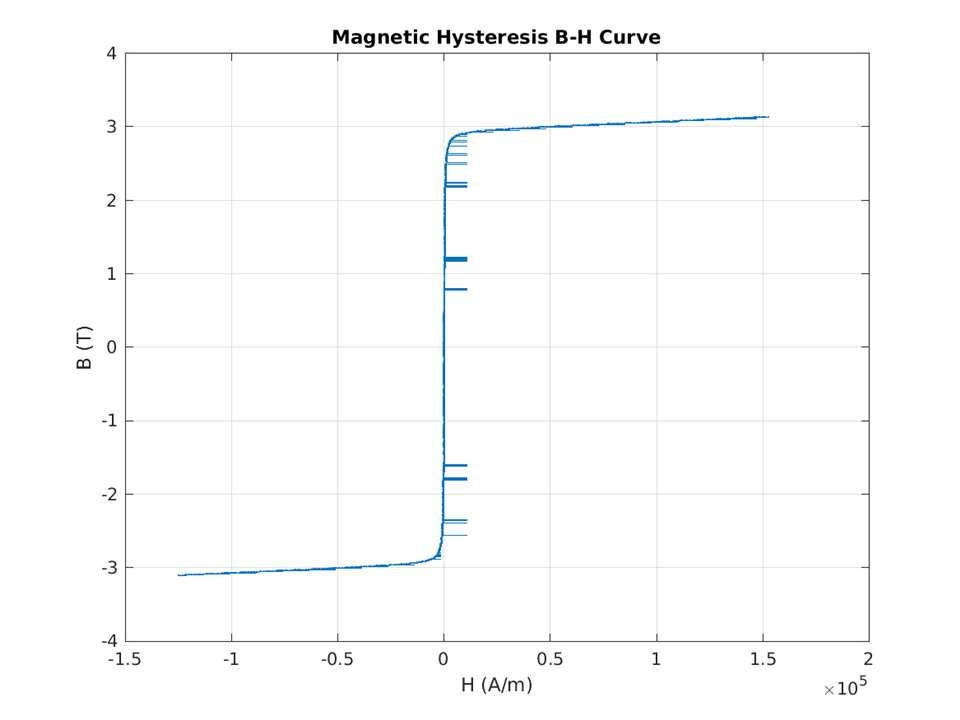
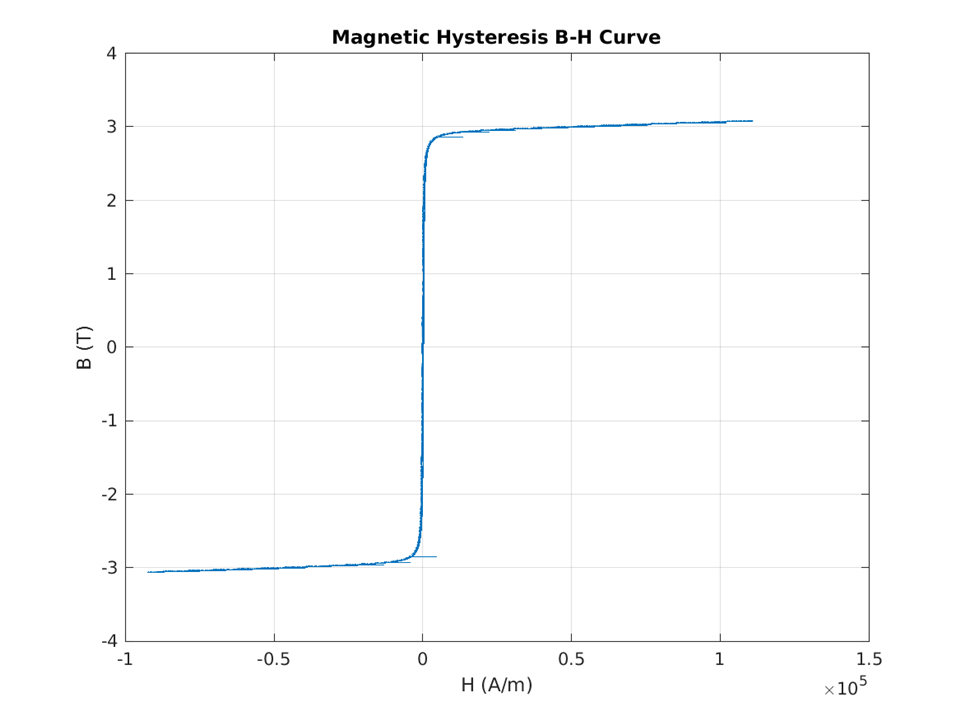
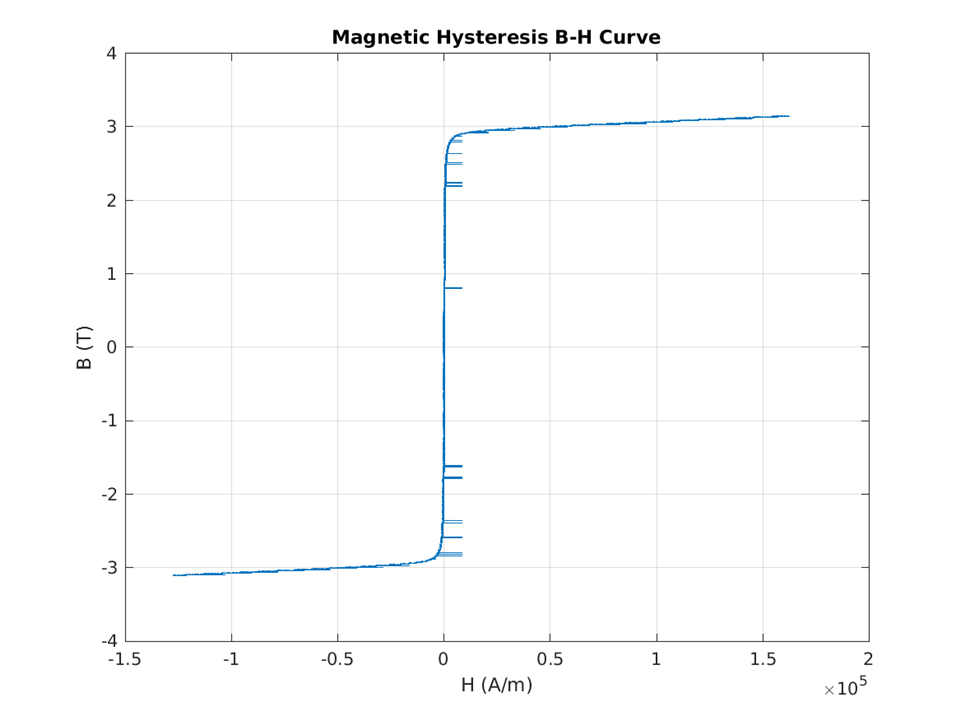
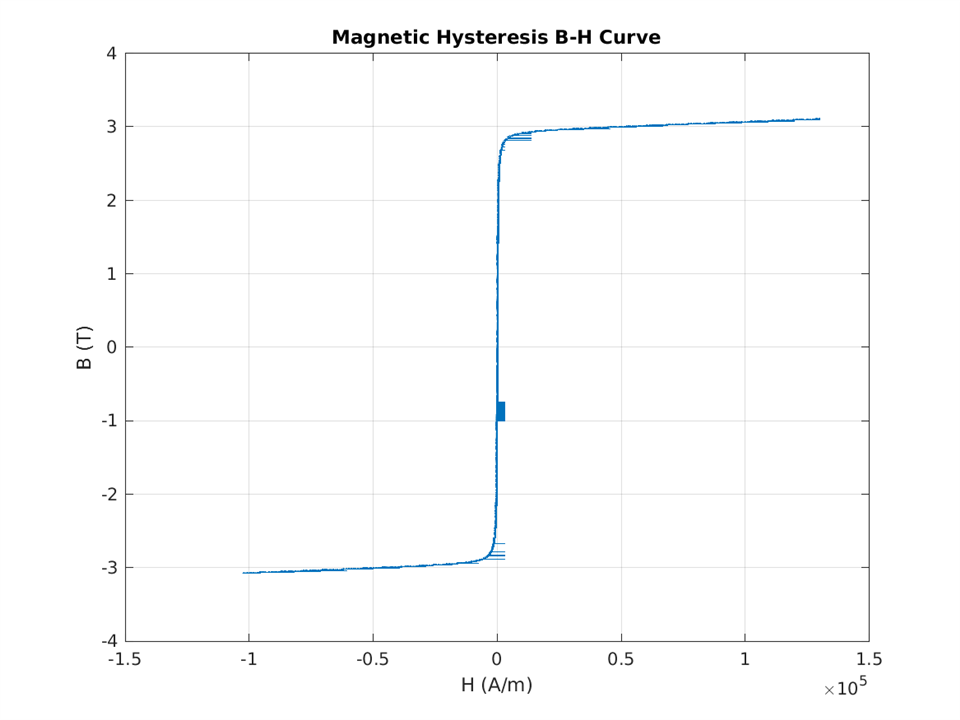
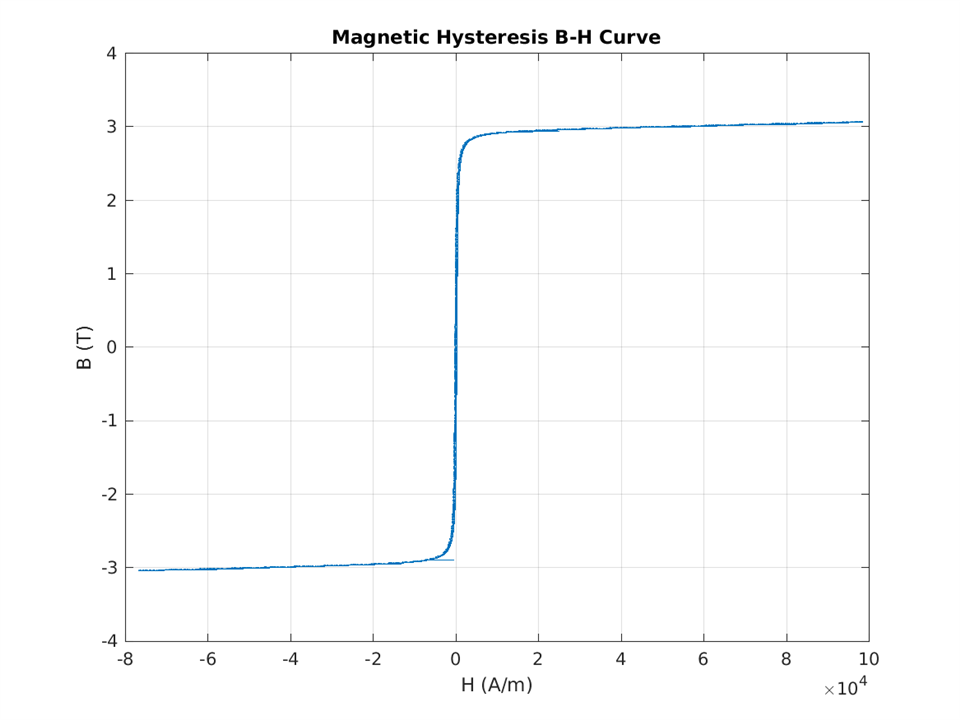
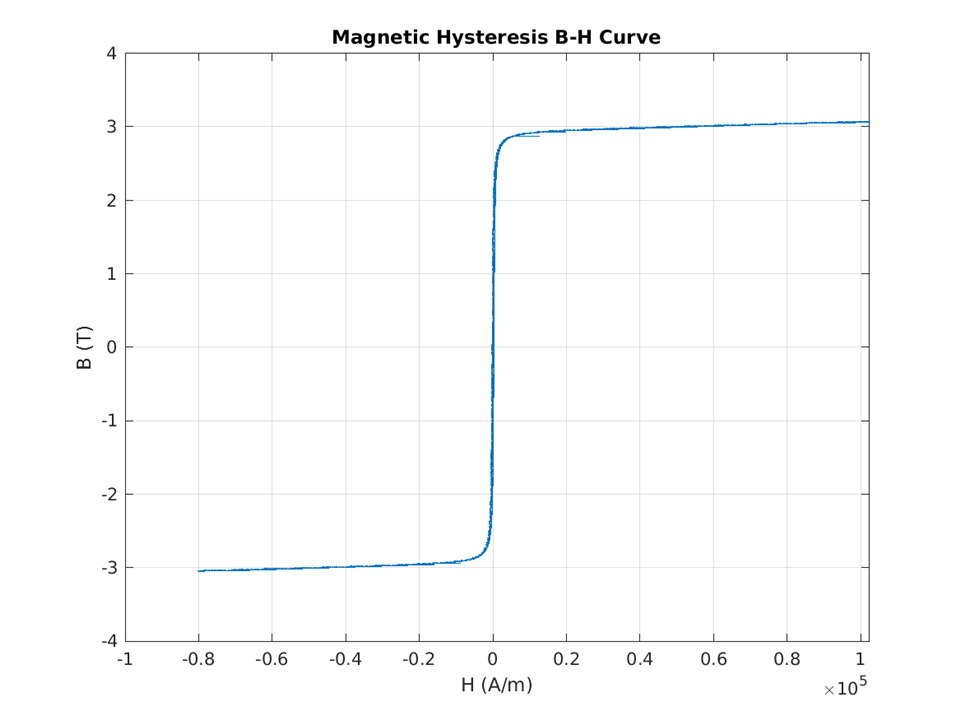
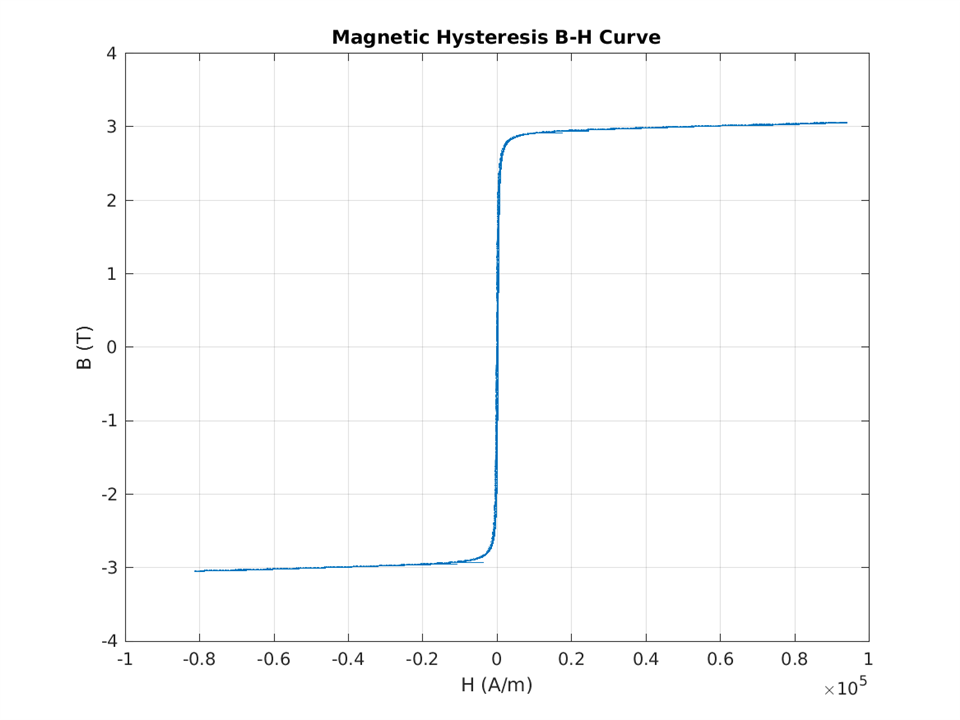
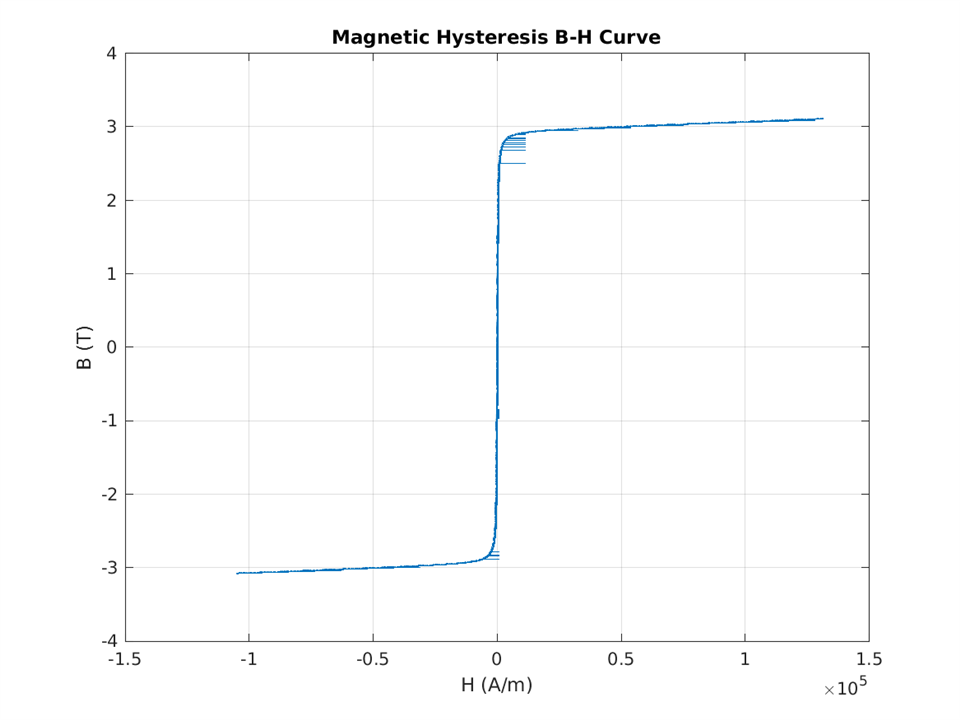
4.3 BH Curves of 36 FBTs based on Transformer 1
| {gallery}BH Curves of 36 FBTs based on Transformer 1 |
|---|
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
|
 |
 |
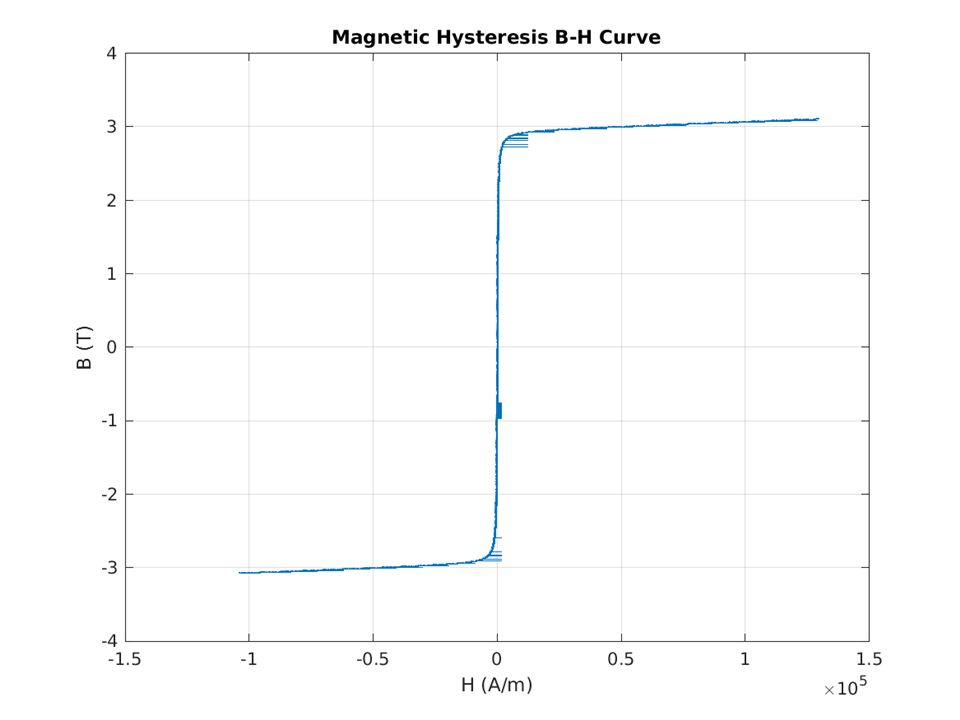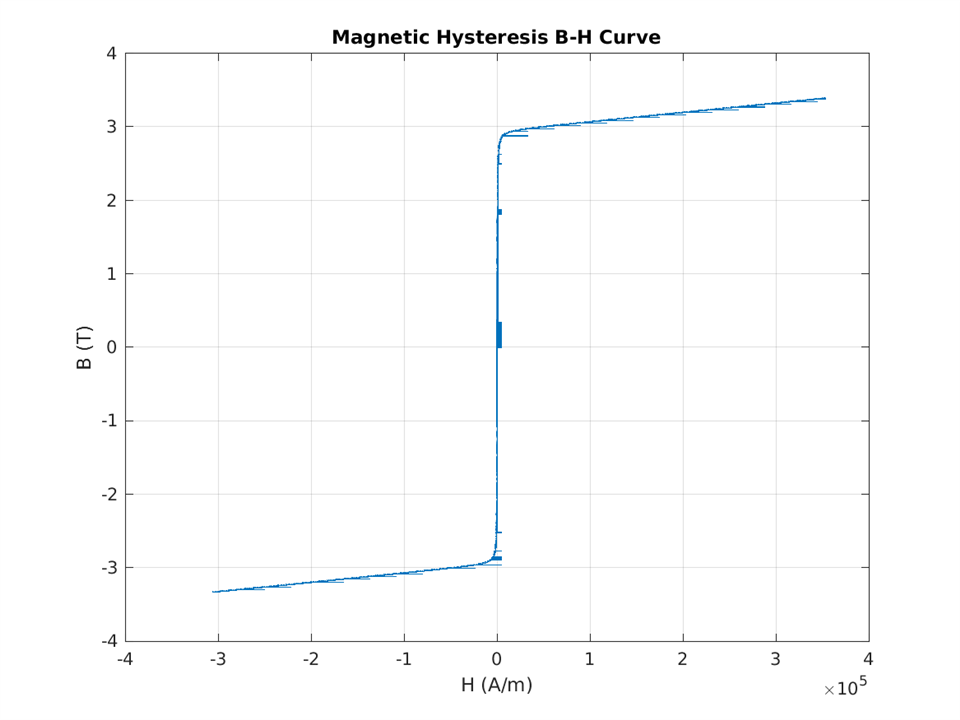
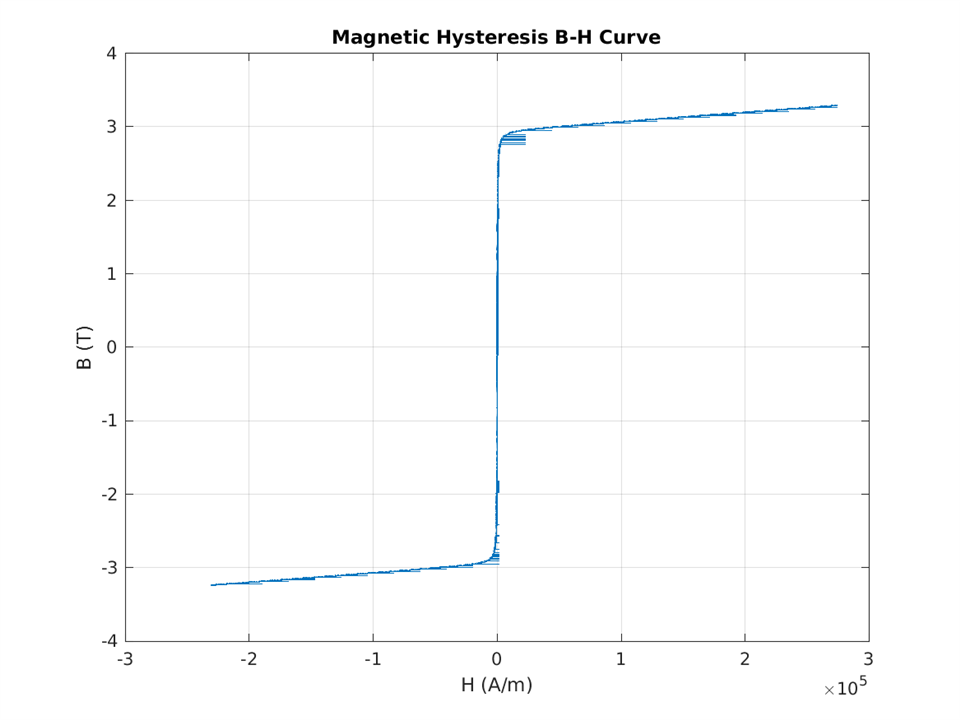
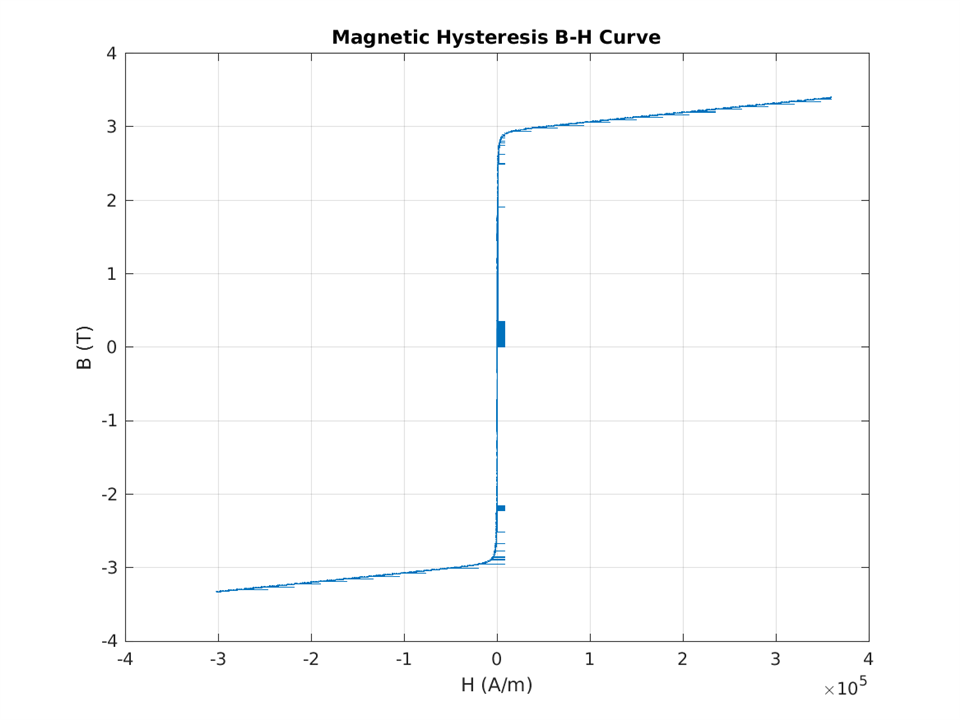
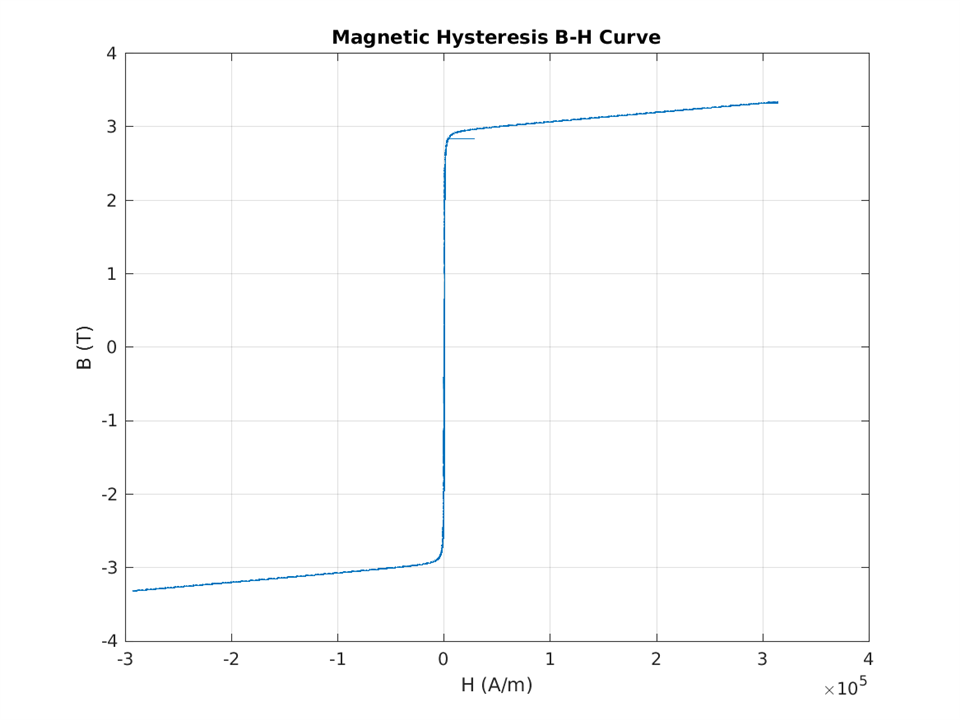
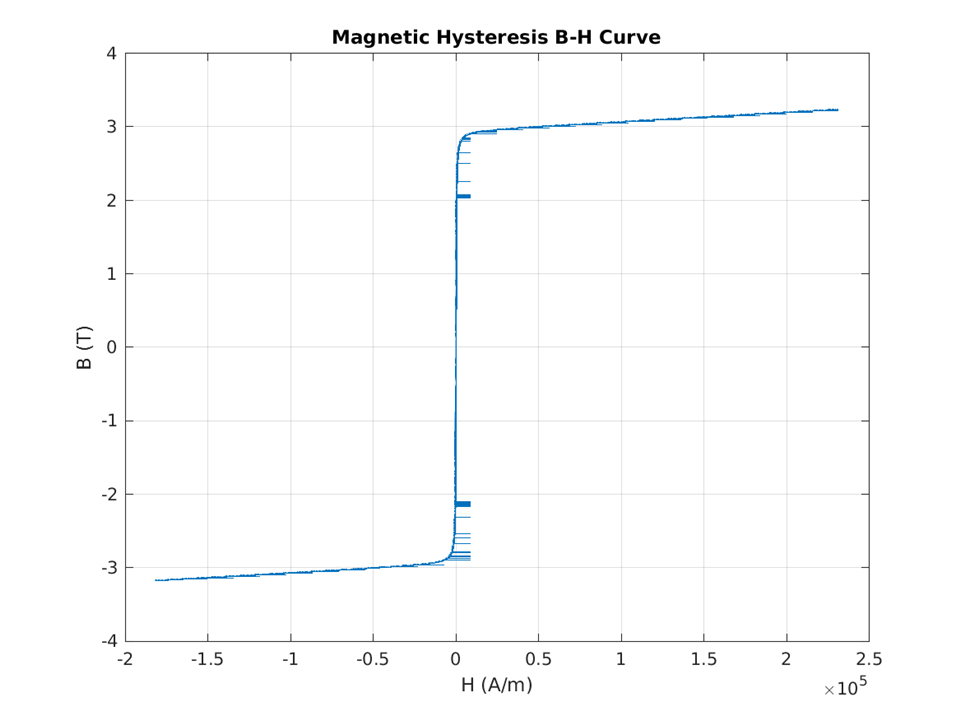
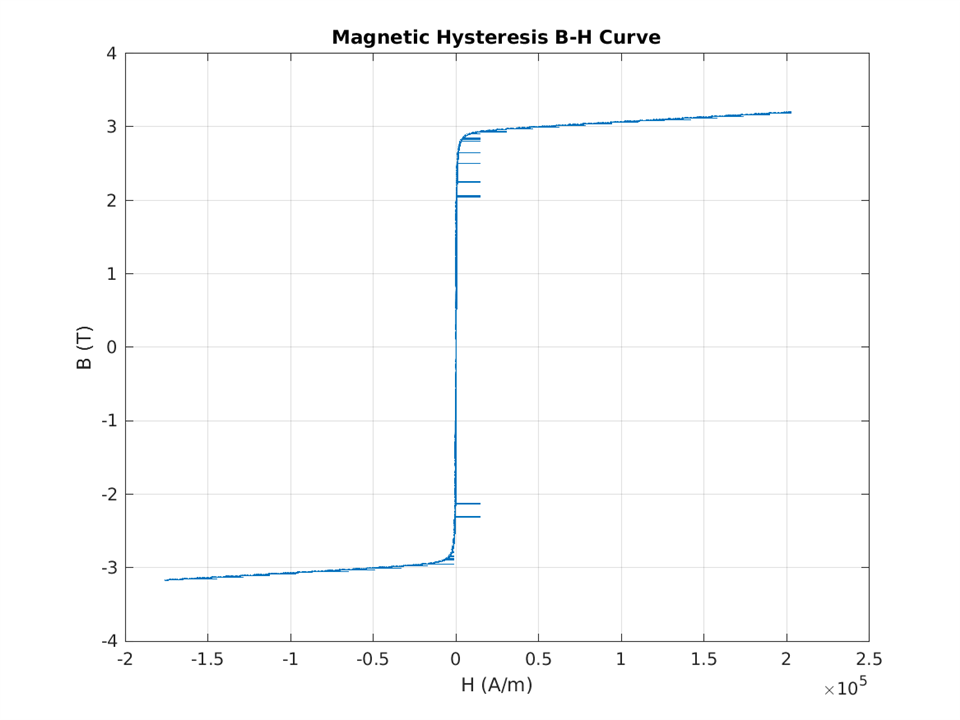
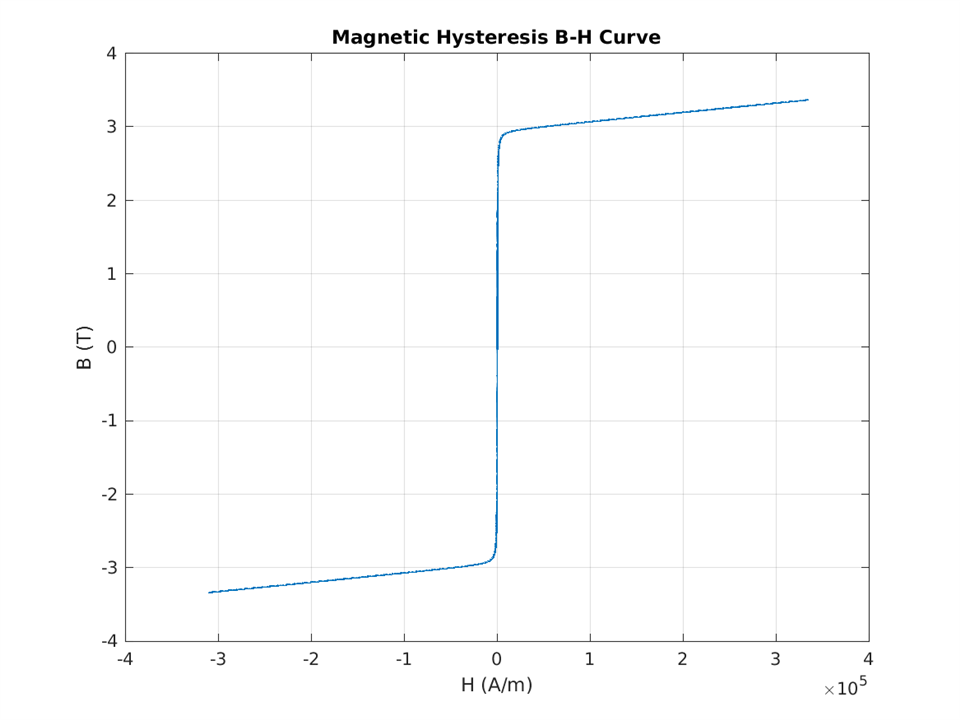
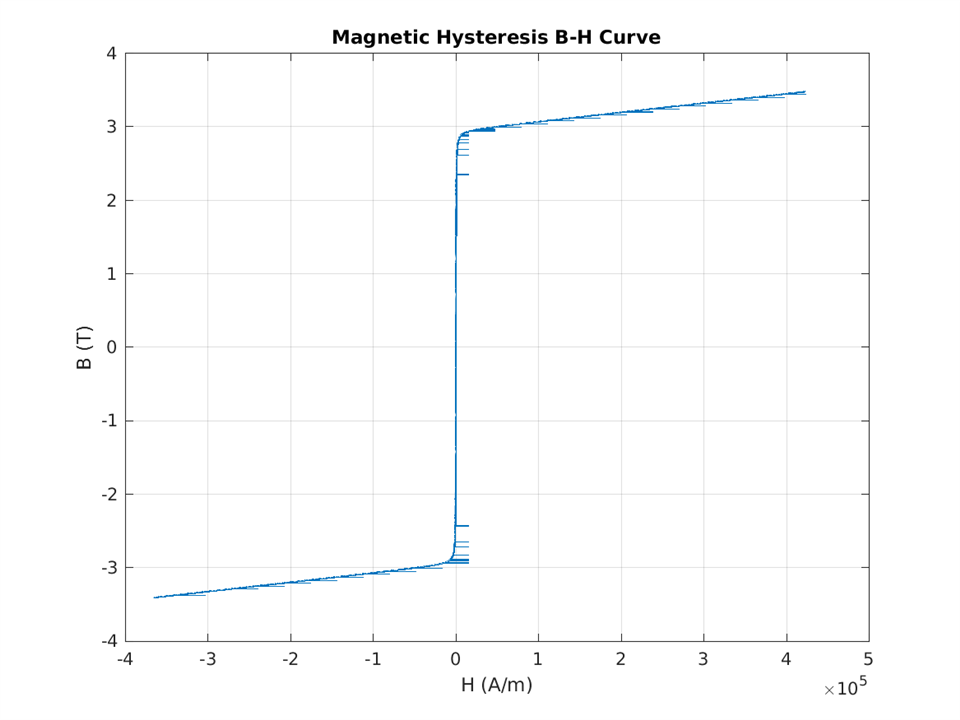
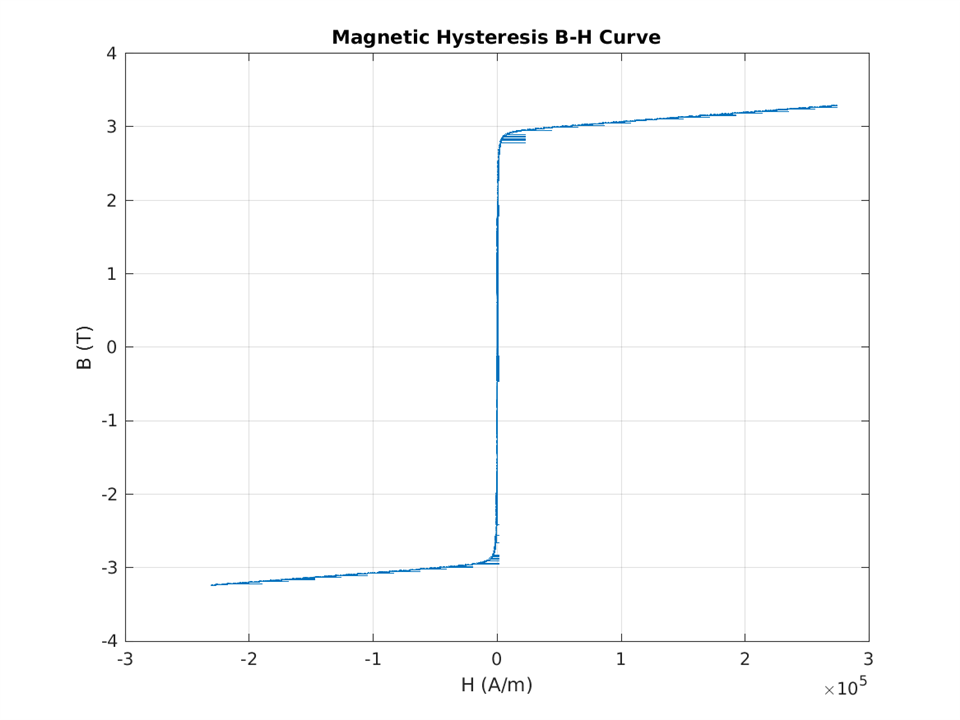
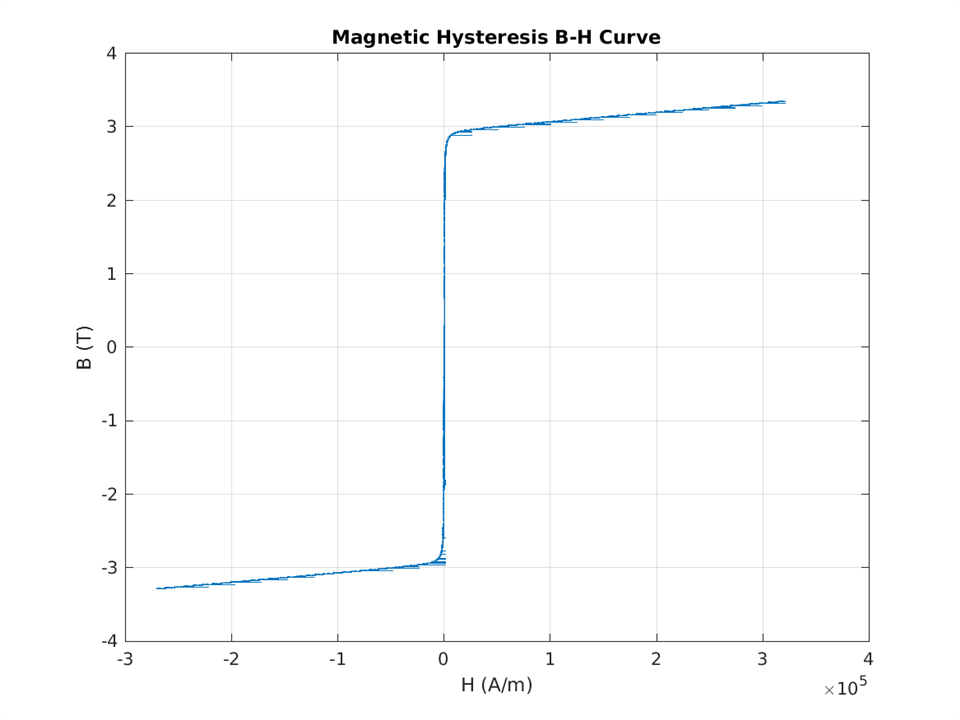
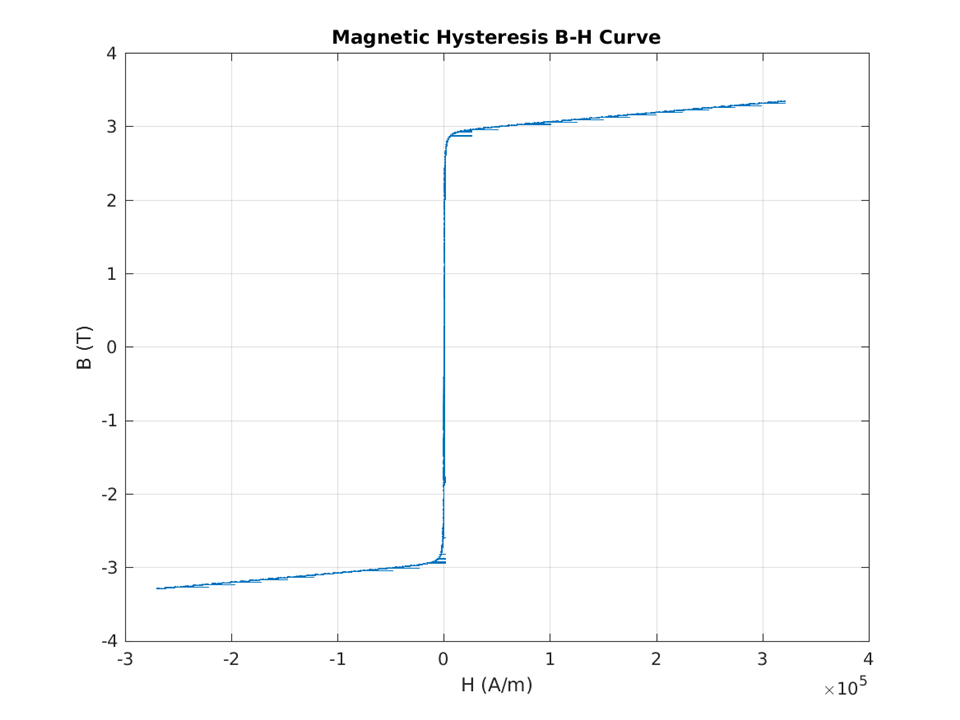
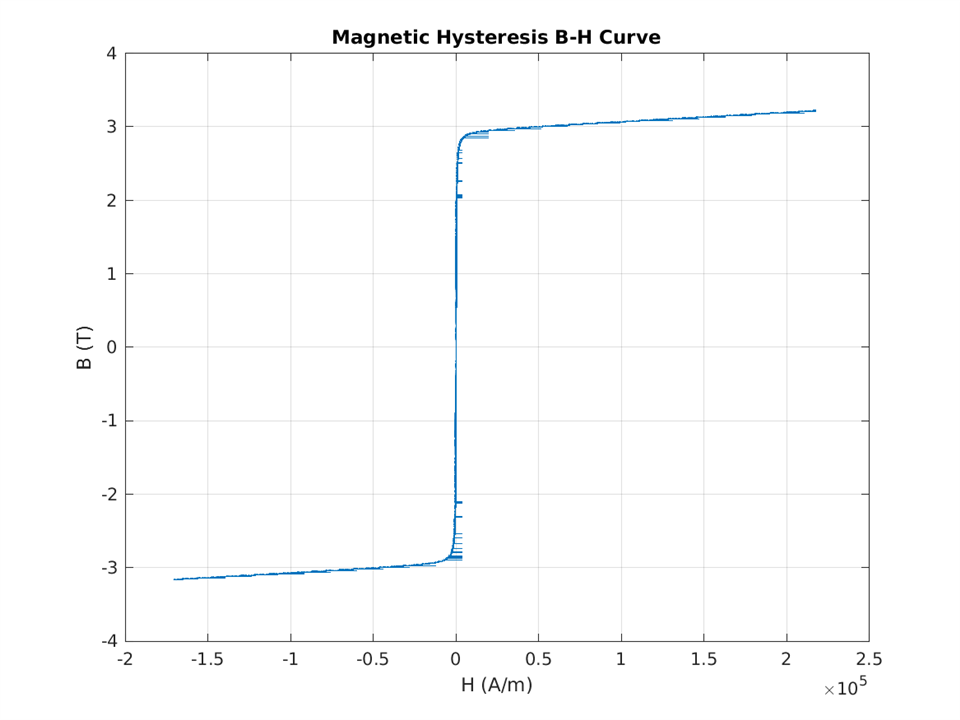
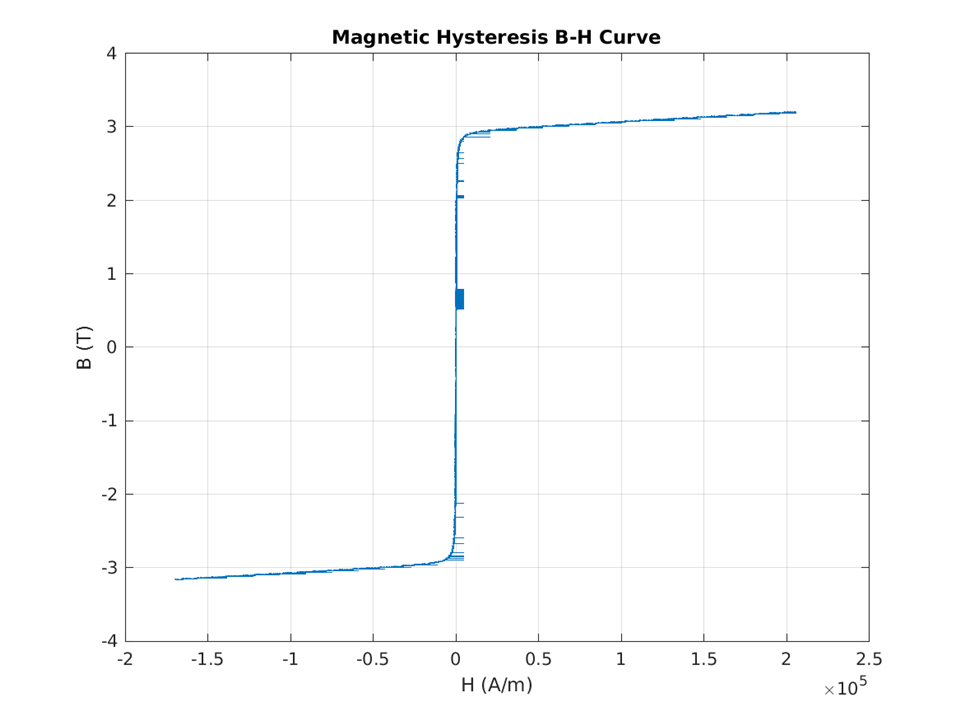
this shows desipte different perturbations, all 72 curves, displays the typical ferrite BH curve, the cores used in designing.
On seeing all 72 curves , it can also noticed that core saturation arms are not exactly parallel to H-axis of the magnetizing current, rather both the arms have many micro zig-zag alternating small perturbations along the arm-line, making small angle with H-axis., i.e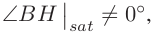 
, proving core is not saturated.
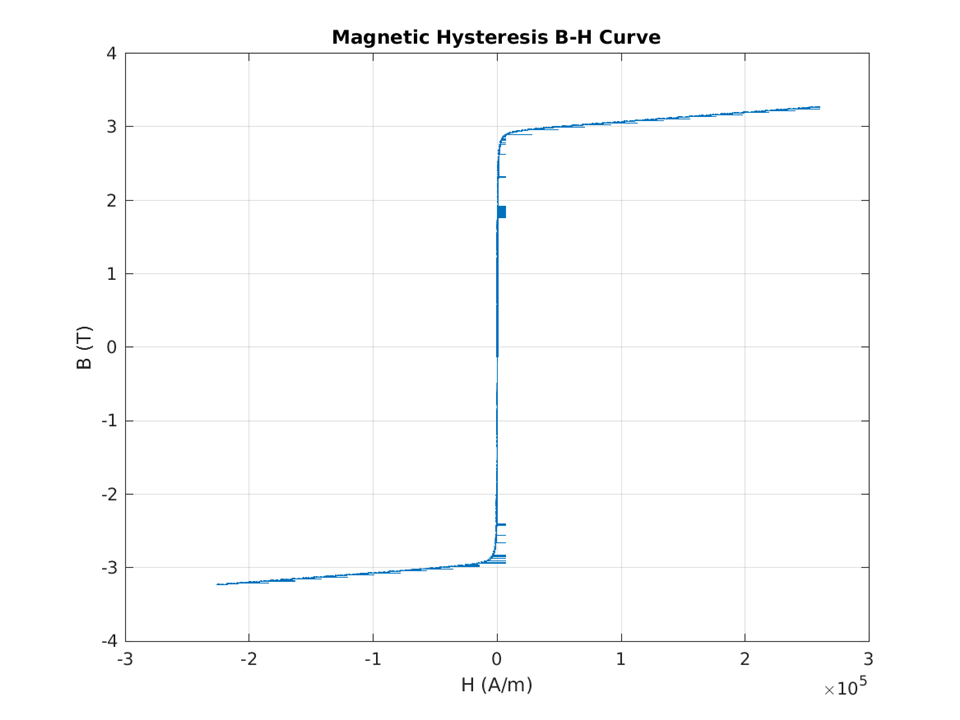
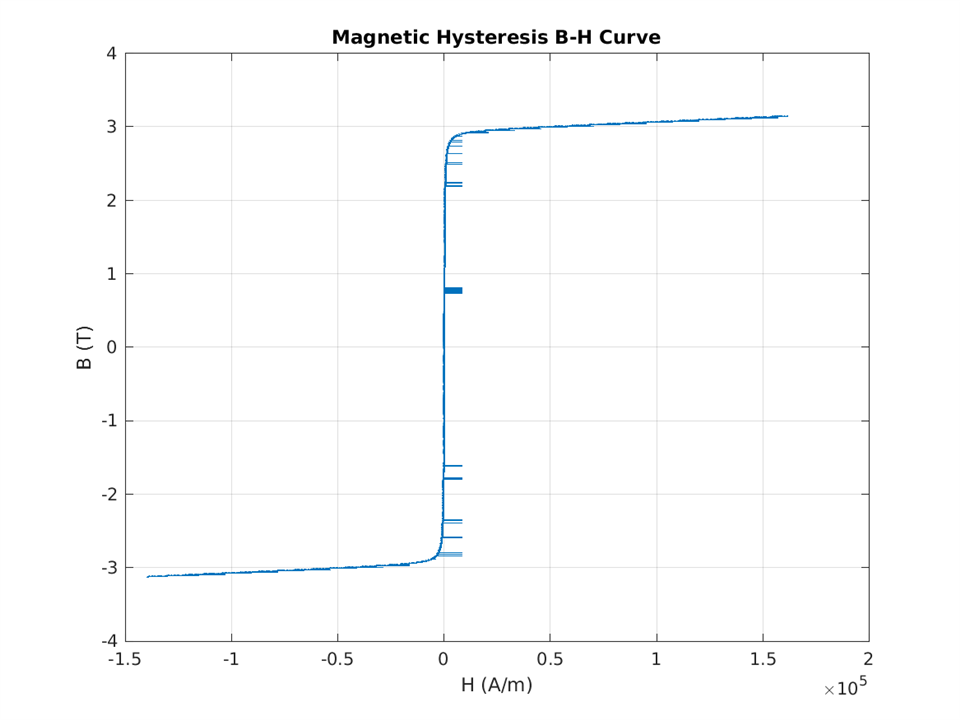
4.4 BH Curves of 36 FBTs based on Transformer 2
| {gallery}BH Curves of 36 FBTs based on Transformer 2 |
|---|
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
|
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
|
 |
 |
 |
 |
 |
 |
 |
 |
4.5 Comparative Results of 72 FBT Transformers
These plots have legends.
| {gallery}Comparative Results of 72 FBT Transformers |
|---|
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
|
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
References
1) Mendeley Data - Title : Data: 72 Magnetic Experiments-Flyback Transformer
2) IEEE CodeOcean - Title : Comparison of 72 Magnetic Experiments-Flyback Transformer
- DOI: https://doi.org/10.24433/CO.4569257.v1
- DOI: https://doi.org/10.24433/CO.4569257.v2
- DOI: https://doi.org/10.24433/CO.4569257.v3
3) IEEE DataPort - Data: 72 Magnetic Experiments-Flyback Transformer
4) Authorea
- Comparative Analysis of 72 Flyback Transformers on 5τ Non-linear Battery with Loss Functions - Part I
- Comparative Analysis of 72 Flyback Transformers on 5τ Non-linear Battery with Loss Functions - Part II
- Comparative Analysis of 72 Flyback Transformers on 5τ Non-linear Battery with Loss Functions - Part III
III. Future Research Work
I was expecting to complete my final research by this blog but could not complete due to number of reasons which include Vivado failure to generate bitstreams(no crash except 3-5 times), health, etc.
In the future research, I expect that I will either write and/or make Videos on
-
Novel ß-Unified (Non Linear)Theory/Postulates/Propositions,
- Development of Novel ß-ElectroMagnetic Equations,
- New Shortened Method of Self Claimed Novel ß Method on 72 Transformers Saturation
- Novel ß Method in Studying The Dynamics of Flyback Converter
- Novel ß Method For Finite Element Modeling and Analysis
I am willing to sell these incomplete/complete personal papers but only on very high costing. One must be rich and read my profile before emailing to buy 1 or more papers.
Top Comments