Introduction
This blog details the results of the tests carried out with some of the radial inductors supplied as part of the Experimenting with Inductors Challenge to build a delay line for use with the rotor reflectometer.
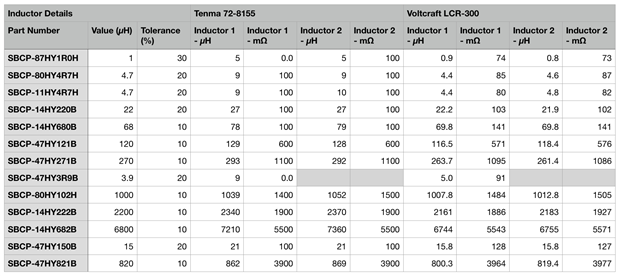
For the initial tests, I measured the inductance and DC resistance of the radial inductors utilising the Tenma 72-815572-8155 LCR meter supplied as part of the challenge and my Voltcraft LCR-300 meter. The table below shows the results obtained.
You can see that the Tenma meter struggled with the lower values of inductance due to its lower resolution, therefore I decided that any calculations carried out would use the values obtained from the Voltcraft LCR-300.
Individual inductor tests
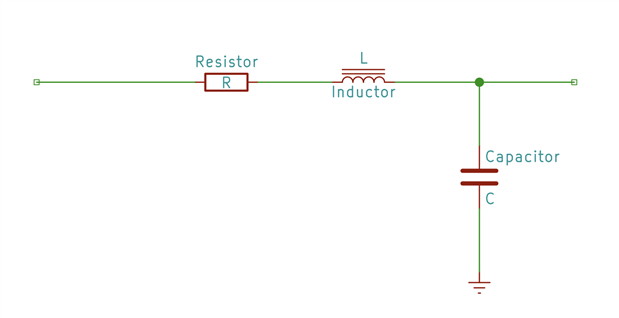
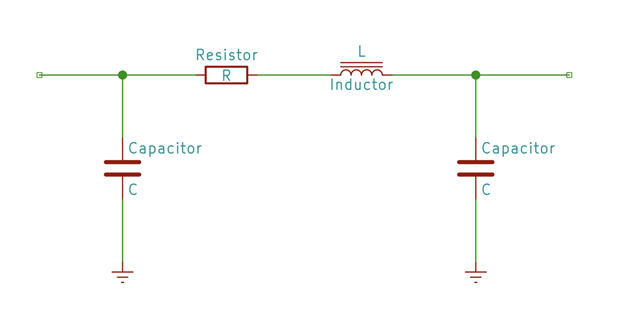
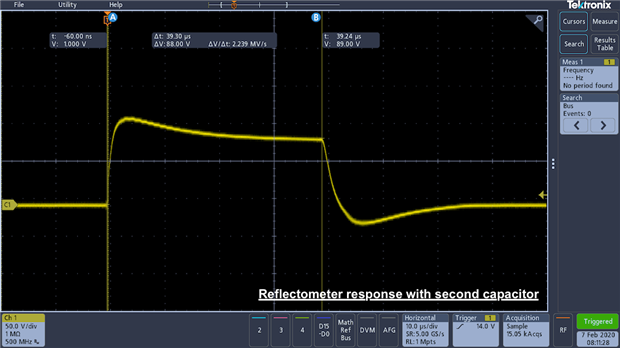
First of all I set up tests on individual inductors with some capacitors I had, left over from other projects. It was immediately found out that the reflectometer would show a discrepancy for a single L-C circuit and so a second capacitor had to be added to the opposite side of the inductor. The resistance in the circuit represents the internal resistance of the inductor.
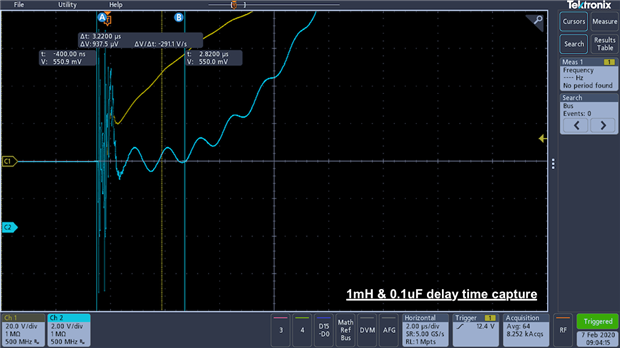
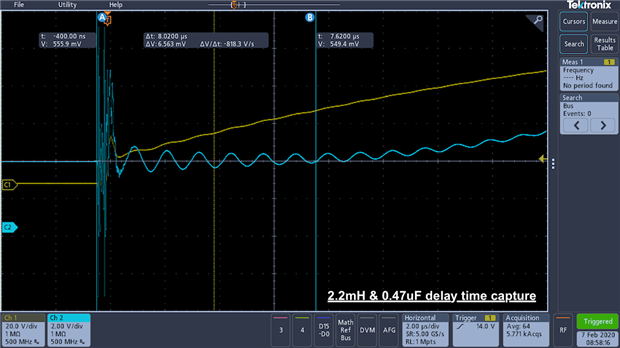
In the next step, I tested three of the inductors against three different capacitance values. Initially a 100 nF capacitor was used, then a 470 nF and then the 100 nF and 470 nF in parallel. The inductors utilised were the 1 mH, 2.2 mH and 6.8 mH radial inductors. The initial concept was to compare the time delay values obtained via the reflectometer against the calculated time delay of the L-C circuit.
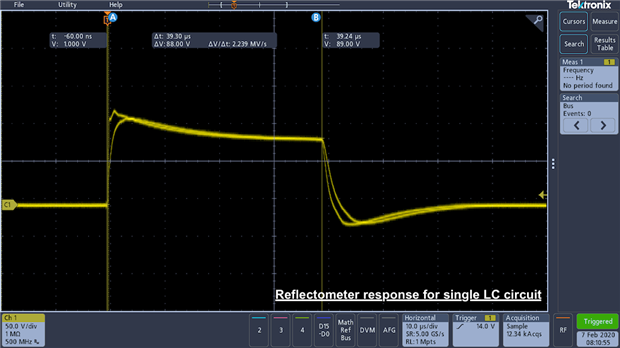
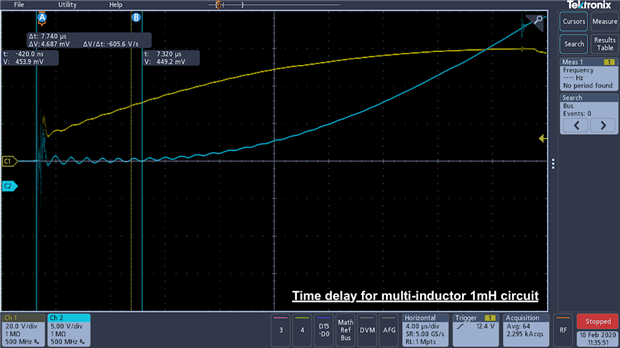
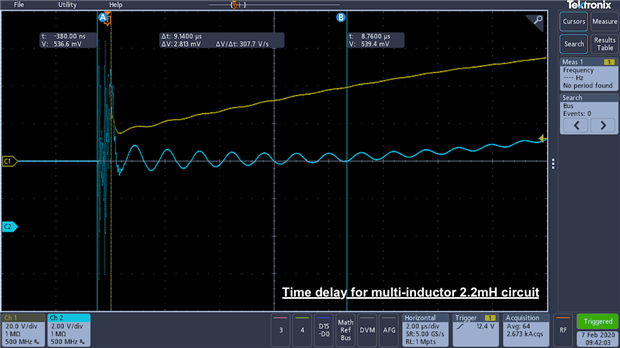
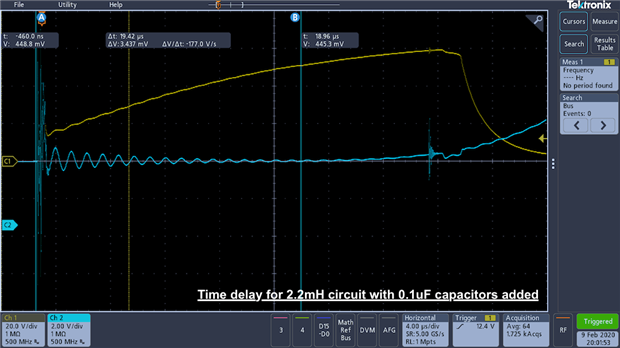
To gain a more accurate reading on the oscilloscope, the data acquisition was turned to averaging mode and the horizontal access zoomed in. The first cursor was set to the initial stabilised voltage and then the second to the point where the oscillating voltage passed through the stabilised voltage value the last time. The two screenshots show an example of a couple of the time delay captures.
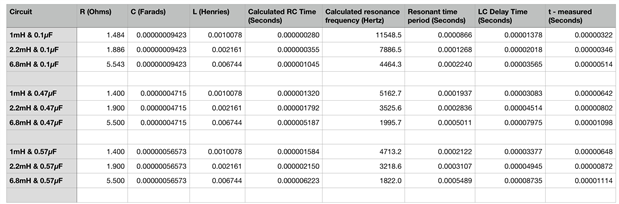
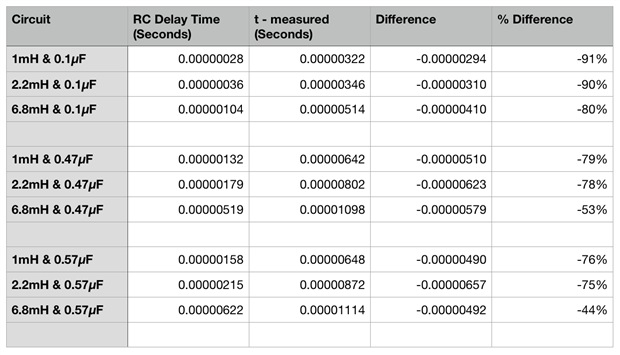
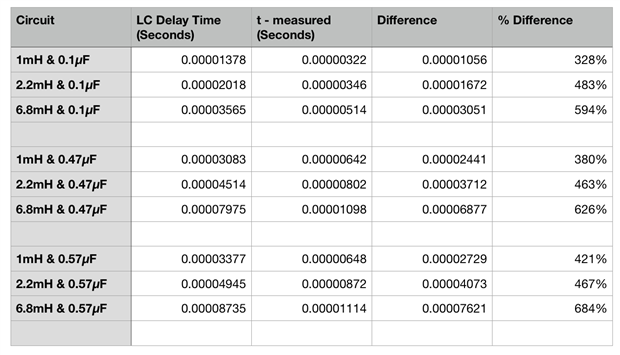
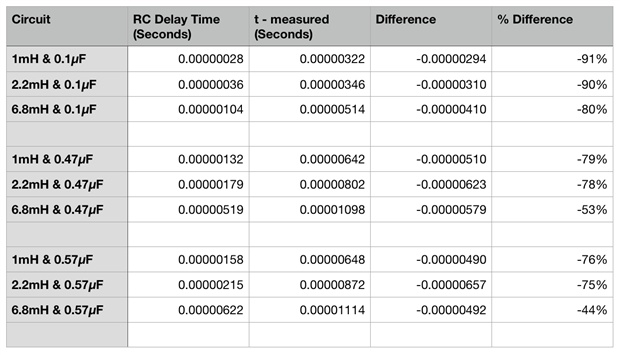
As can be seen from the table below, there was very little correlation between any of the calculation methods and the measured value. The closest calculation was that of the R-C time constant, but the difference varied between -44 % and -90 %. The difference between the L-C time delay and the measured was 380 % best case. The value of capacitance used in the calculations was the sum of both capacitors.
For both the L-C time and resonant time, the differential became worse as the inductance was increased. The R-C time was the opposite to this and saw a lower differential with the higher inductance values.
| {gallery} Difference between calculations and measured time delays |
|---|
R-C Delay calculation difference to measured |
L-C Delay calculation difference to measured |
Resonance Frequency Time delay calculation difference to measured |
Multi-inductor circuit tests
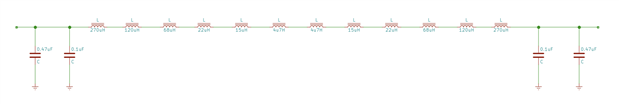
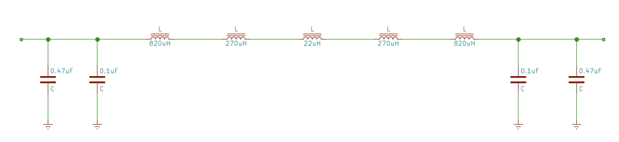
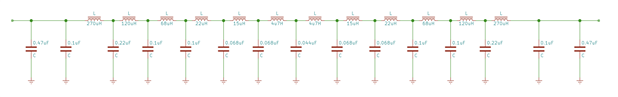
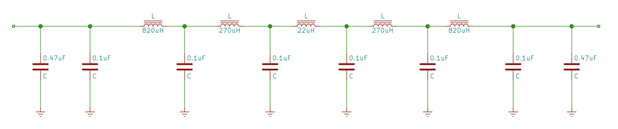
With no correlation observed between any of the calculated tine delay values and the actual measurements, I moved on to simulating faults within the test circuit. To enhance this, I repeated the 1 mH and 2.2 mH tests with the 0.57 uF capacitors by building up the inductance with the smaller inductors included within the kit. The 1 mH was made out of 12 inductors and the 2.2 mH was made out of 5 inductors as detailed in the two schematics.
| {gallery} Multi-Inductor delay line schematics |
|---|
1 mH delay line made from 12 inductors |
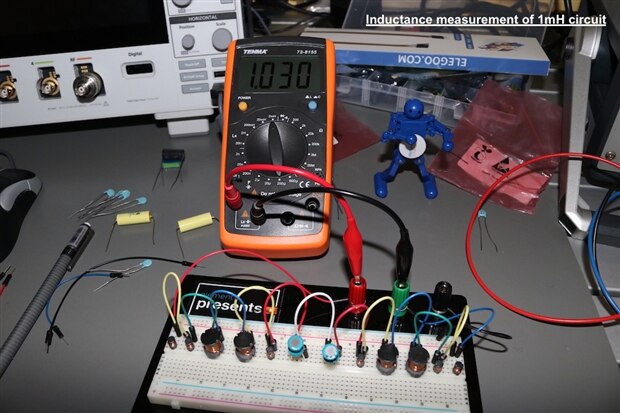
Inductance value of 1 mH circuit |
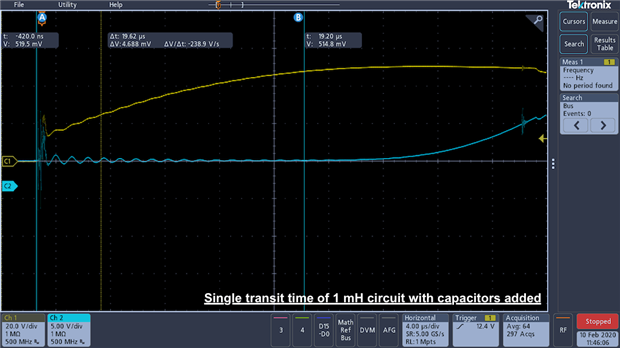
Single transit time measurement for 1 mH circuit |
2.2 mH delay line made from 5 inductors |
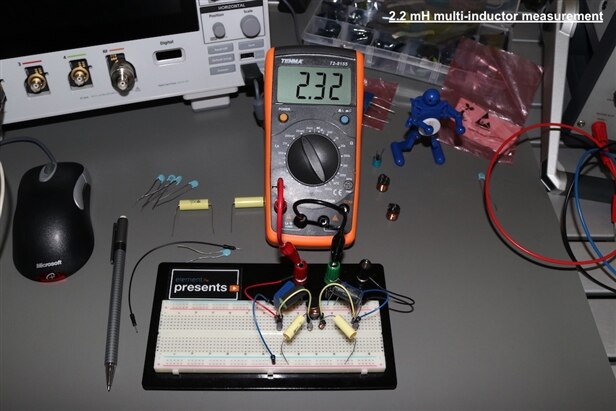
Inductance value of 2.2 mH circuit |
Single transit time measurement for 2.2 mH circuit |
The single transit time captures for each circuit were compared to the times for the single inductors. For a 1 mH single inductor the single transit time was measured as 6.48 us, for the equivalent multi-inductor circuit it was 7.74 us, indicating that inductance is not the only aspect causing the delay. For the 2.2 mH single inductor the single transit time was 8.72 us and 9.14 us for the multi-inductor circuit. This was a bit better correlation, but this circuit consisted of only 5 inductors, so the amount of wire may have been closer to the single inductor circuit. The 1 mH circuit consisted of 12 inductors and was therefore made up physically of more wire than the single 1 mH inductor.
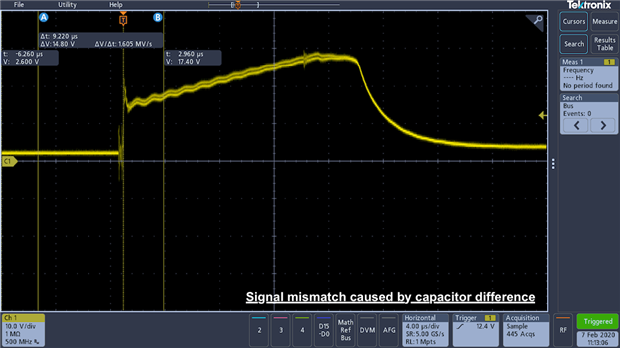
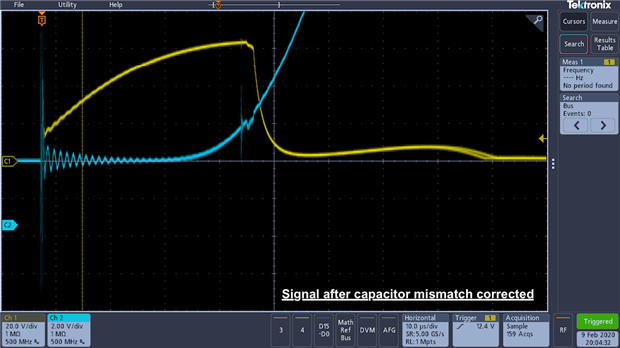
An issue with the test circuits was that the double transit traces were split from one another as seen below. This is not normal and was tracked down to an impedance differential caused by the tolerances within the capacitors and first pair of inductors.
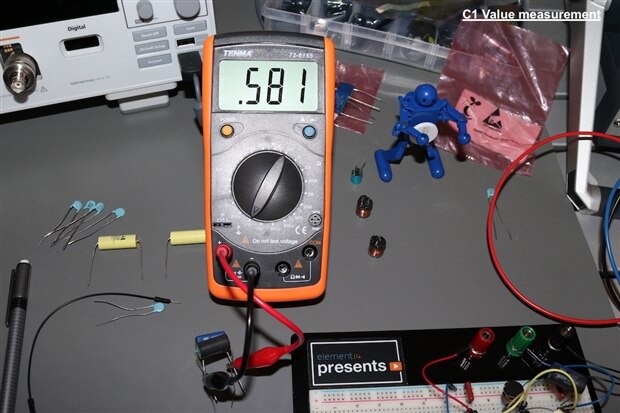
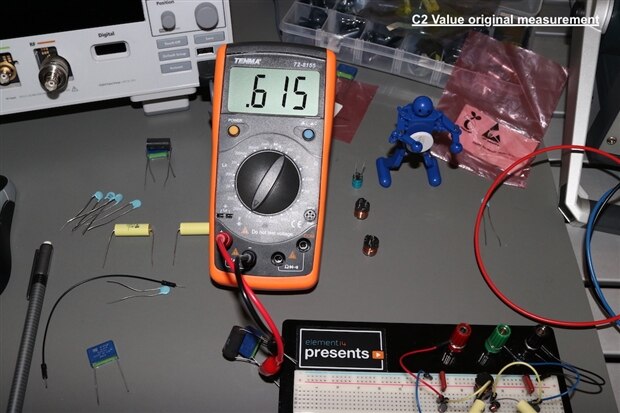
As I was limited by the inductors within the kit, I measured through some different capacitors to find closer matches.
| {gallery} Capacitance correction |
|---|
C1 capacitor value |
C2 capacitor original value |
C2 capacitor corrected value |
This corrected the mismatched signals, to allow some more testing to be carried out.
Initially, faults placed on the circuit, by shorting out the inductors, did not show any distortion in the double transit waveform as expected, but instead just shortened the single transit time. This seems to be caused by the delays of each individual inductor being so short. To improve these delays, I added some capacitors to each of the inductors. For the 1 mH circuit, I used a mixture of 220 nF, 100 nF, 68 nF and 22 nF capacitors. This causes the single transit time to be increased. For the 1 mH circuit the time increased to 19.62 us.
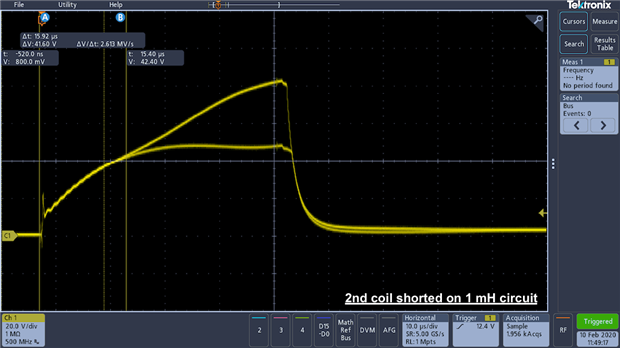
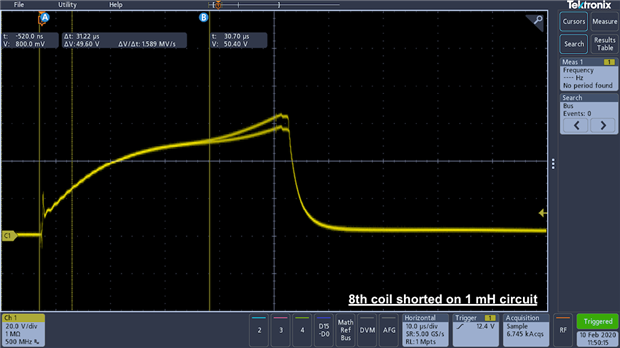
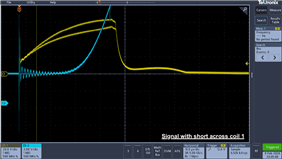
I placed two faults on this circuit, one that shorted out the second inductor and then a second that shorted out the eighth inductor.
| {gallery} 1 mH circuit fault times |
|---|
Double transit trace for short across second coil |
Double transit trace for short across eight coil |
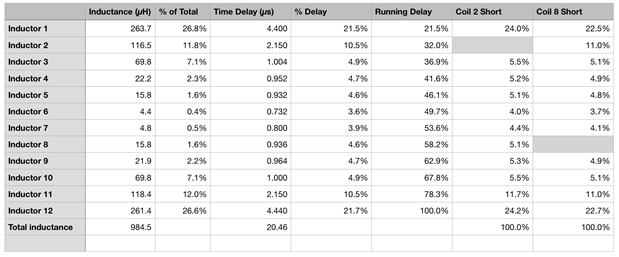
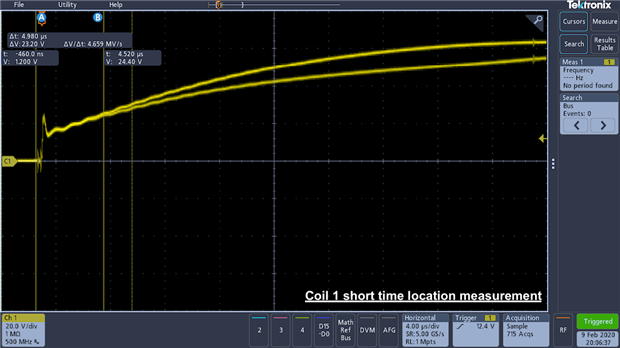
The short across the 2nd coil caused the double transit traces to diverge at 15.92 us. Applying the fault location formula; (15.92 us / (2 x 19.62 us)) x 100% gives a fault location of 41% into the circuit. Applying the same process to the short across the eighth coil gives a fast location of 80% into the circuit, based upon the 30.7 us fault location time recorded. I compared these two times to the delay measurements of the individual coils.
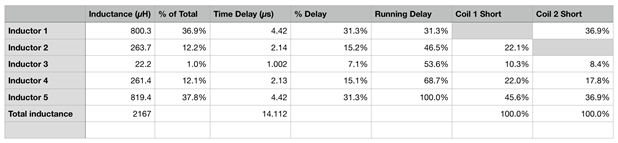
For a short circuit across coil 2, I would be expecting to get a location between 24 and 29.5%. The short across coil 8 should be located between 56 and 61%. On both occasions, the calculation from the reflectometer reading is well below that of the calculation from the delays of the individual coils. The table above shows that the sum of the individual delays is 20.46 us against the reading from the reflectometer of 15.92 us.
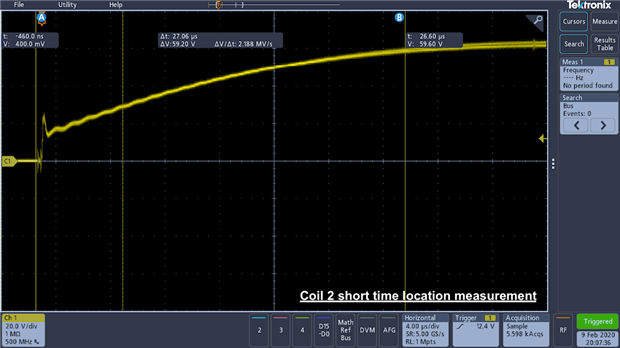
For the 2.2 mH circuit, 100 nF inductors were added to increase the single transit time up to 19.42 us. I also applied two faults to this circuit, one across the first inductor, that gave a fault time of 4.980 us and another across the second inductor that gave a fault time of 27.06 us.
Applying the fault location formula to these two faults provides locations at 13% and 70% into the circuit.
The table above shows the individual measured delays for the 5 coils. With coil 1 shorted, the location should have been around 22% and for coil 2 it should have measured between 36.9% and 45.3%. This follows the results for the 1mH circuit with the locations calculated from the reflectometer coming out lower than the expected results based upon the individual delays measured. However, for this circuit, the sum of the individual delays was lower than that of the delay measured from the rotor reflectometer.
It is likely that the accuracy of the values taken from the oscilloscope traces is questionable, due to the constant variation of the cursor values when measuring the single transit delays and the awkwardness of setting the cursor for measuring the divergence point on the double transit traces. The single transit traces were recorded with the oscilloscope set to averaging, where as the double transit traces were captured in norma sampling mode. In hindsight, using two different sampling methodologies may not have been the best idea, but at the time I felt that it did allow for more accurate cursor placement for the single transit time measurements.
Double Transit Pulse Shapes
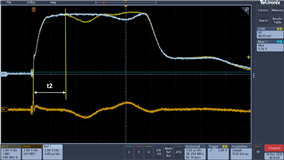
It was noted that for both circuits, the double transit traces did not follow a uniform divergence as would be expected when measuring an actual rotor winding with a fault. Usually both traces with show a displacement, seen below on the left. But during these tests, only one trace showed a divergence, seen below on the right.
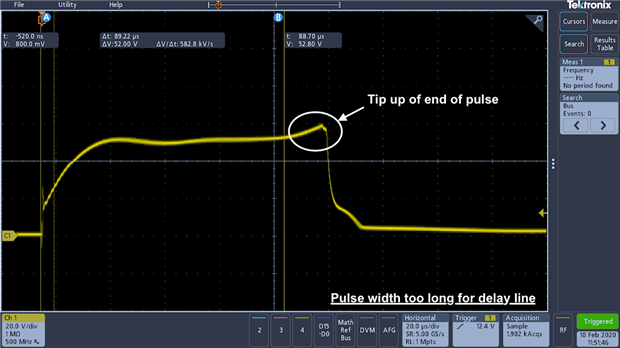
I am not sure what is causing this. This type of behaviour is seen more when there is an impedance mismatch at the start or beginning of the circuit rather than a short along it. I also saw that for the 2.2 mH multi-inductor circuit, I could make the pulse width too long for the test circuit, indicating I had reached the maximum output of the reflectometer. The pulse width is seen to be too long for the circuit when the end of the pulse is tipped upwards, due to the reflected signal sitting on top of the original pulse, as seen for the 1 mH multi-inductor circuit below.
Conclusions
Whilst I have been able to create a delay line of sorts, this just hasn't worked out as I had thought it would. At the moment I cannot find a correlation between the measured delay with the reflectometer and any calculation of the delay. I believe that the delay will be a composite of the L-C network and the length of the wire utilised within the inductor. I could not find the length of the inductor within the technical data sheets to try a different approach to the calculations.